GE Senographe DS, Руководство пользователя

Страница: 688 / 1810

Chapter 7 Page no. 688
JC-ELE-A-028.fm
GE Healthcare Senographe DS
Revision 1 Service Information and Procedures Class A 2385072-16-8EN
Job Card ELE A028 - Collimation Checks
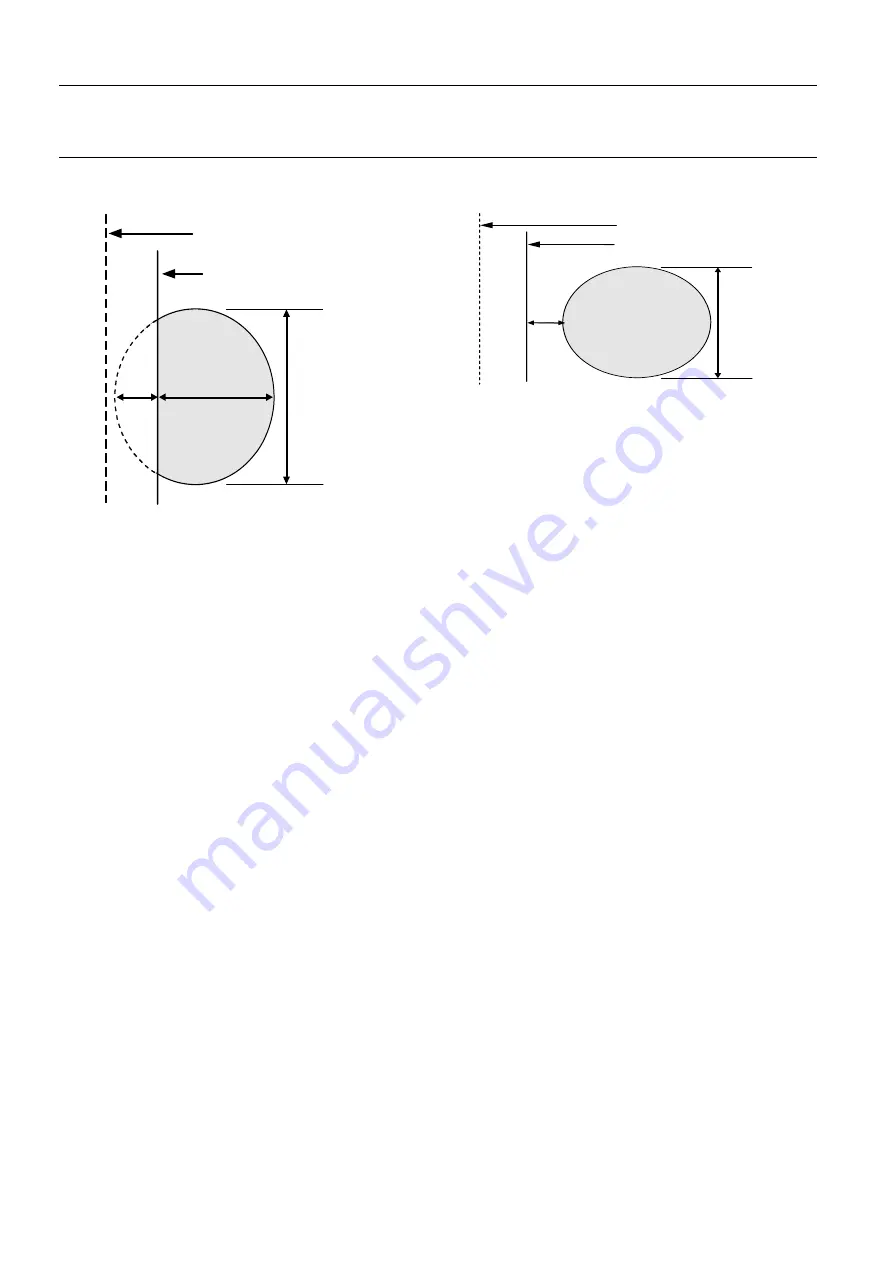
1. There are two possible geometric cases for the coin.
• Case 1: The outer edge of the coin on the compression paddle goes past the detector edge. Part
of the coin is therefore outside of the detector.
• Case 2: The outer edge of the coin on the compression paddle does not go past the detector
edge. All of the coin is therefore in the detector.
2. Examine the image and determine the geometric case.
• If all of the coin is in the detector (case 2), there is a problem with the position of the compression
paddle relative to the chest wall edge of the detector. Either change the compression paddle or
make adjustments to it, then make another exposure and return to step 1 in this section.
• If part of the coin passes past the edge of the detector (case 1), there is no problem with the posi-
tion of the compression paddle relative to the chest wall edge of the detector. Continue with the
measurements in this procedure to determine whether the edge of the compression paddle
passes.
3. From the RAW digital image in the AWS, use the Segment tool to measure the following in the plane
of the segment tool :
• diameter of the coin (W
d
)
• partial diameter of the coin perpendicular to the edge of the image receptor (P
d
)
Insert the measured values of W
d
and P
d
in the table above.
4. From the measured values of W
d
and P
d
, calculate the deviation (Z’
d
) between the alignment of the
edge of the compression paddle and the edge of the image receptor ( in the plane of the segment
tool ), from the following equation:
Z’
d
= (W
d
– P
d
) Eq 6
5. Because the reference plane of the Segment tool is 2 cm above the Breast Support surface, use the
following equation to scale the calculated deviation (Z’
d
) in the plane of the segment tool to the
plane of the image receptor :
Z
d
= Z’
d
•
M Eq. 7
P
d
W
d
Edge of detector
Edge of x-ray field
Z’
d
Case 1
E d g e o f
d e te c to r
E d g e o f x -ra y
fie ld
Z ’
d
W
d
Case 2