Concepts and Features
R&S
®
ZNB/ZNBT
94
User Manual 1173.9163.02 ─ 38
Marker Frmt" ), or formatted individually (TRACE > MARKER > "Marker Props" >
"Marker Format").
The available marker formats are defined for all measured quantities and trace formats
(see
Chapter 4.2.3.3, "Measured Quantities and Trace Formats"
Essentially, a marker format is simply a conversion between points on a complex-val-
ued trace (the raw measurement data) and the respective target format. This must be
kept in mind when interpreting the results and physical units displayed.
The following table describes how a complex marker value z = x + jy is converted. It
makes use of the polar representation z = x + jy = |z| e
j
φ
(z)
, where
|z| = ( x
2
+ y
2
)
1/2
and
φ
(z) = arctan( y / x )
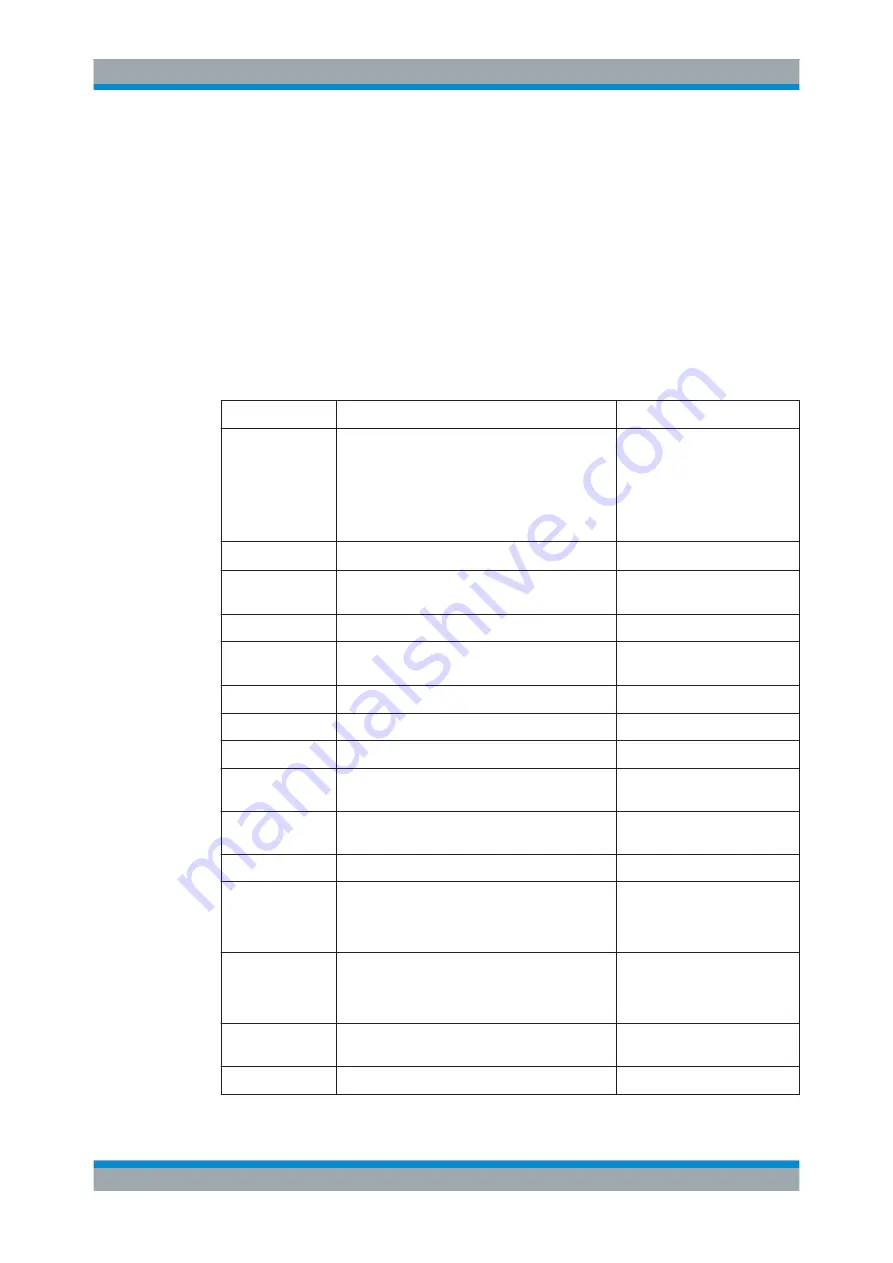
Table 4-2: Marker formats
Marker Format
Description
Formula
Default
●
For an
individual marker
this means the
marker is formatted according to the default
marker format of the related trace.
●
For a
trace's default marker format
this
means the default format is (dynamically)
adjusted according to the selected trace for-
mat.
–
Lin Mag
Magnitude of z, unconverted
|z| = sqrt ( x
2
+ y
2
)
dB Mag
Magnitude of z in dB
|z| = sqrt ( x
2
+ y
2
) dB Mag(z) =
20 * log|z| dB
Phase
Phase of z
φ (z) = arctan (y/x)
Delay
Group delay, neg. derivative of the phase
response
*)
– dφ(z) / dω, where ω denotes
the stimulus frequency
Real
Real part of z
Re(z) = x
Imag
Imaginary part of z
Im(z) = y
SWR
(Voltage) Standing Wave Ratio
SWR = (1 + |z|) / (1 – |z|)
dB Mag Phase
Magnitude of z in dB and phase in two lines
20 * log|z| dB arctan ( Im(z) /
Re(z) )
Lin Mag Phase
Magnitude of z (unconverted) and phase in two
lines
|z| arctan ( Im(z) / Re(z) )
Real Imag
Real and imaginary part of z in two lines
x y
R + j X
Unnormalized resistance, reactance, and L or C in
three lines (Smith diagram)
R
X
L or C
**)
G + j B
Unnormalized conductance, susceptance, and L
or C in three lines (Inverted Smith diagram)
G
B
L or C
**)
IMP Mag
Impedance Magnitude (combined complex impe-
dance)
**)
|Z| = (R
2
+ X
2
)
1/2
Index
Index of the current sweep point
–
Screen Elements






























