Rohde & Schwarz 1311.6010.22, User Manual

Page: 120 / 1451

Concepts and Features
R&S
®
ZNB/ZNBT
120 User Manual 1173.9163.02 ─ 53
  
P S E S P S
1
0 0
1
1

   
 
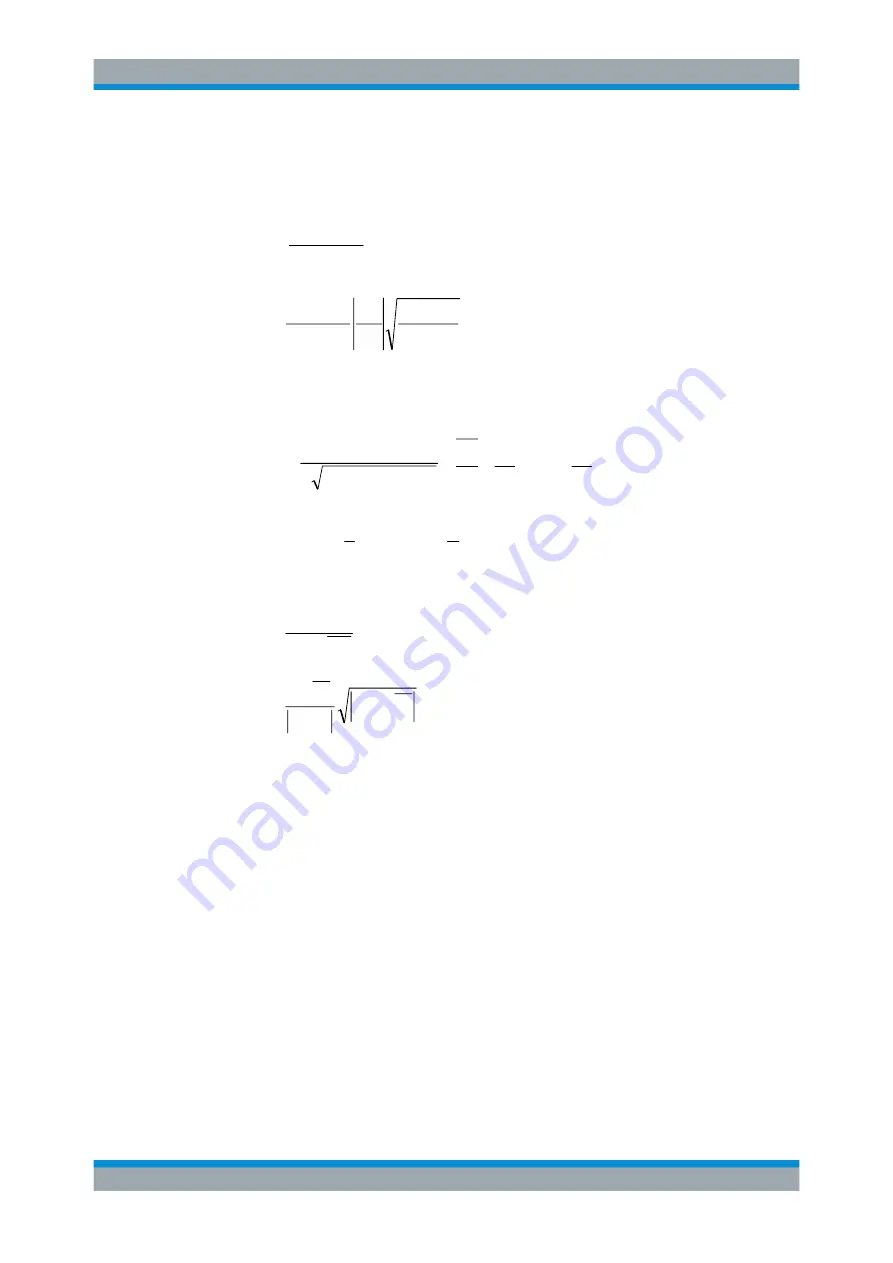
with the unit matrix E and two additional matrices with the elements
i i
i i
ii
Z Z
Z Z
0 1
0 1




) Re(
) Re(
1
0
0
1
1 0
0
i
i
i
i
i i
i
ii
Z
Z
Z
Z
Z Z
Z
P


2. Power waves
In the model of Kurokawa ("Power Waves and the Scattering Matrix"), the wave
quantities a and b are transformed as follows:













 
 
 






i
i
i i i i
i i i i
i i
i
i
b
a
Z Z Z Z
Z Z Z Z
Z Z
b
a
0
0
1 0 1 0
1 0 1 0
1 0
1
1
) Re( ) Re( 2
1
The renormalized S-matrix S1 is calculated as:
 
 
A S E S A S
1
0 0
1
1

     
with the unit matrix E and two additional matrices with the elements
i i
i i
ii
Z Z
Z Z
0 1
0 1


 
ii ii
ii
ii
ii
A
  
 
 

1
1
1
5.3.3 Impedance Parameters
An impedance is the complex ratio between a voltage and a current. The analyzer pro-
vides two independent sets of impedance parameters:
●
Converted impedances (each impedance parameter is obtained from a single S-
parameter)
●
Z-parameters (complete description of an n-port DUT)
5.3.3.1 Converted Impedances
The converted impedance parameters Z
ij
(1 ≤i,j ≤n) describe the input impedances of
an n-port DUT with fully matched outputs. The analyzer converts a single measured S-
parameter S
ij
to determine the corresponding converted impedance, under the
assumption that each of the other ports is terminated with its respective reference
impedance Z
0k
(matched-circuit parameters). As a result, converted impedances can-
not completely describe general n-port DUTs.
Measurement Results