Concepts and Features
R&S
®
ZNC
37
User Manual 1173.9557.02 ─ 13
Trace Format
Description
Formula
Lin Mag
Magnitude of z, unconverted
|z| = sqrt ( x
2
+ y
2
)
dB Mag
Magnitude of z in dB
dB Mag(z) = 20 * log|z| dB
Phase
Phase of z
φ (z) = arctan (y/x)
Real
Real part of z
Re(z) = x
Imag
Imaginary part of z
Im(z) = y
SWR
(Voltage) Standing Wave Ratio
SWR = (1 + |z|) / (1 – |z|)
Delay
Group delay, neg. derivative of the
phase response
– d φ (z) / dΩ (Ω = 2
π
* f)
An extended range of formats and conversion formulas is available for markers. To con-
vert any point on a trace, create a marker and select the appropriate marker format.
Marker and trace formats can be selected independently.
3.2.4.3
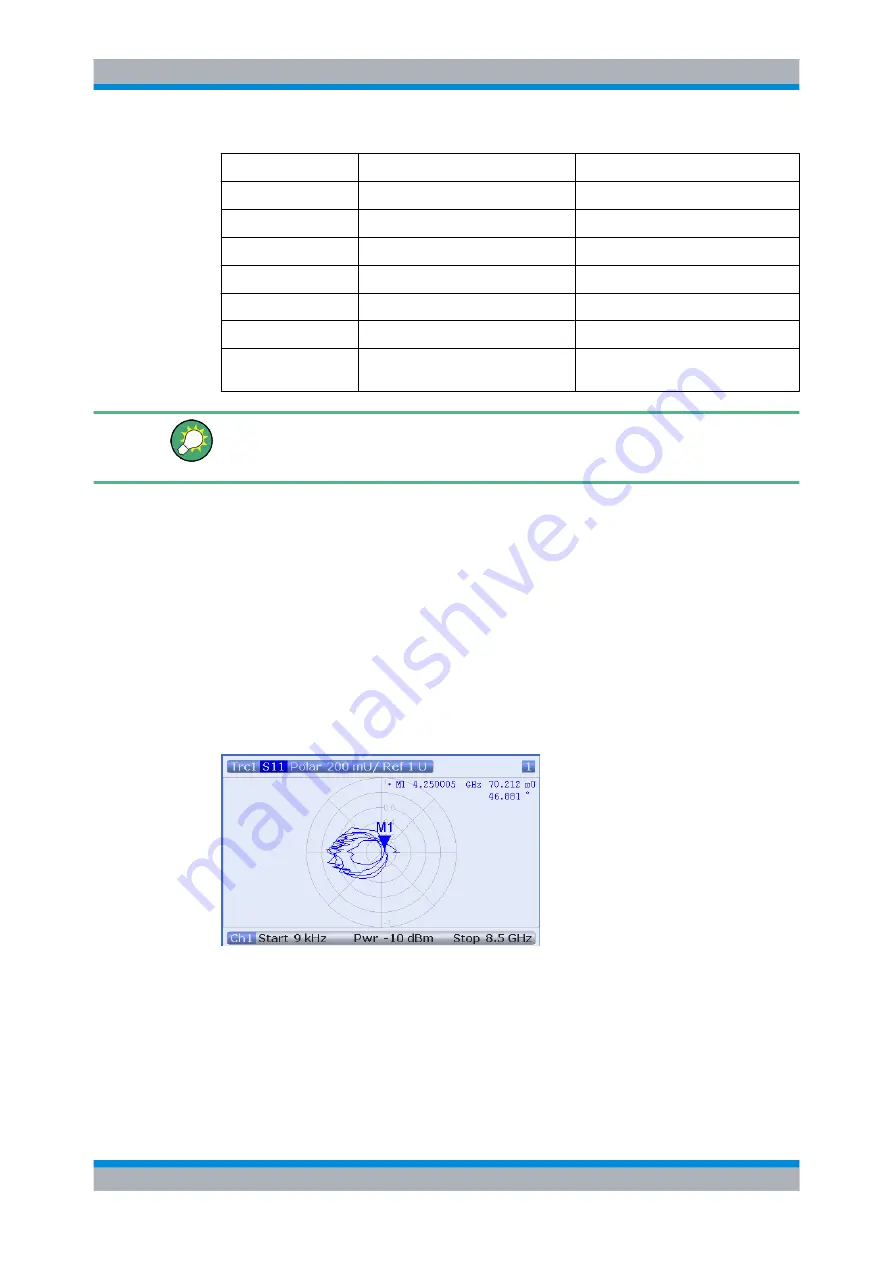
Polar Diagrams
Polar diagrams show the measured data (response values) in the complex plane with a
horizontal real axis and a vertical imaginary axis. The grid lines correspond to points of
equal magnitude and phase.
●
The magnitude of the response values corresponds to their distance from the center.
Values with the same magnitude are located on circles.
●
The phase of the response values is given by the angle from the positive horizontal
axis. Values with the same phase are on straight lines originating at the center.
The following example shows a polar diagram with a marker used to display a pair of
stimulus and response values.
Example: Reflection coefficients in polar diagrams
If the measured quantity is a complex reflection coefficient (S
11
, S
22
etc.), then the center
of the polar diagram corresponds to a perfect load Z
0
at the input test port of the DUT (no
reflection, matched input), whereas the outer circumference (|S
ii
| = 1) represents a totally
reflected signal.
Screen Elements
















































