19
Pixel size
A quantity of decisive importance in this connec-
tion is the maximum scanning angle set via the
scanning zoom. By varying the scanning angle, it is
possible to directly influence the edge length of
the scanned field in the intermediate image plane
(or object plane), and thus the pixel size (at a given
number of pixels per line). The smaller the scan-
ning angle, the smaller is the edge length of the
scanned field, and the smaller is the pixel (see the
example below).
In this way, the user of a Carl Zeiss confocal LSM
can control the sampling rate (pixel size). For set-
ting the suitable scanning zoom for correct
Nyquist sampling, the pixel size d
Pix
in the object
plane is important.
For example, with a 40 x objective (NA = 1.3),
512 pixels per scan line and a wavelength of 488 nm,
the full resolving power (correct sampling) is
achieved with a scanning zoom of 4.56 as a mini-
mum; the corresponding pixel size is 95.8 nm.
With lower factors of the scanning zoom the pixel
size itself will be the limiting factor for resolution
(pixel resolution). Higher factors will cause over-
sampling. Hence, the zoom factor influences not
only the total magnification but also the resolution
properties of the system.
With the more recent LSM systems of Carl Zeiss,
the number of sampling points can also be influ-
enced by an increase in the number of pixels per
scan line.
(The number of pixels (X/Y) per image can be
freely selected between 4 x 2 and 2048 x 2048).
For a Carl Zeiss confocal LSM, there is a simple for-
mula, based on the edge length of the scanned
field in the intermediate image:
Number of pixels = number of pixels per line
Zoom factor (Z) = scanning zoom set in the software
(Example: Zoom factor 2 reduces the edge length of the
scanned field by a factor of 2)
Magnification
obj
= objective magnification
System constant = 8.94 mm with LSM 510, LSM 5 Pascal
(minimum zoom factor = 0.7); 12.77 mm with LSM 310,
LSM 410 (minimum zoom factor =1)
The minimum scanning zoom needed to fullfill the
Nyquist theorem can therefore be calculated as
follows:
NA = numerical aperture of objective
λ
exc
= excitation wavelength
Signal Processing
Part 2
b)
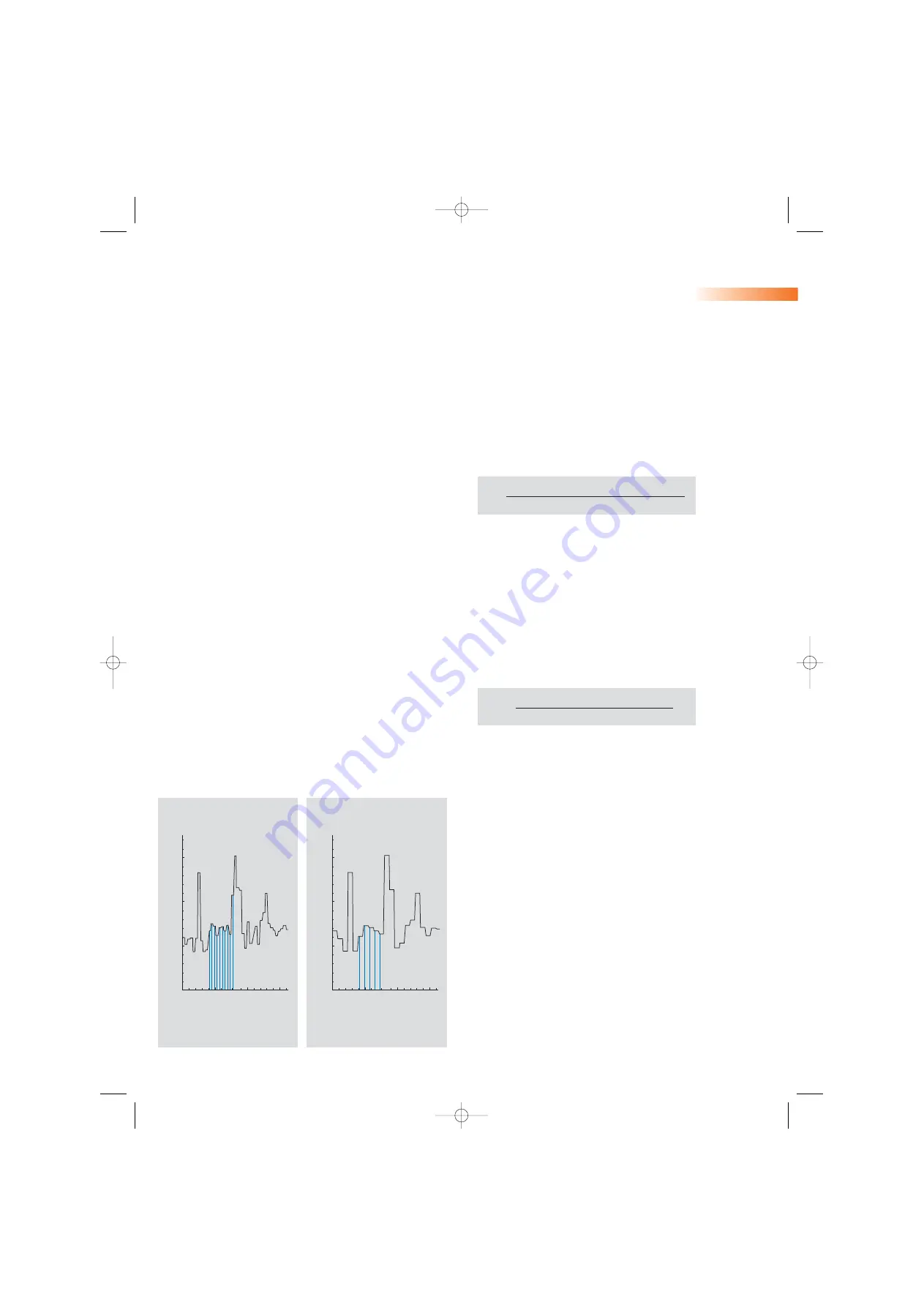
c)
Correct sampling
Undersampling
Pixels
Pixels
50
100
150
50
100
150
0
50
100
150
0
50
100
150
system constant
number of pixels
.
zoomfactor
.
magnification
obj
d
pix
=
3.92
.
NA
.
system constant
number of pixels
.
magnification
obj
.
exc
Z
≥
337_Zeiss_Grundlagen_e 25.09.2003 16:16 Uhr Seite 22






























