HP 49g+, Руководство пользователя, Страница: 560 / 862

Page 17-11
The Student-t distribution
The Student-t, or simply, the t-, distribution has one parameter
ν
, known as the
degrees of freedom of the distribution. The probability distribution function
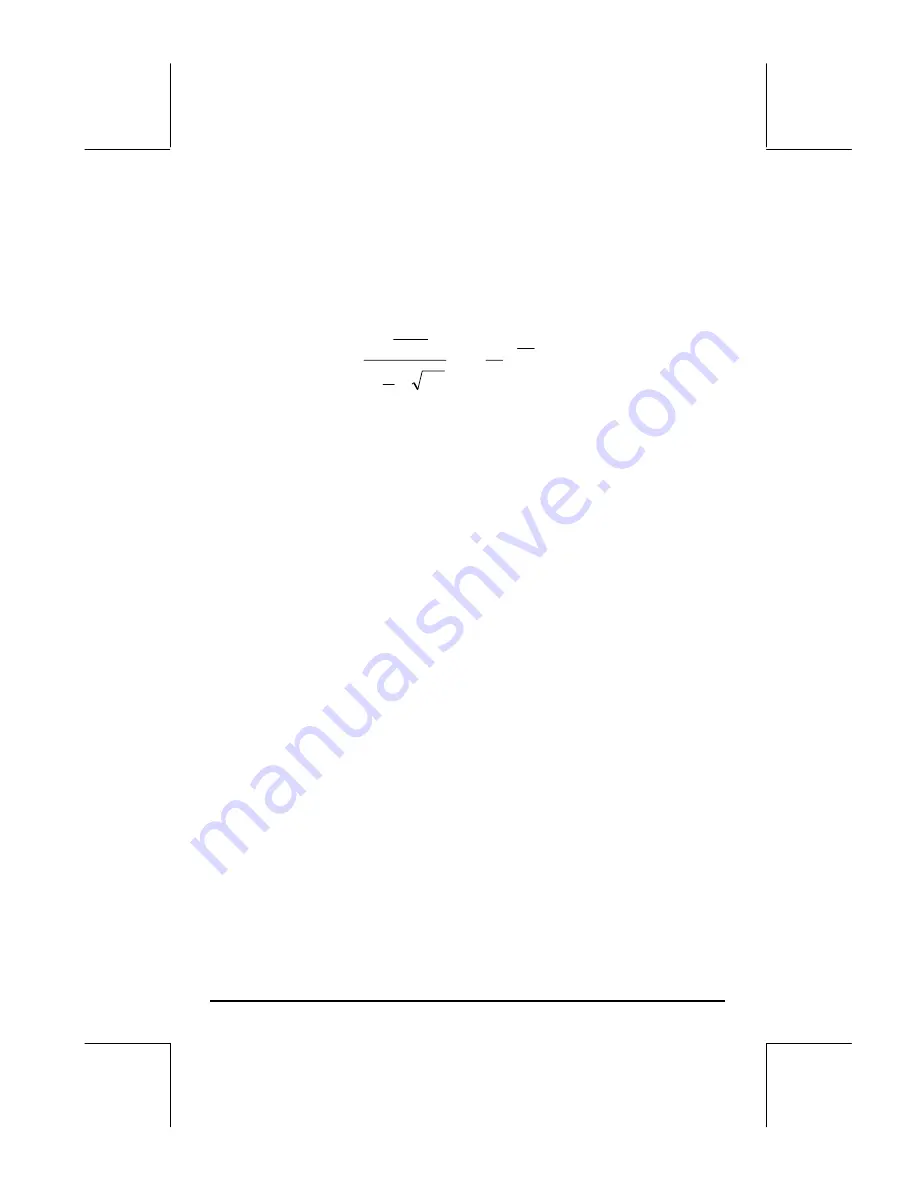
(pdf) is given by
∞ < < −∞ + ⋅
⋅ Γ
+
Γ
=
+
−
t
t
t f , ) 1 (
)
2
(
)
2
1
(
) (
2
1
2
ν
ν
πν
ν
ν
where
Γ
(
α
) = (
α
-1)! is the GAMMA function defined in Chapter 3.
The calculator provides for values of the upper-tail (cumulative) distribution
function for the t-distribution, function UTPT, given the parameter
ν
and the
value of t, i.e., UTPT(
ν
,t). The definition of this function is, therefore,
∫ ∫
∞ −
∞
≤ − = − = =
t
t
t T P dt t f dt t f t UTPT
) ( 1 ) ( 1 ) ( ) , ( ν
For example, UTPT(5,2.5) = 2.7245…E-2. Other probability calculations for
the t-distribution can be defined using the function UTPT, as follows:
•
P(T<a) = 1 - UTPT(
ν
,a)
•
P(a<T<b) = P(T<b) - P(T<a) = 1 - UTPT(
ν
,b) - (1 - UTPT(
ν
,a)) =
UTPT(
ν
,a) - UTPT(
ν
,b)
•
P(T>c) = UTPT(
ν
,c)
Examples: Given
ν
= 12, determine:
P(T<0.5) = 1-UTPT(12,0.5) = 0.68694..
P(-0.5<T<0.5) = UTPT(12,-0.5)-UTPT(12,0.5) = 0.3738…
P(T> -1.2) = UTPT(12,-1.2) = 0.8733…
The Chi-square distribution
The Chi-square (
χ
2
) distribution has one parameter
ν
, known as the degrees of
freedom. The probability distribution function (pdf) is given by