FSB Design Guidelines
R
Intel
®
855PM Chipset Platform Design Guide
35
C
SELF
= C22 (Trace 2, i.e. CS2a + CS2b)
If a stack-up that is employed does not adhere to the recommended stack-up, then a new extraction must
be made for the stack-up using a 2D field solver program. According to the 2D field solver results, new
coupling calculations must be performed to ensure that the coupling results are less than the
aforementioned capacitive coupling maximum value of 8.15%. If the coupling results are greater than
the maximum value, then additional system level simulations must be performed to avoid any signal
quality issues due to crosstalk effects.
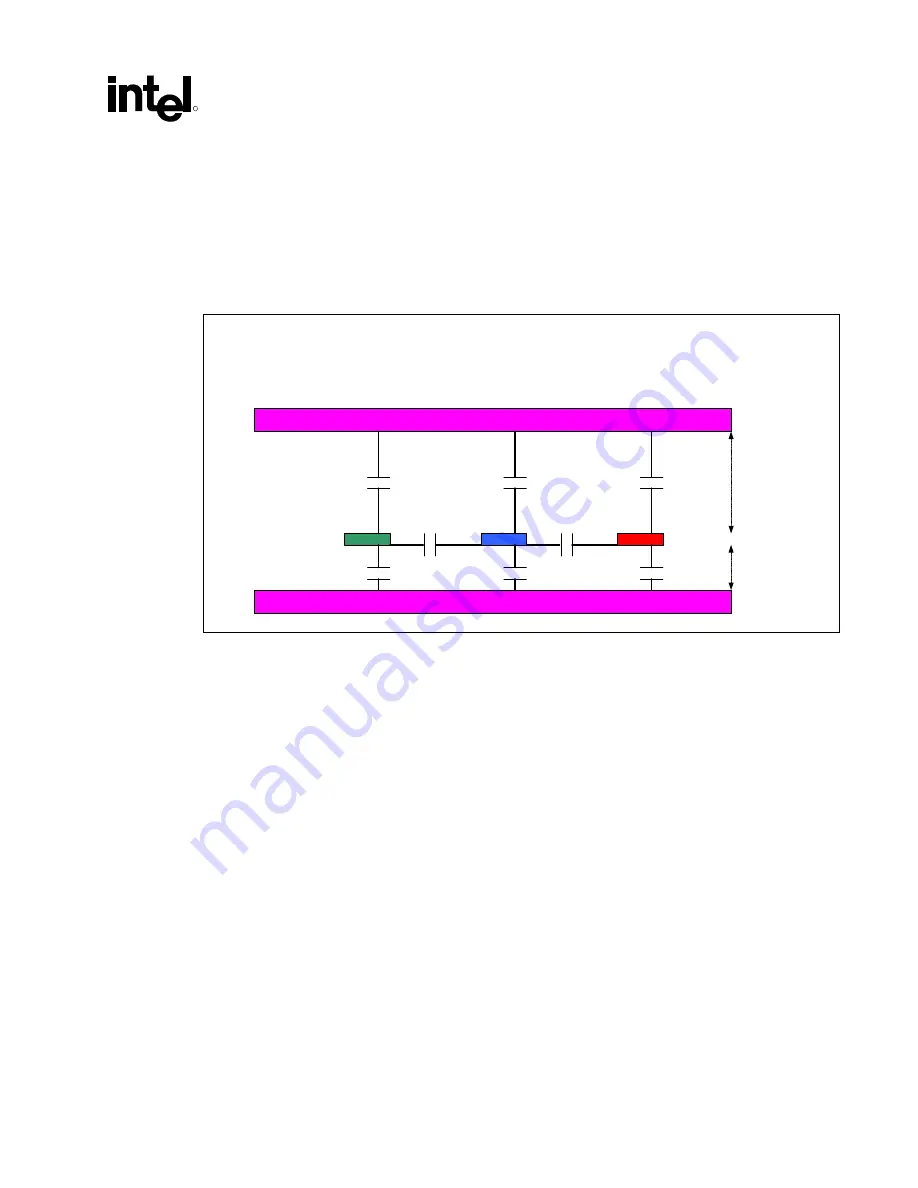
Figure 5. Recommended Stack-up Capacitive Coupling Model
Trace 1
Trace 2
Trace 3
GND
GND
C21
C23
4.8 Mil
11.2 Mil
CS2b
CS2a
CS1a
CS3a
CS1b
CS3b
Note : CS1a + CS1b = C11
CS2a + CS2b = C22
CS3a +CS3b = C33
4.1.1.4.
Signal Propagation Time to Distance Relationship and Assumptions
Due to the high frequency nature of some interfaces and signals, length matching may or may not exist
as part of the routing requirements for a given interface. In general, the tolerances that specific signals in
a bus must be routed to will be stated as a length measured in mils or inches and is specific to the
recommended motherboard stack-up (see Figure 2). However, some length matching tolerances for
signals listed in this design guide may be stated as a measurement of time. In such cases, the correlation
of the period of time to an actual length value will depend on board stack-up.
Based on the recommended stack-up, the signal propagation time to distance relationship, for the
purpose of this design guide, is as follows:
Strip-line (internal layer) Routing: 180 ps for 1.0 inch
Micro-strip (external layer) Routing: 162 ps for 1.0 inch
For example, a length-matching requirement of ± 50 ps for routing on a strip-line (internal) layer would
correlate to a trace length whose tolerance is within ± 278 mils of an associated trace. The signal
propagation time to distance relationship listed above is based on a single transmission line model
incorporating a
typical
stack-up. Thus, no other signals or traces are accounted for in such a model and
there is an assumption of zero coupling with other traces. Also, the recommended stack-up’s parameter
tolerances are not taken into account in the “typical” stack-up assumptions. Finally, in cases that need to
account for worst-case stack-up parameters and for even or odd mode coupling, new extractions from
the stack-up model must be done to provide an accurate signal propagation time to distance relationship.















































