HV100 Series High Performance Current Vector Inverter
98
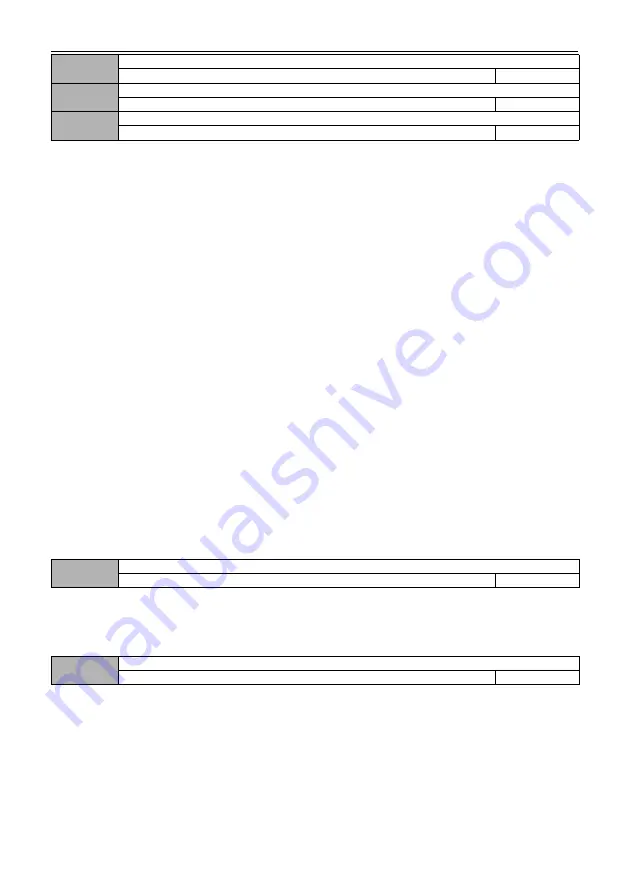
08.05
Proportional gain KP1
0.01
~
100.00s
2.50
08.06
Integral time Ti1
0.01
~
10.00s
0.10
08.07
D
ifferential time Td1
0.01
~
10.00s
0.00
0.00: No derivative adjustment
Proportional gain (Kp):
The adjustment intensity of the whole PID regulator is determined by this. And the greater the P is, the greater the
adjustment intensity is. But if in too large state, there is easy to produce oscillation.
When the feedback deviates from the set value, the deviation and output become the regulating value of proportion. If
the deviation is constant, the regulating value is also constant. Proportional adjustment can quickly show out the feedback
changes, but it is impossible to achieve error-free control by proportional control alone. The larger the proportional gain is,
the faster the adjustment speed of the system will be, but if too large, oscillation will occur. The adjustment method is to set
the integration time for a longer time, and the differentiation time for zero, then use proportional control to make the system
run. With changing the size of the given quantity, it can observe the stable deviation (static difference) between the
feedback signal and the set value. If the static difference changes in the direction of the set value (for example, if the set
value is increased and the feedback value is always less than the set value after the system is stable), then increasing the
proportional gain continues. Otherwise reduce the proportional gain, and repeat the above process until the static
difference is relatively small (It's hard to make it without any static errors)
Integral time (ti):
Determine the speed of PID regulators to do the integral adjustment of deviation.
When the feedback deviates from the set value, the output adjustment value need to continuously accumulate. And if
the deviation persists, the adjustment value continuously increases until there is no deviation. Integral regulator can validly
eliminate static difference. If the integral regulator is too strong, there will be repeated overshoot, which will make the
system oscillate. Generally, the adjustment of integration time parameters is from large to small, and the integration time is
gradually adjusted, while the effect of system adjustment is observed,all until the stable speed of the system meets the
requirements.
Differential time (Td):
Determine the strength of PID regulator to adjust the rate of deviation change.
When the feedback changes with the set deviation, the rate of deviation change and output become the regulating value of
proportion, which is only related to the direction and magnitude of deviation change, but has nothing to do with the direction
and magnitude of deviation itself. when the feedback signal changes, the function of derivative adjustment is to adjust
according to the changing trend of it, so as to restrain the change of it. Please use derivative regulator with caution,
because derivative regulator is easy to amplify the interference of the system, especially the interference from large
changing frequency.
08.08
Sampling period T
0.01
~
100.00s
0.10
0.00
:
Automatic
Sampling period is the period to sample the feedback value, and the regulator operates once in each sampling period.
The larger the sampling period, the slower the response, but the better the suppression effect on interference signal, so it is
generally unnecessary to set it.
08.09
Deviation limit
0.0
~
100.0%
0.0%
The deviation limit is the ratio of the set value and the absolute value,which means the absolute deviation value
between system feedback value and the set value. When the feedback value is within the deviation limit, PID control does
not work. As shown in the figure below, setting a reasonable deviation limit can prevent the system from frequently
adjusting near the target value, which is helpful to improve the stability of the system.















































