10-14
L90 LINE CURRENT DIFFERENTIAL SYSTEM – INSTRUCTION MANUAL
OVERVIEW
CHAPTER 10: THEORY OF OPERATION
10
•
For all the terminal currents that are above the 3 pu level, the relative angle difference is calculated. If all three
terminals see significant current, then all three pairs (1, 2), (2, 3), and (1, 3) are considered and the maximum angle
difference is used in further calculations.
•
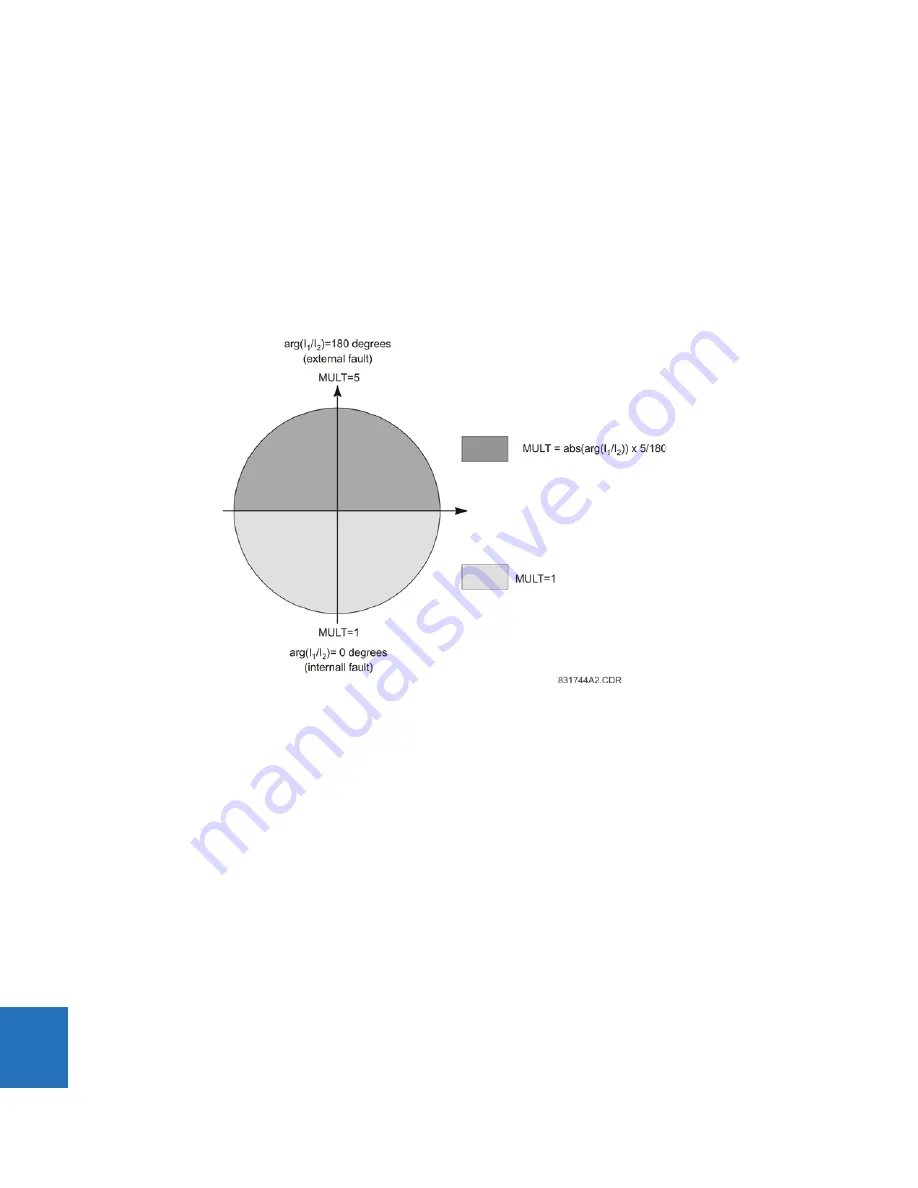
Depending on the angle difference between the terminal currents, the value of sigma used for the adaptive restraint
current is increased by the multiple factor of 1, 5, or 2.5 to 5 as shown in the figure. As shown, a factor of 1 is used for
internal faults, and a factor of 2.5 to 5 is used for external faults. This allows the relay to be sensitive simultaneously for
internal faults and robust for external faults with a possible CT saturation.
If more than one CT is connected to the relay (breaker-and-the half applications), the CT saturation mechanism is executed
between the maximum local current against the sum of all others, then between the maximum local and remote currents
to select the secure multiplier MULT. A maximum of two (local and remote) is selected and then applied to adaptive
restraint.
Figure 10-4: CT saturation adaptive restraint multiplier
10.1.18 Charging current compensation
The basic premise for the operation of differential protection schemes in general, and of the L90 line differential element in
particular, is that the sum of the currents entering the protected zone is zero. In the case of a power system transmission
line, this is not entirely true because of the capacitive charging current of the line. For short transmission lines the charging
current is a small factor and can therefore be treated as an unknown error. In this application, the L90 can be deployed
without voltage sensors and the line charging current is included as a constant term in the total variance, increasing the
differential restraint current. For long transmission lines, the charging current is a significant factor and needs to be
computed to provide increased sensitivity to fault current.
Compensation for charging current requires the voltage at the terminals be supplied to the relays. The algorithm
calculates I
q
= C x dv/dt for each phase, which is then subtracted from the measured currents at both ends of the line. This
is a simple approach that provides adequate compensation of the capacitive current at the fundamental power system
frequency. Travelling waves on the transmission line are not compensated for, and contribute to restraint by increasing the
measurement of errors in the data set.
The figures show underlying single phase models for compensation for two and three-terminal systems.
















































