User Manual
Chapter 7
GFK-1742F
Jan 2020
Programmed Motion
216
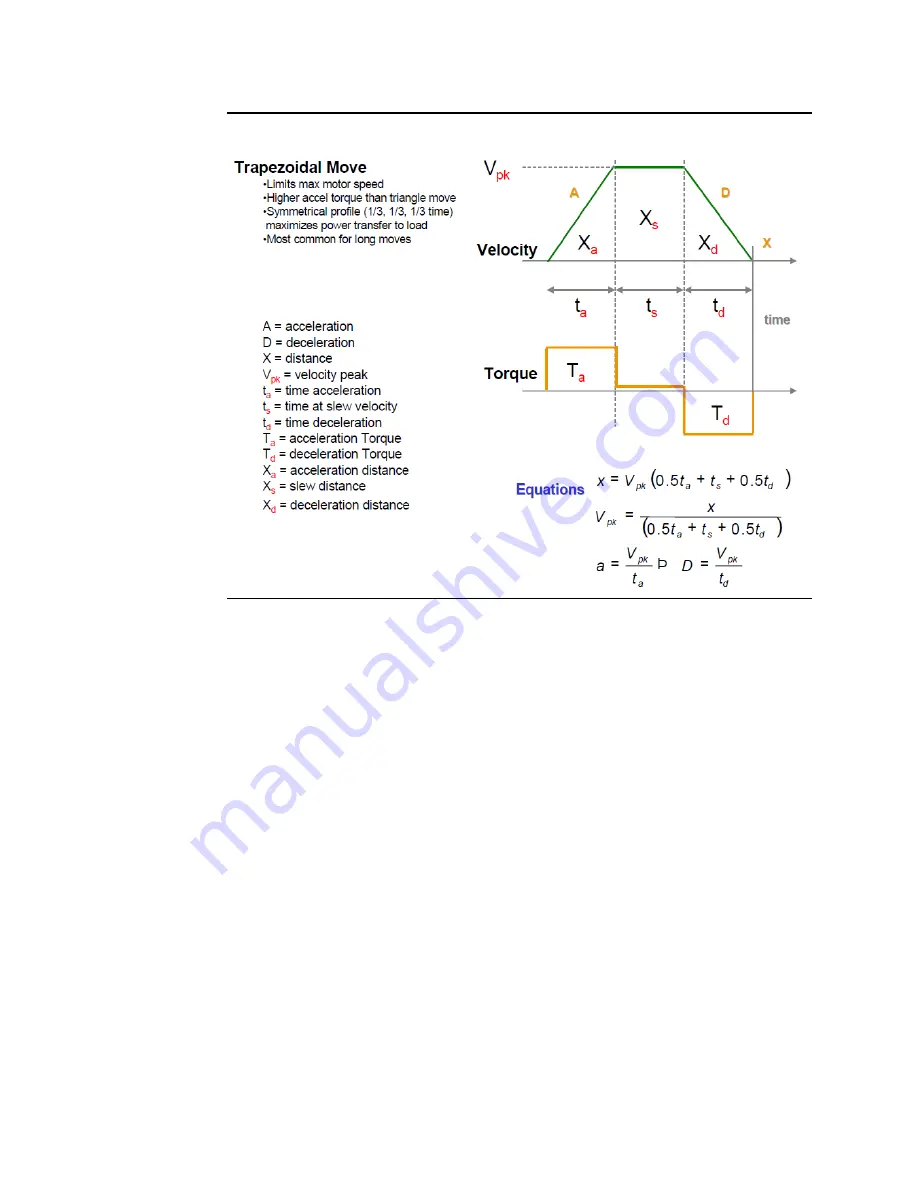
Figure 91: Trapezoidal Move
Once the move segment outline is drawn, you will need to examine specifications or
physical restrictions applicable to the move. For instance, the move may have to complete
in a certain time interval (t
a
+ t
s
+ t
d
) or move a fixed distance (X). The maximum velocity
(V
pk
) of the servomotor is one example of a physical limitation. Given any two known values
of the acceleration portion of the move segment, a remaining variable can be found using
the kinematic equations as illustrated in the example below.
Trapezoidal Velocity Profile Application Example
For this example, assume that a complete move of 16 inches must be made in three seconds
and the maximum motor velocity, translated through the gearing is 15 inches per second.
Using the rule of thumb for trapez
oidal moves, divide the move’s time into thirds:
t
a
= 1 sec,
t
s
= 1 sec and t
d
= 1 sec. You can also subdivide the 16-inch move into three distances. The
slew (X
s
) section of an equally divided trapezoidal velocity profile represents ½ of the
distance moved and the acceleration (X
a
) and deceleration (X
d
) portions each represent ¼
of the total distance: X
a
=4 in, Xs =8 in and X
d
=4 in.
To calculate peak Velocity (V
pk
), the first acceleration portion of the move must travel a
given distance (X
a
) in a given time (t
a
). From the above Kinematic Velocity formula (2X/t)
using the given, X
a
=4 inches and t
a
= 1 second, (2*4 inches) / 1 second = 8 inches/second.
To calculate Acceleration the simplest formula is (V/T)=(8 inches/second / 1 second)=8
inches/second/second.
The Position (Distance = X) is the entire distance moved (X
a
+ X
s
+ X
d
) or 16 inches.






























