LNP1(<num>)
As in the previous function, this is supplied to supplement the
LN
function and gives a more accurate value
when x is near zero. Again, this is not something which would normally be of concern at school level.
T
T
h
h
e
e
‘
‘
C
C
a
a
l
l
c
c
u
u
l
l
u
u
s
s
’
’
g
g
r
r
o
o
u
u
p
p
o
o
f
f
f
f
u
u
n
n
c
c
t
t
i
i
o
o
n
n
s
s
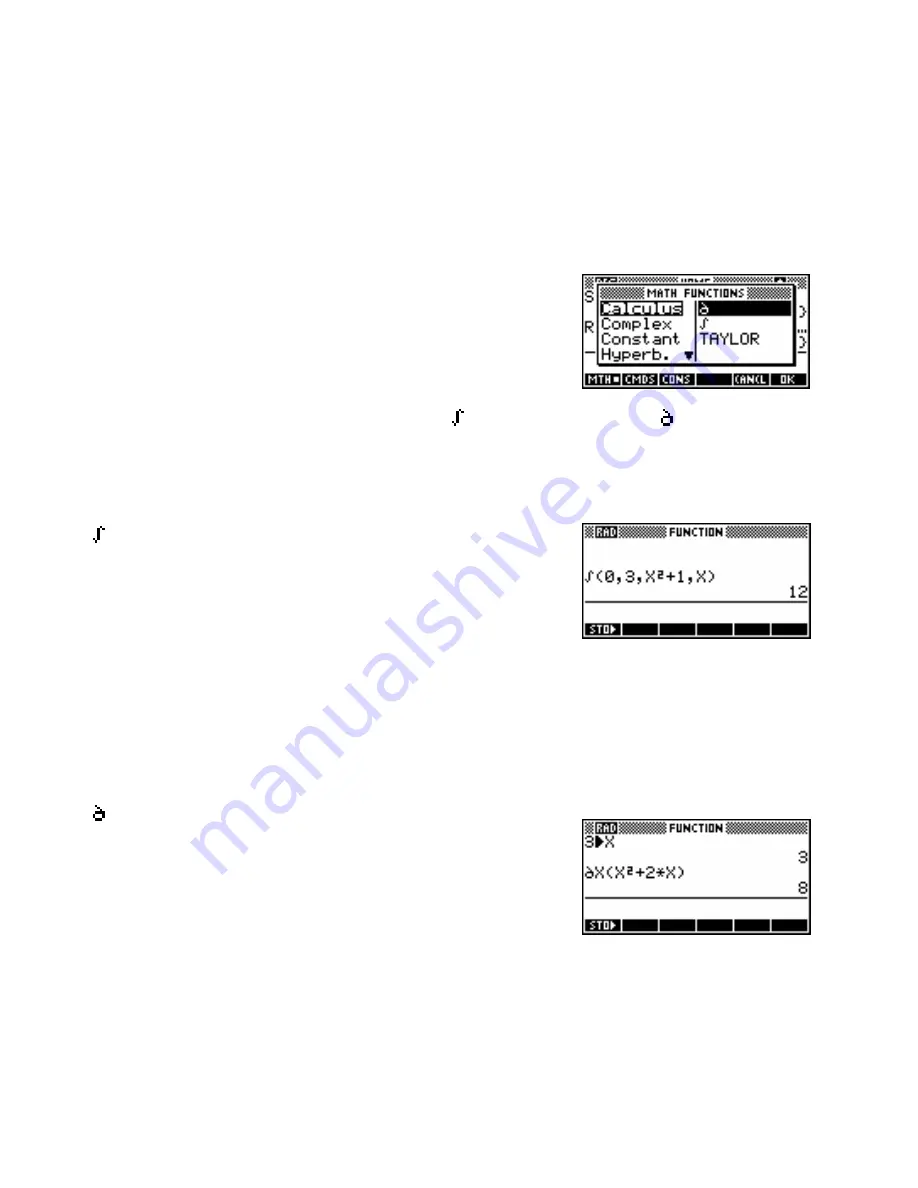
This group consists of three functions, the integrate, or
function, the differentiate, or
function and the
TAYLOR
function. The first two are discussed in detail in the chapter dealing with the Function aplet (see
pages 59 to 75) and so a brief outline only is given below.
(<num>,<num>,<expression>,<var_name>)
This function will return the definite integral of the expression when
integrated with respect to the variable specified. Any other variables in
the expression will be regarded as constants with values taken from the
current memory values.
Symbolic integration can be done in two ways. Firstly by replacing one of the limits of integration with a
symbolic variable
S1
(
S1…S5
). Secondly, and more conveniently, by doing it in the Function aplet (see
pages 59 to 75).
<var_name>(<expression>)
This function will differentiate the expression with respect to the variable
specified. This can be done in two ways. When done in the
HOME
view the result is numeric because the derivative is evaluated for the
current value of the variable in memory. For example, if
X
currently has
the value of 3 then the result is as shown right.
When done in the Function aplet, or using a symbolic variable (
S1…S5
), the result is the algebraic derivative
(see pages 59 to 75).
184






























