15
T
T
H
H
E
E
E
E
X
X
P
P
E
E
R
R
T
T
:
:
S
S
E
E
Q
Q
U
U
E
E
N
N
C
C
E
E
S
S
&
&
S
S
E
E
R
R
I
I
E
E
S
S
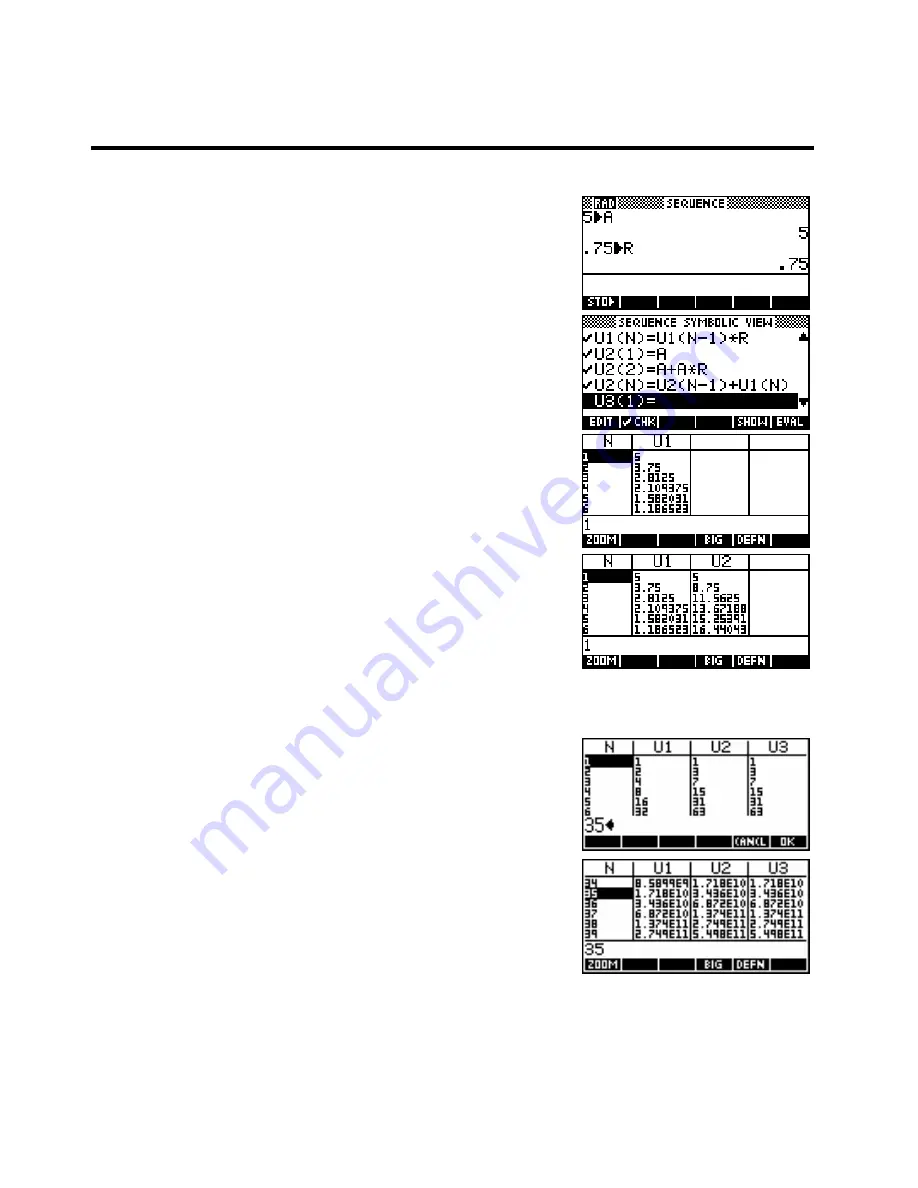
Defining a generalized GP and the sum to n terms for it.
If we define our GP using memory variables then it
becomes far more flexible.
The advantage of this method is that you now need only
change the values of
A
and
R
in the
HOME
view to
change the sequence.
Defining a series (sum to n terms of a sequence) is fairly straight-forward
using a similar method. Note the reference to
U1
in the definition of
U2
.
Once
U2
is defined in this way you can change both
U1
&
U2
. by
simply storing new values into
A
and
R
from the
HOME
view.
Solving sequence problems
Questions like “What term is the first to be greater than 10 000?”
or “When does S
n
first exceed 10 000?” can be answered in the
NUM
view.
Simply move into the
N
column, make a guess as to the term you require
and type it in. The table jumps to that value.
For example, in answer to the first question, we might estimate
N=35
.
However we would find that
U1(35)
is far too large. By successive
guesses, we find that T
15
is the one we were seeking. The second
problem is as easily solved in this way.
102






























