Section 8
277
MikroScan 7600PRO
Operator’s Manual
Catalog 11180-94
V15.4F 050406
Principle of Thermal Imaging
8.3 Blackbody Radiation
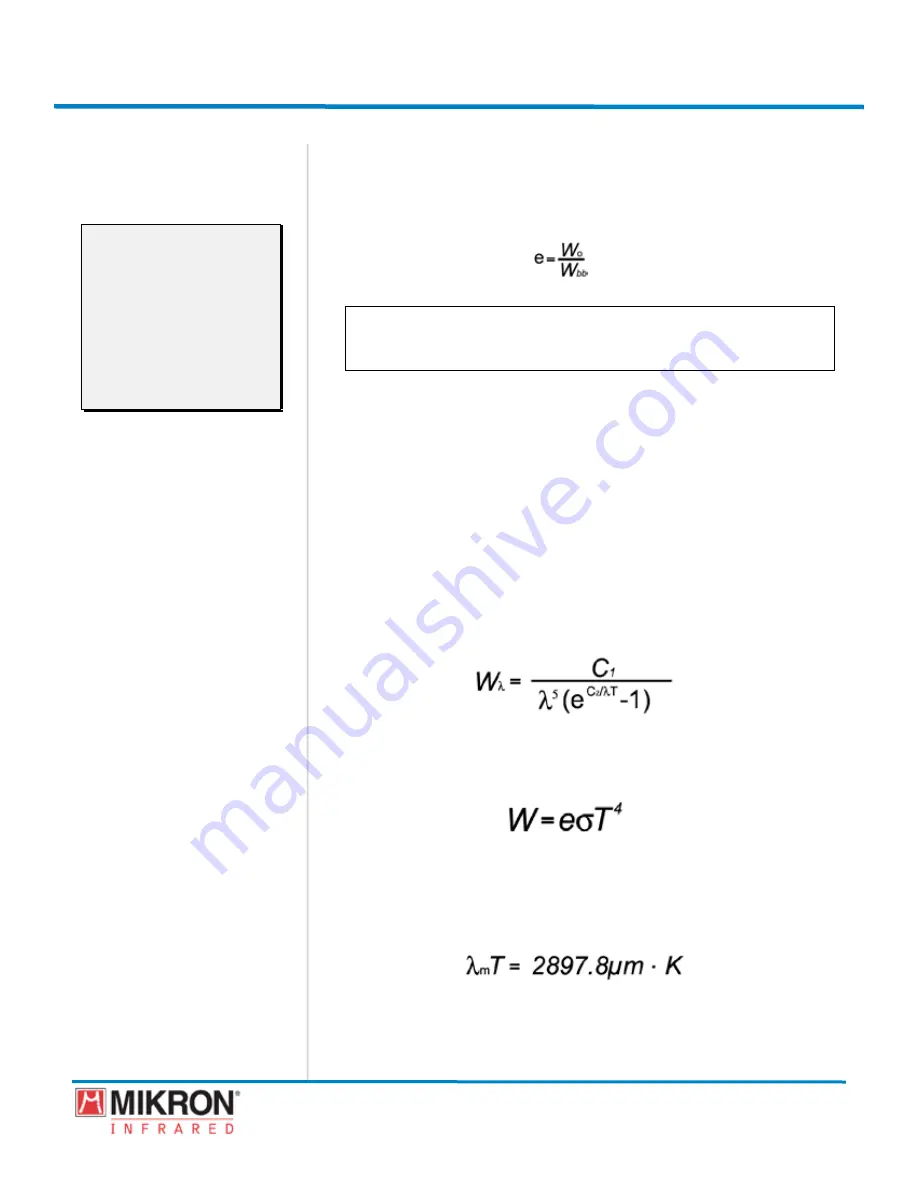
The emissivity of a body is defined formally by the equation below as the ratio of the
radiant energy emitted by the body to the radiation, which would be emitted by a black
-
body at the same temperature.
Where,
If all energy falling on an object were absorbed (no transmission or reflection), the
absorptivity would equal to 1. At a steady temperature, all the energy absorbed could be
re-radated (emtted) so that the emssvty of such a body would equal 1. Therefore n a
blackbody,
absorptivity = emissivity = 1
Practical real life objects do not behave exactly as this ideal, but as described with trans
-
missivity and reflectivity,
absorp transmis reflectivity = 1
Energy radiated from the blackbody is described as follows [“Planck’s Law”.]
In order to obtain total radiant emittance of the blackbody, integrate the equation (1)
through all wavelengths (0 to infinity). The result is as follows and is called “Stefan-
Bolzmann equaton.”
The temperature of blackbody can be obtaned drectly from the radant energy of the
blackbody by this equation. In order to find out the wavelength on the maximum spec
-
tral radiant emittance, differentiate Planck’s law and take the value to 0.
Note:
A blackbody s a theoret-
cal surface, which absorbs
and re-radates all the IR
energy t receves. It does
not reflect or transmit any
IR energy. Perfect black-
body surfaces do not exst
n nature.
W
o
=
total radant energy emtted by a body at a gven temperature T.
W
bb
=
total radant energy emtted by a blackbody at the same temperature T.
Planck’s Law
(1)
Stefan Bolzmann’s equation
(2)
(3)
wien’s displacement law
Summary of Contents for 7600PRO
Page 2: ......
















































