Page 16-55
Bessel’s equation
The ordinary differential equation x
2
⋅
(d
2
y/dx
2
) + x
⋅
(dy/dx)+ (x
2
-
ν
2
)
⋅
y = 0,
where the parameter
ν
is a nonnegative real number, is known as Bessel’s
differential equation. Solutions to Bessel’s equation are given in terms of
Bessel functions of the first kind of order
ν
:
∑
∞
=
+
+
+
Γ
⋅
⋅
⋅
−
⋅
=
0
2
2
,
)
1
(
!
2
)
1
(
)
(
m
m
m
m
m
m
x
x
x
J
ν
ν
ν
ν
where
ν
is not an integer, and the function Gamma
Γ
(
α
) is defined in Chapter
3.
If
ν
= n, an integer, the Bessel functions of the first kind for n = integer are
defined by
∑
∞
=
+
+
⋅
⋅
⋅
−
⋅
=
0
2
2
.
)!
(
!
2
)
1
(
)
(
m
n
m
m
m
n
n
m
n
m
x
x
x
J
Regardless of whether we use
ν
(non-integer) or n (integer) in the calculator,
we can define the Bessel functions of the first kind by using the following finite
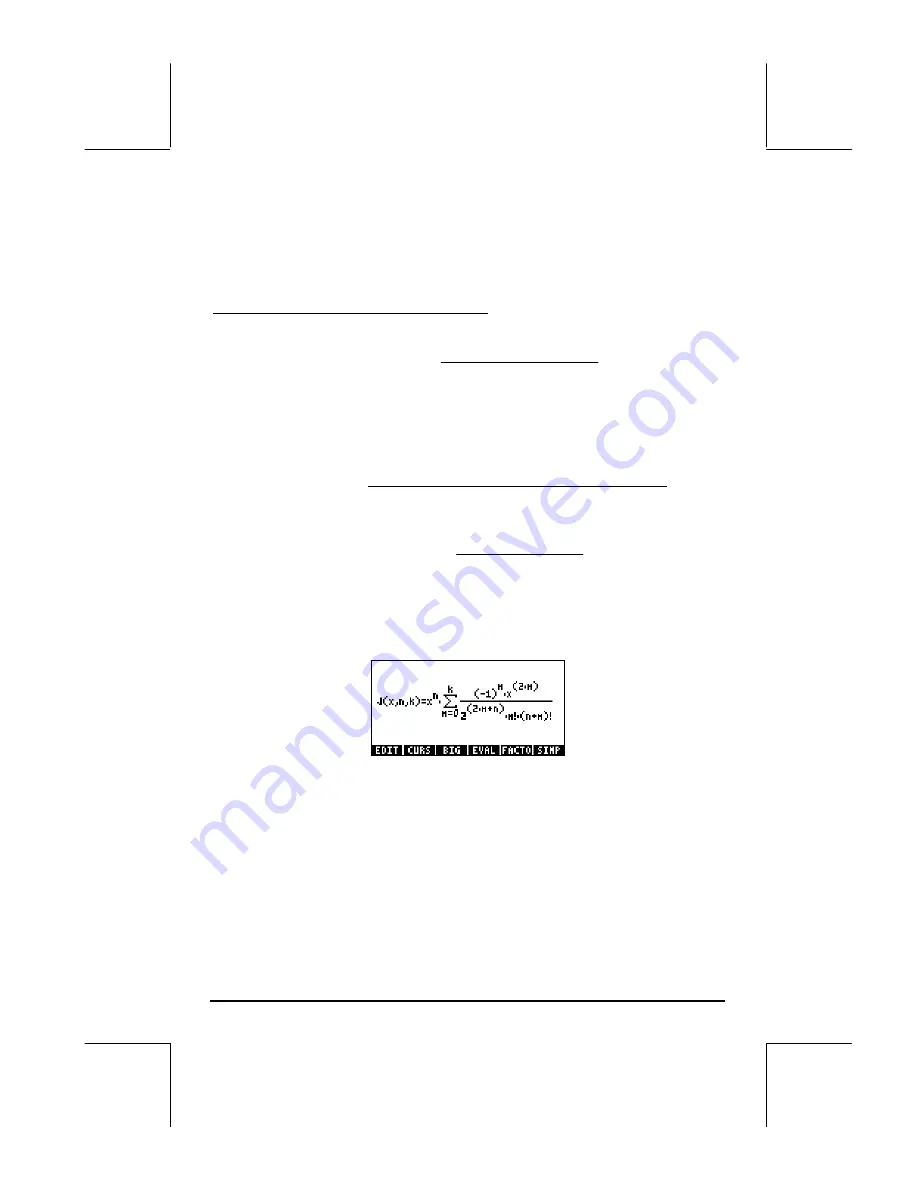
series:
Thus, we have control over the function’s order, n, and of the number of
elements in the series, k. Once you have typed this function, you can use
function DEFINE to define function J(x,n,k). This will create the variable
@@@J@@@
in the soft-menu keys. For example, to evaluate J
3
(0.1) using 5 terms in the
series, calculate J(0.1,3,5), i.e., in RPN mode:
.1#3#5
@@@J@@@
The result is 2.08203157E-5.






























