Page 13-7
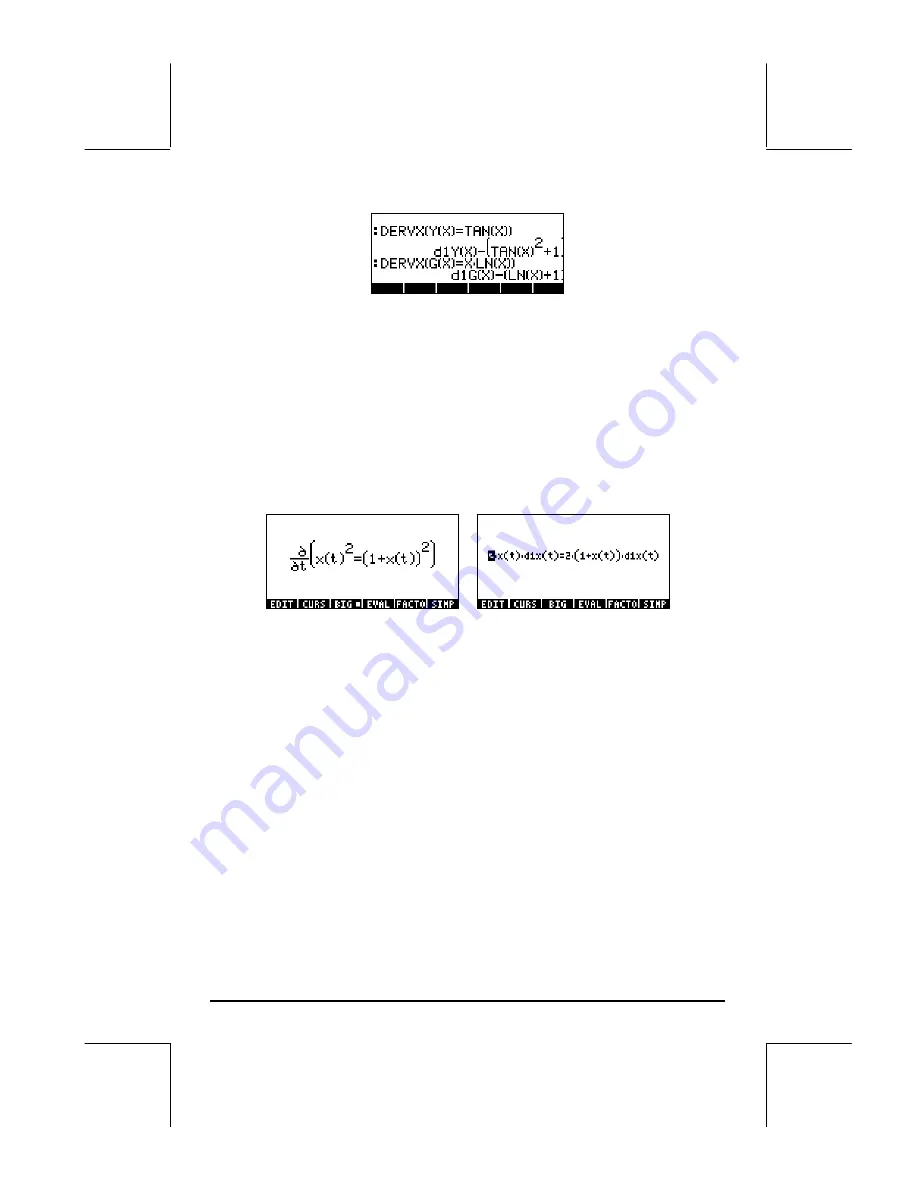
Notice that in the expressions where the derivative sign (
∂
) or function DERIV
was used, the equal sign is preserved in the equation, but not in the cases
where function DERVX was used. In these cases, the equation was re-written
with all its terms moved to the left-hand side of the equal sign. Also, the equal
sign was removed, but it is understood that the resulting expression is equal to
zero.
Implicit derivatives
Implicit derivatives are possible in expressions such as:
Application of derivatives
Derivatives can be used for analyzing the graphs of functions and for
optimizing functions of one variable (i.e., finding maxima and minima).
Some applications of derivatives are shown next.
Analyzing graphics of functions
In Chapter 11 we presented some functions that are available in the graphics
screen for analyzing graphics of functions of the form y = f(x). These
functions include (X,Y) and TRACE for determining points on the graph, as
well as functions in the ZOOM and FCN menu. The functions in the ZOOM
menu allow the user to zoom in into a graph to analyze it in more detail.
These functions are described in detail in Chapter 12. Within the functions of
the FCN menu, we can use the functions SLOPE, EXTR, F’, and TANL to
determine the slope of a tangent to the graph, the extrema (minima and






























