HP 49g+, User Manual, Page: 137 / 862

Page 3-15
GAMMA: The Gamma function
Γ
(
α
)
PSI: N-th derivative of the digamma function
Psi: Digamma function, derivative of the ln(Gamma)
The Gamma function is defined by
∫
∞
− −
= Γ
0
1
) (
dx e x
x α
α
. This function has
applications in applied mathematics for science and engineering, as well as
in probability and statistics.
Factorial of a number
The factorial of a positive integer number n is defined as n!=n
⋅
(n-1)
⋅
(n-
2) …3
⋅
2
⋅
1 , with 0! = 1 . The factorial function is available in the calculator by
using
~‚2
. In both ALG and RPN modes, enter the number first,
followed by the sequence
~‚2
. Example:
5~‚2`
.
The Gamma function, defined above, has the property that
Γ(α) = (α−1) Γ(α−1)
, for
α
> 1.
Therefore, it can be related to the factorial of a number, i.e.,
Γ(α) = (α−1) !,
when
α
is a positive integer. We can also use the factorial function to
calculate the Gamma function, and vice versa. For example,
Γ
(5) = 4! or,
4~‚2`
. The factorial function is available in the MTH menu,
through the 7. PROBABILITY.. menu.
The PSI function,
Ψ
(x,y) , represents the y-th derivative of the digamma function,
i.e.,
) ( ) , (
x
dx
d
x n
n
n
ψ = Ψ
, where
ψ
(x) is known as the digamma function, or
Psi function. For this function, y must be a positive integer.
The Psi function,
ψ
(x) , or digamma function, is defined as
)] ( ln[ ) ( x x Γ = ψ
.
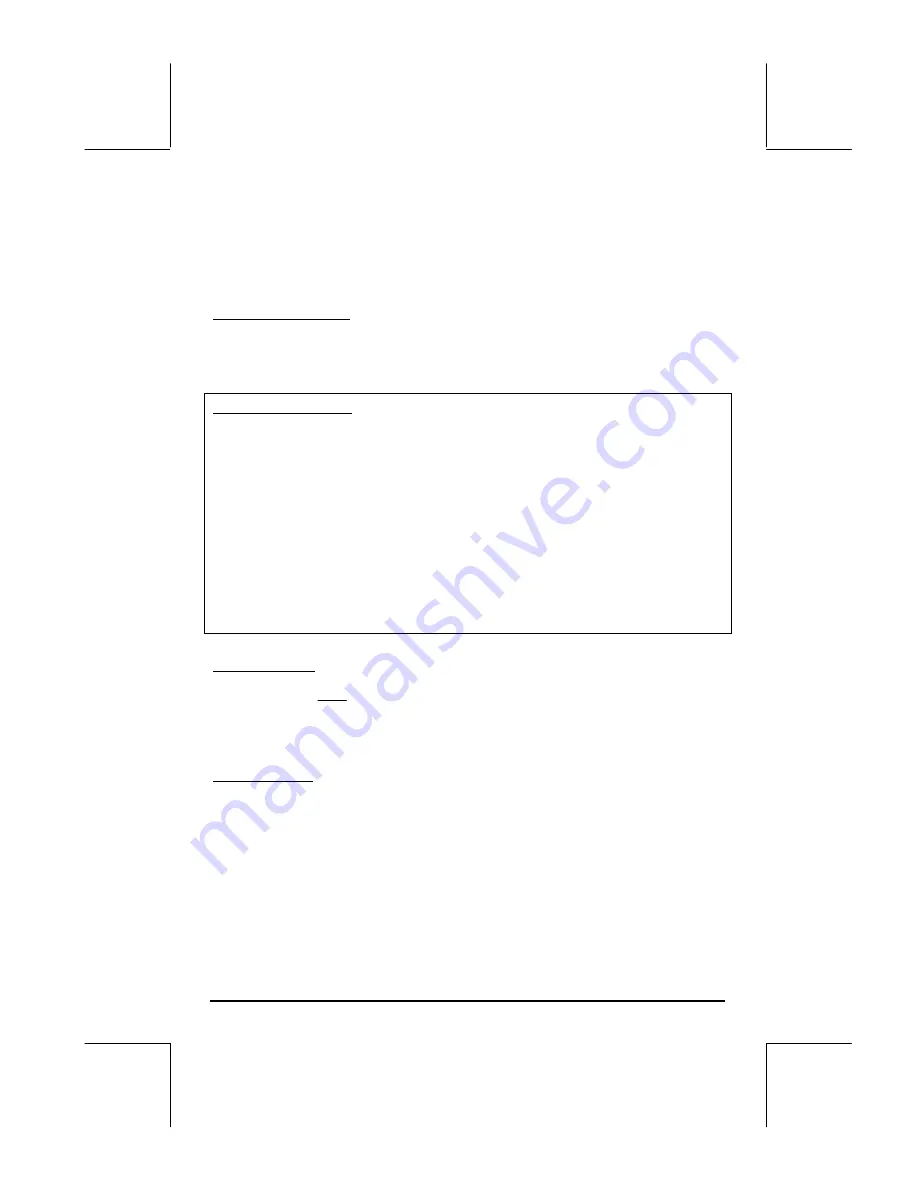
Examples of these special functions are shown here using both the ALG and
RPN modes. As an exercise, verify that
GAMMA(2.3) = 1.166711…,
PSI(1.5,3) = 1.40909.., and Psi(1.5) = 3.64899739..E-2 .
These calculations are shown in the following screen shot: