Concepts and features
R&S
®
ZNB/ZNBT
145
User Manual 1173.9163.02 ─ 62
Upper and lower limit lines are both defined as a combination of segments with a linear
or logarithmic dependence between the measured quantity and the sweep variable
(stimulus variable). Similar to this segmentation, ripple limits can be defined in several
ranges. The limit lines (except circle limits) can be stored to a file and recalled. Data or
memory traces can be used to define the segments of an upper or lower limit line.
Moreover it is possible to modify the upper and lower limit lines globally by adding an
offset to the stimulus or response values.
4.4.1.1
Rules for limit line definition
The analyzer places few restrictions on the definition of limit line segments.
The following rules ensure a maximum of flexibility:
●
Segments do not have to be sorted in ascending or descending order (e.g. the
"Start Stimulus" value of segment no. n does not have to be smaller than the "Start
Stimulus" value of segment no. n+1).
●
Overlapping segments are allowed. The limit check in the overlapping area is rela-
ted to the tighter limit (the pass test involves a logical AND operation).
●
Gaps between segments are allowed and equivalent to switching off an intermedi-
ate limit line segment.
●
Limit lines can be partially or entirely outside the sweep range, however, the limits
are only checked at the measurement points.
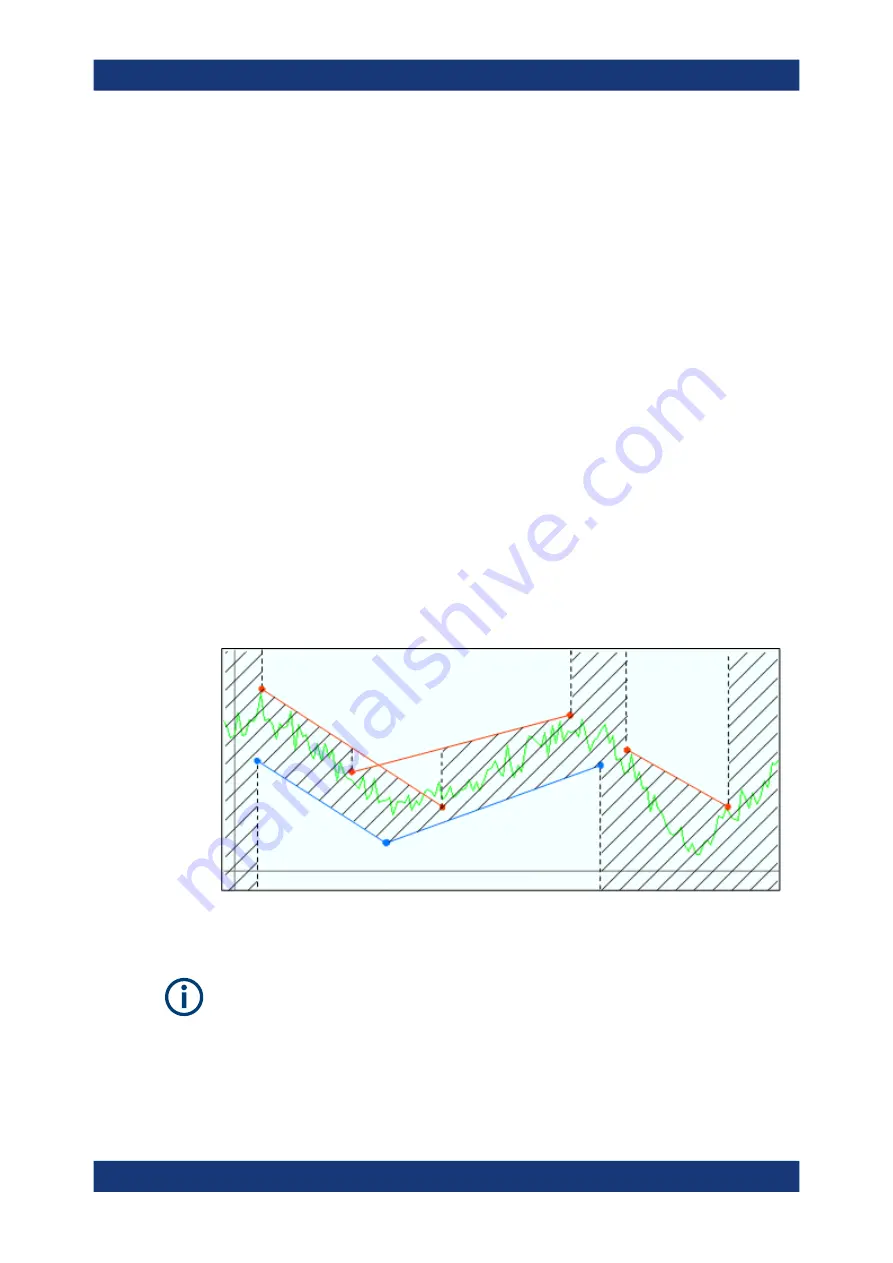
The following figure shows a limit line consisting of 3 upper and 2 lower limit line seg-
ments. To pass the limit check, the trace must be confined to the shaded area.
As a consequence of the limit line rules, a DUT always passes the limit check if no limit
lines are defined.
Formula-defined limit lines
A limit line doesn't have to be straight. You can also use a custom formula to define it.
When the sweep axis is changed from linear frequency sweep to logarithmic sweeps,
straight limit lines are transformed into exponential curves. The sweep points are redis-
tributed along the x-axis, so the number of failed points can change.
Operations on traces






























