HP 50G, User Manual

Page: 483 / 887

Page 16-6
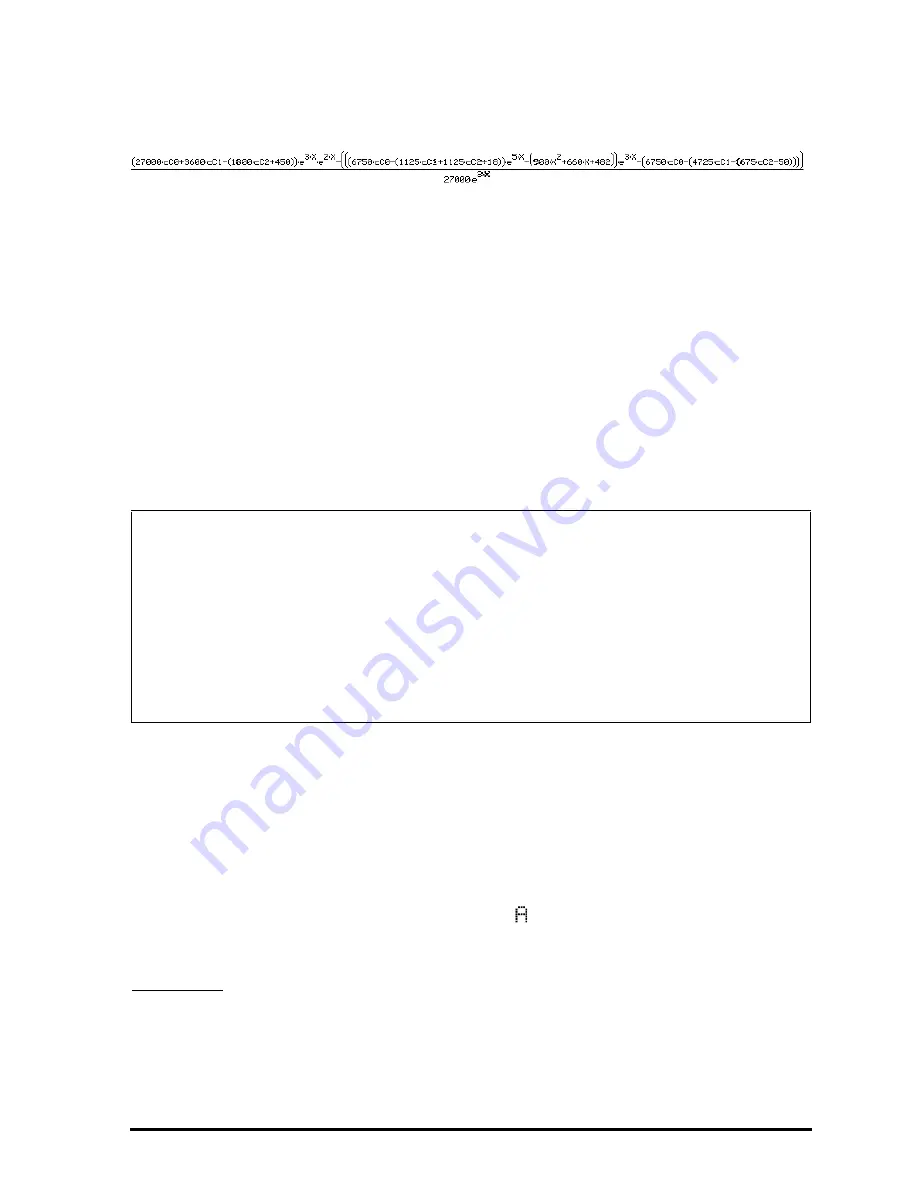
The solution, shown partially here in the Equation Writer, is:
Replacing the combination of constants accompanying the exponential terms
with simpler values, the expression can be simplified to y = K
1
⋅
e
–3x
+ K
2
⋅
e
5x
+
K
3
⋅
e
2x
+ (450
⋅
x
2
+330
⋅
x+241)/13500.
We recognize the first three terms as the general solution of the homogeneous
equation (see Example 1, above). If y
h
represents the solution to the
homogeneous equation, i.e., y
h
= K
1
⋅
e
–3x
+ K
2
⋅
e
5x
+ K
3
⋅
e
2x
. You can prove
that the remaining terms in the solution shown above, i.e., y
p
=
(450
⋅
x
2
+330
⋅
x+241)/13500, constitute a particular solution of the ODE.
To verify that y
p
= (450
⋅
x
2
+330
⋅
x+241)/13500, is indeed a particular solution
of the ODE, use the following:
'd1d1d1Y(X)-4*d1d1Y(X)-11*d1Y(X)+30*Y(X) = X^2'
`
'Y(X)=(450*X^2+330*X+241)/13500'
`
SUBST EV L
Allow the calculator about ten seconds to produce the result: ‘X^2 = X^2’.
Example 3 - Solving a system of linear differential equations with constant
coefficients.
Consider the system of linear differential equations:
x
1
’(t) + 2x
2
’(t) = 0,
Note : This result is general for all non-homogeneous linear ODEs, i.e., given
the solution of the homogeneous equation, y
h
(x), the solution of the
corresponding non-homogeneous equation, y(x), can be written as
y(x) = y
h
(x) + y
p
(x),
where y
p
(x) is a particular solution to the ODE.