HP 50G, User Manual

Page: 475 / 887

Page 15-5
Curl
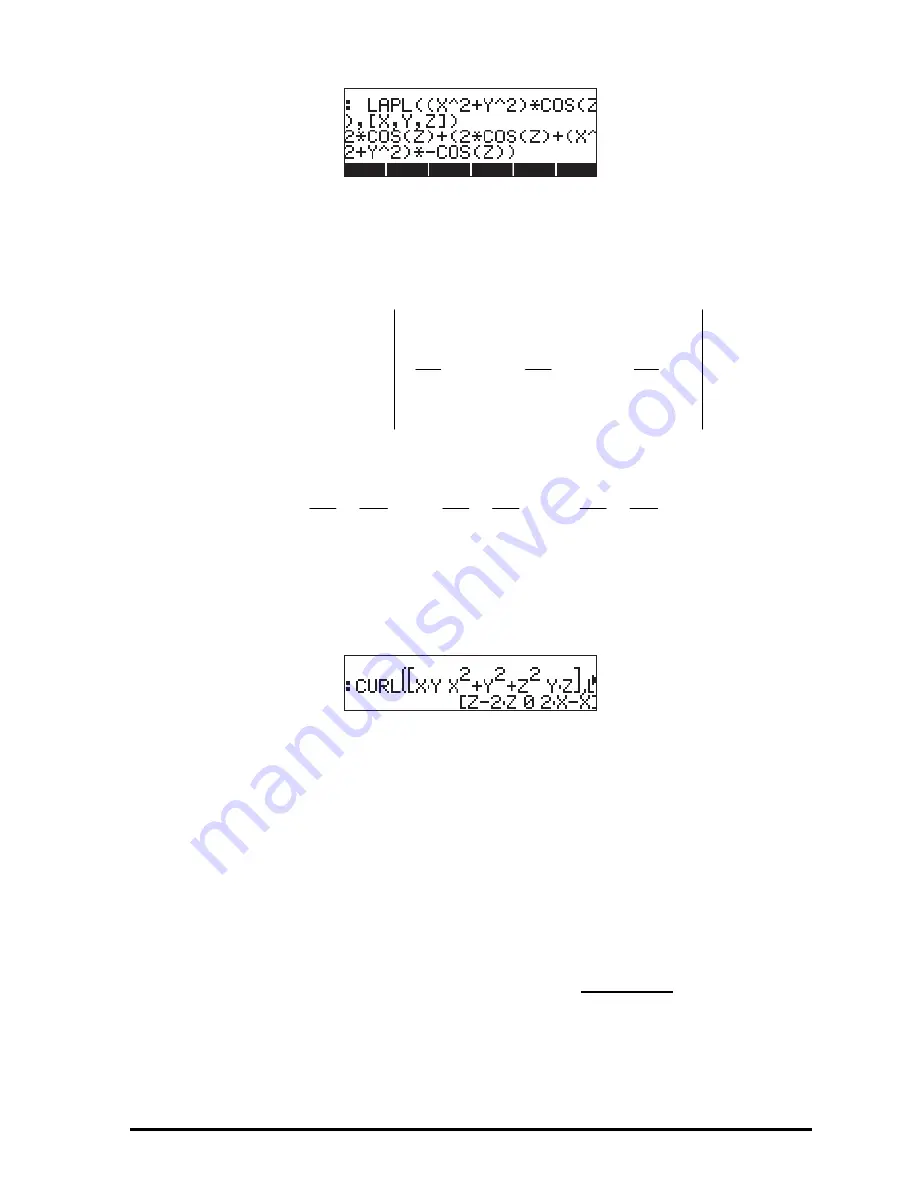
The curl of a vector field F (x,y,z) = f(x,y,z) i +g(x,y,z) j +h(x,y,z) k , is defined by a
“cross-product” of the del operator with the vector field, i.e.,
The curl of vector field can be calculated with function CURL. For example, for
the function
F (X,Y,Z) = [XY,X
2
+Y
2
+Z
2
,YZ], the curl is calculated as follows:
Irrotational fields and potential function
In an earlier section in this chapter we introduced function POTENTIAL to
calculate the potential function
φ
(x,y,z) for a vector field, F (x,y,z) = f(x,y,z) i +
g(x,y,z) j + h(x,y,z) k , such that F = grad
φ
=
∇φ
. We also indicated that the
conditions for the existence of
φ
, were:
∂
f/
∂
y =
∂
g/
∂
x,
∂
f/
∂
z =
∂
h/
∂
x, and
∂
g/
∂
z =
∂
h/
∂
y. These conditions are equivalent to the vector expression
curl F =
∇×
F = 0.
A vector field F (x,y,z), with zero curl, is known as an irrotational field. Thus, we
conclude that a potential function
φ
(x,y,z) always exists for an irrotational field
F (x,y,z).
[ ] [ ] [ ]
) , , ( ) , , ( ) , , ( z y x h z y x g z y x f
z y x
curl
∂
∂
∂
∂
∂
∂
= × ∇ =
k j i
F F
⎟⎟⎠
⎞
⎜⎜⎝
⎛
∂
∂
−
∂
∂
+
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
−
∂
∂
+
⎟⎟⎠
⎞
⎜⎜⎝
⎛
∂
∂
−
∂
∂
=
z
g
y
h
x
h
z
f
z
g
y
h
k j i