HP 50G, User Manual

Page: 388 / 887

Page 12-5
Some useful PLOT operations for FUNCTION plots
In order to discuss these PLOT options, we'll modify the function to force it to
have some real roots (Since the current curve is totally contained above the x
axis, it has no real roots.) Press
‚ @@@Y1@@
to list the contents of the function Y1
on the stack: <<
→
X ‘EXP(-X^2/2)/
√
(2
*
π
)
‘ >>. To edit this expression use:
˜
Launches the line editor
‚˜
Moves cursor to the end of the line
ššš-0.1
Modifies the expression
`
Returns to calculator display
Next, store the modified expression into variable y by using
„ @@@Y1@@
if in RPN
mode, or
„îK @@@Y1@@
in ALG mode.
The function to be plotted is now,
Enter the PLOT WINDOW environment by entering
„ò
(press them
simultaneously if in RPN mode.) Keep the range of –4 to 4 for H-VIEW, press
˜ @AUTO
to generate the V-VIEW. To plot the graph, press
@ERASE @DRAW
Θ
Once the graph is plotted, press
@)@FCN!
to access the function menu.
With this menu you can obtain additional information about the plot
such as intersects with the x-axis, roots, slopes of the tangent line, area
under the curve, etc.
Θ
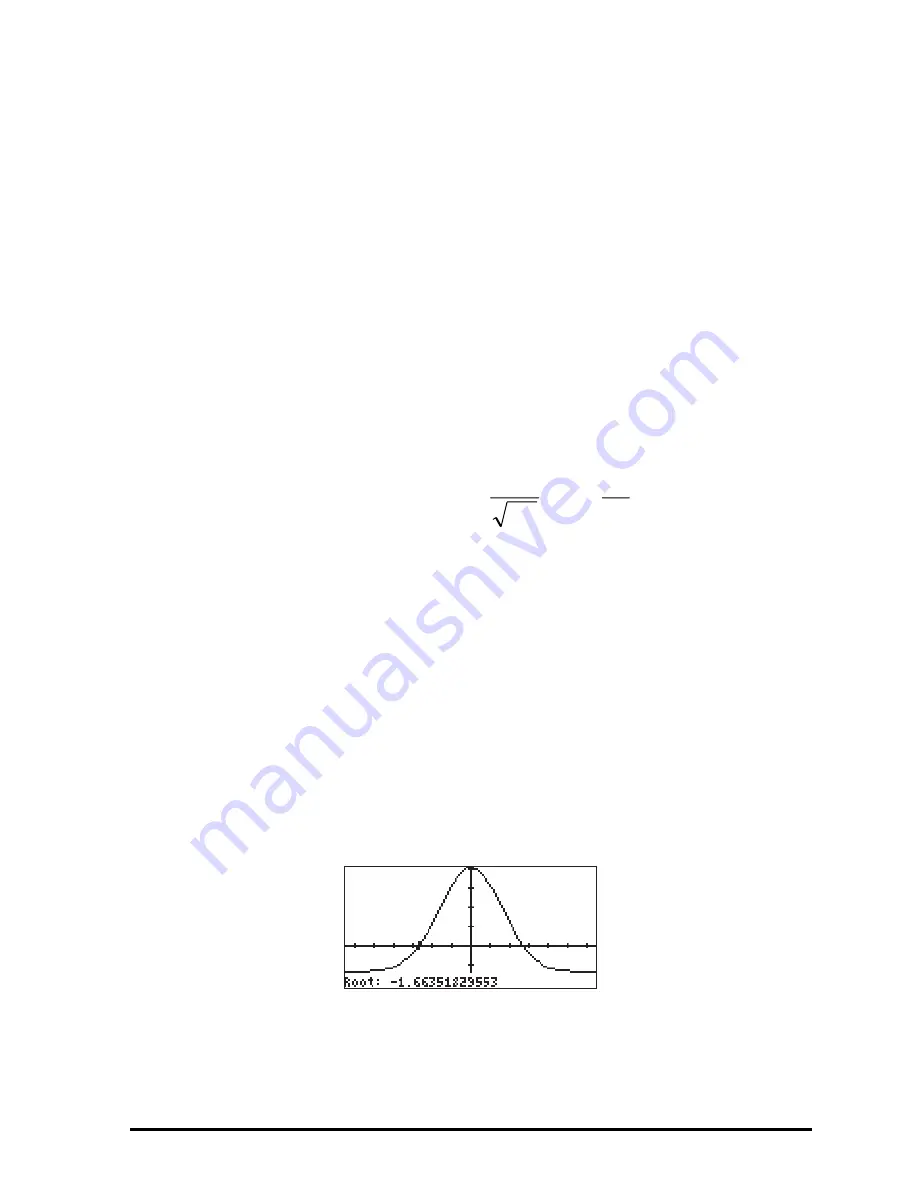
For example, to find the root on the left side of the curve, move the
cursor near that point, and press
@ROOT
. You will get the result: ROOT: -
1.6635…. Press
L
to recover the menu. Here is the result of
ROOT in the current plot:
Θ
If you move the cursor towards the right-hand side of the curve, by
pressing the right-arrow key (
™
), and press
@ROOT
, the result now is
1 . 0 )
2
exp(
2
1
) (
2
− − =
x
x f
π