HP 50G, User Manual

Page: 225 / 887

Page 6-19
Θ
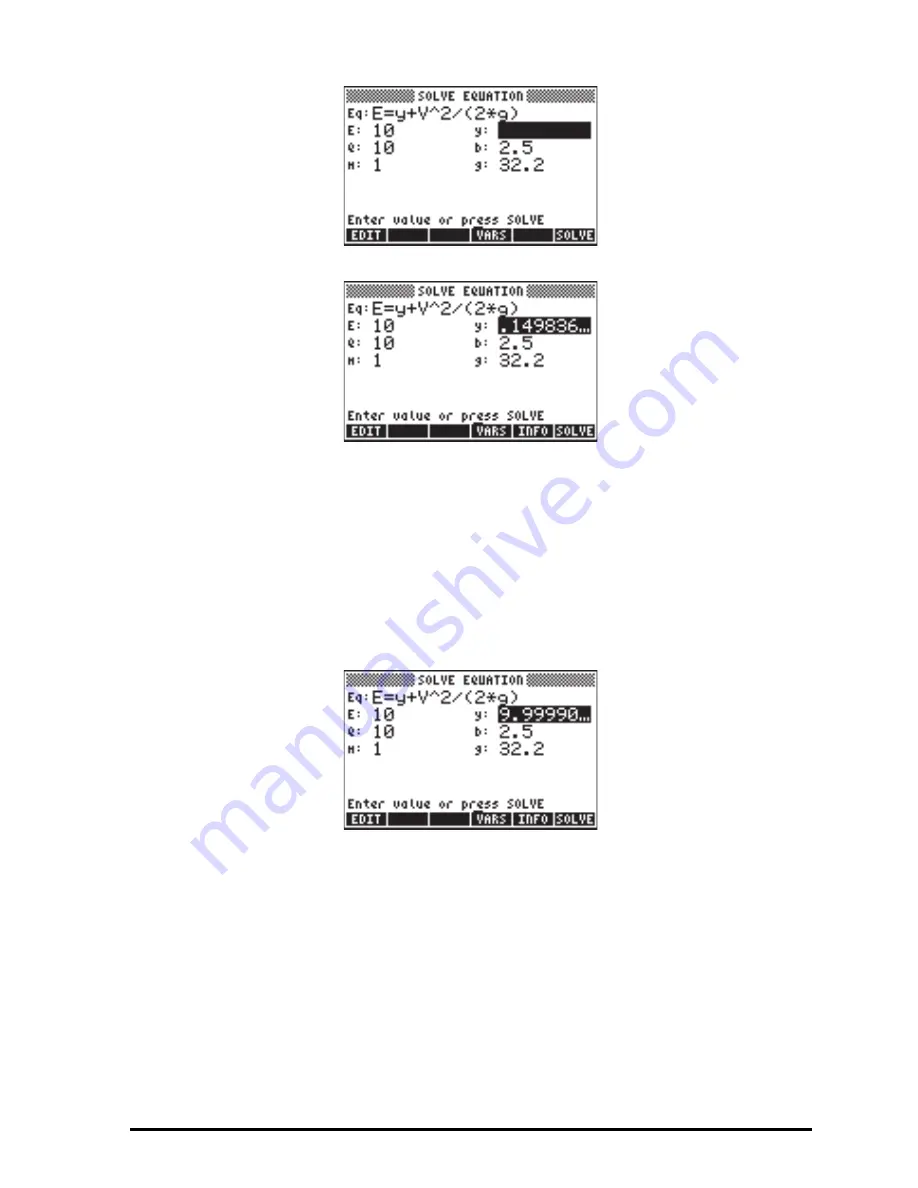
Solve for y.
The result is 0.149836.., i.e., y = 0.149836.
Θ
It is known, however, that there are actually two solutions available for
y in the specific energy equation. The solution we just found
corresponds to a numerical solution with an initial value of 0 (the
default value for
y , i.e., whenever the solution field is empty, the initial
value is zero). To find the other solution, we need to enter a larger
value of y, say
15 ,
highlight the y input field and solve for y once
more:
The result is now 9.99990, i.e., y = 9.99990 ft.
This example illustrates the use of auxiliary variables to write complicated
equations. When NUM.SLV is activated, the substitutions implied by the
auxiliary variables are implemented, and the input screen for the equation
provides input field for the primitive or fundamental variables resulting from the
substitutions. The example also illustrates an equation that has more than one
solution, and how choosing the initial guess for the solution may produce those
different solutions.