HP 50G, User Manual

Page: 465 / 887

Page 14-5
A different version of the chain rule applies to the case in which z = f(x,y), x =
x(u,v), y = y(u,v), so that z = f[x(u,v), y(u,v)]. The following formulas represent
the chain rule for this situation:
Determining extrema in functions of two variables
In order for the function z = f(x,y) to have an extreme point (extrema) at (x
o
,y
o
),
its derivatives
∂
f/
∂
x and
∂
f/
∂
y must vanish at that point. These are necessary
conditions. The sufficient conditions for the function to have an extreme at point
(x
o
,y
o
) are
∂
f/
∂
x = 0,
∂
f/
∂
y = 0, and
Δ
= (
∂
2
f/
∂
x
2
)
⋅
(
∂
2
f/
∂
y
2
)-[
∂
2
f/
∂
x
∂
y]
2
> 0.
The point (x
o
,y
o
) is a relative maximum if
∂
2
f/
∂
x
2
< 0, or a relative minimum if
∂
2
f/
∂
x
2
> 0. The value
Δ
is referred to as the discriminant.
If
Δ
= (
∂
2
f/
∂
x
2
)
⋅
(
∂
2
f/
∂
y
2
)-[
∂
2
f/
∂
x
∂
y]
2
< 0, we have a condition known as a
saddle point , where the function would attain a maximum in x if we were to
hold y constant, while, at the same time, attaining a minimum if we were to
hold x constant, or vice versa.
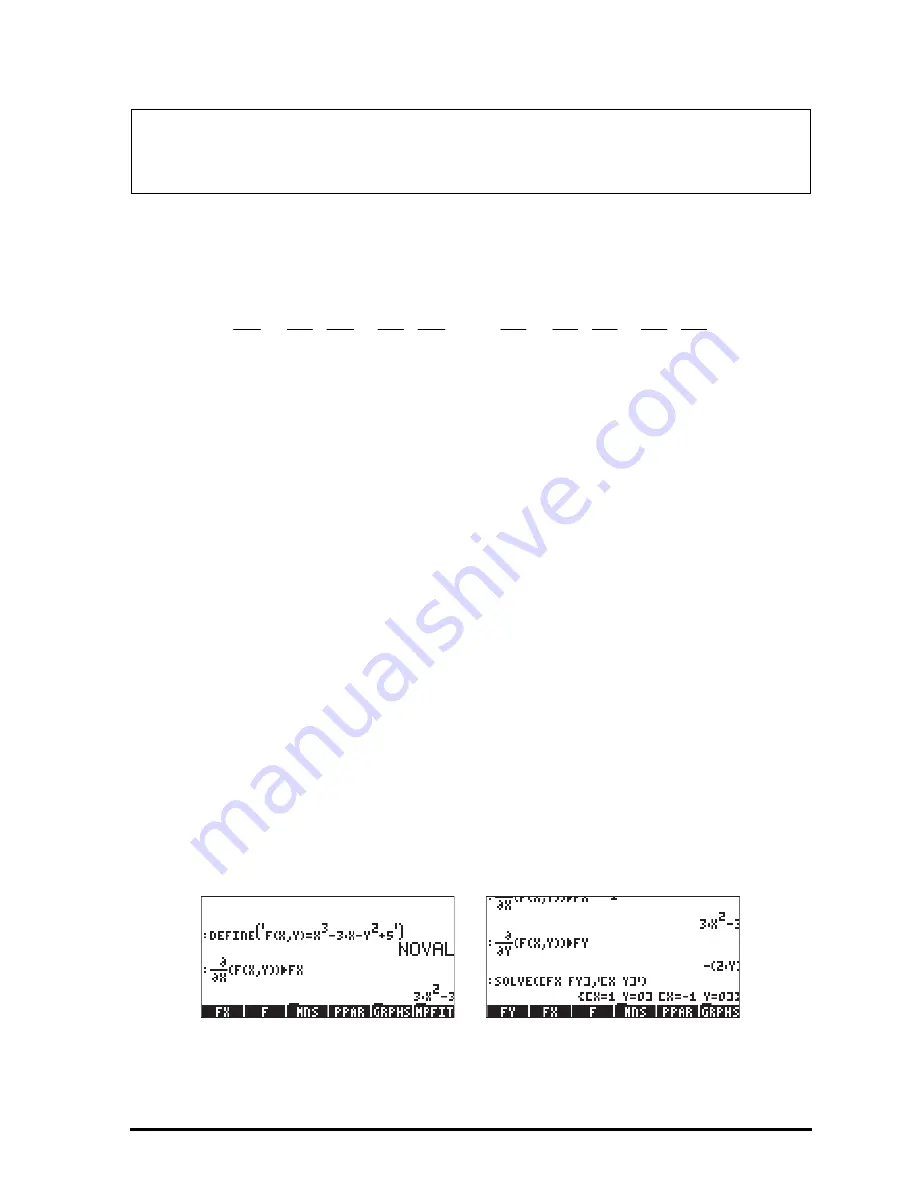
Example 1 – Determine the extreme points (if any) of the function f(X,Y) = X
3
-3X-
Y
2
+5. First, we define the function f(X,Y), and its derivatives fX(X,Y) =
∂
f/
∂
X,
fY(X,Y) =
∂
f/
∂
Y. Then, we solve the equations fX(X,Y) = 0 and fY(X,Y) = 0,
simultaneously:
Total differential of a function z = z(x,y)
From the last equation, if we multiply by dt, we get the total differential of the
function z = z(x,y), i.e., dz =
(
∂
z/
∂
x)
⋅
dx + (
∂
z/
∂
y)
⋅
dy.
v
y
y
z
v
x
x
z
v
z
u
y
y
z
u
x
x
z
u
z
∂
∂
⋅
∂
∂
+
∂
∂
⋅
∂
∂
=
∂
∂
∂
∂
⋅
∂
∂
+
∂
∂
⋅
∂
∂
=
∂
∂
,