Gradient at a point as the limit of the slope of a chord
PLOT
view or via the
δ
differentiation operator. For students first being introduced to calculus a common task
is to investigate the slope of the chord joining two points as the length of the chord tends towards zero.
The true gradient at a point is available in a number of ways. For example, via the
Slope
tool in the
⎛
f
(
x
h
f x
)
⎞
+
)
−
(
ie.
lim
⎜
⎟
h
→
0
⎝
h
⎠
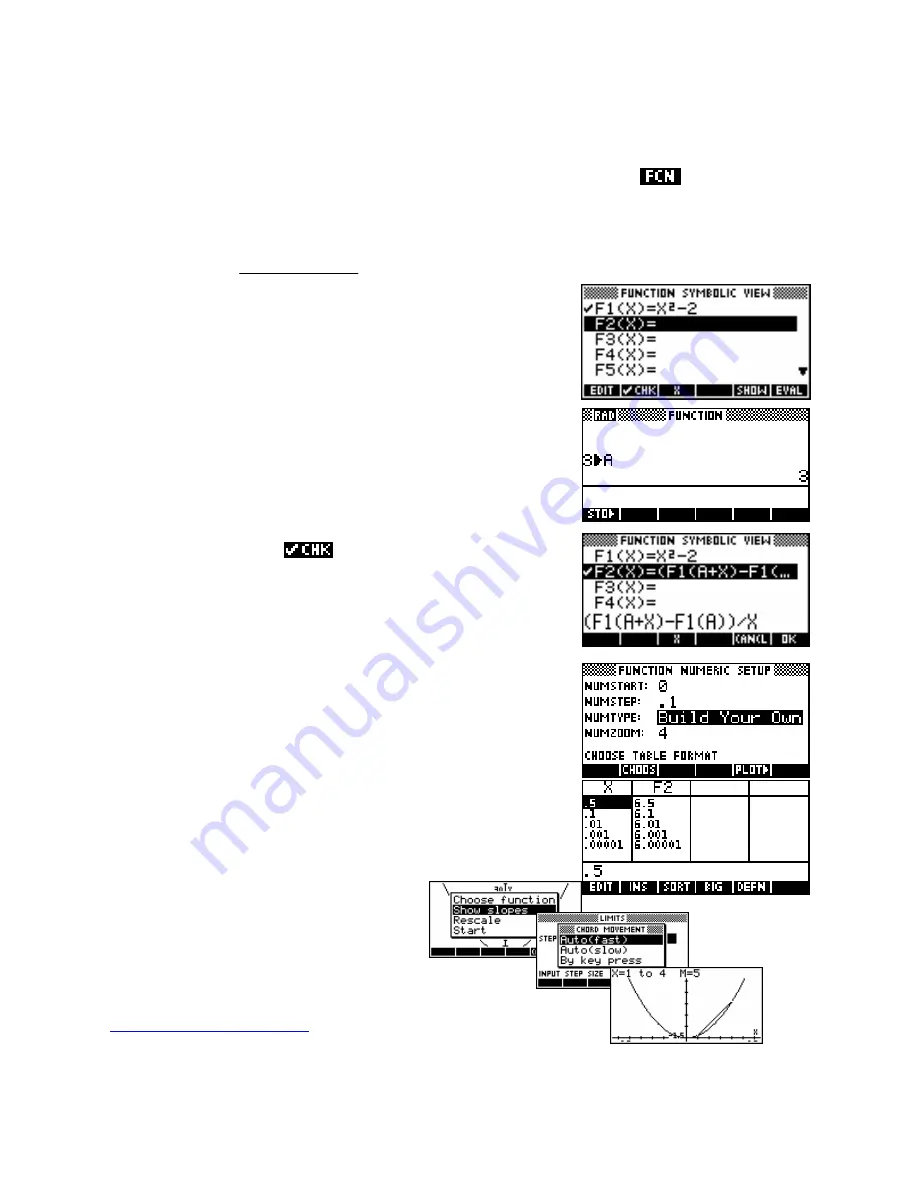
This can be effectively introduced via the Function aplet.
Begin by entering the function being studied into
F1(X)
as shown.
To examine the gradient at x=3, store 3 into memory
A
in the
HOME
view as shown right.
Return to the
SYMB
view, un-
the function
F1(X)
and enter the
expression:
F2(X)=(F1(A+X)-F1(A))/X
in
F2(X)
.
This is the basic differentiation formula quoted above with
X
taking the
role of
h
and
A
being the point of evaluation, in this case with
A = 3
.
Change to the
NUM SETUP
view and change the
NumType
to “Build
Your Own”. By entering successively smaller values for
X
you can now
investigate the limit as
h
tends towards zero.
In this case it is clear that the limit for x=3 is the value 6.
To investigate the gradient at a different point simply change back to the
HOME
view, enter a new value into
A
and then return to the
NUM
view.
The disadvantage of the previous method is that
it is not very visual. An alternative is to use an
aplet downloaded from the web. An aplet that
will automate the process and provide a visual display of the
chord diminishing in length can be found on the author’s website
at
http://www.hphomeview.com
.
83






























