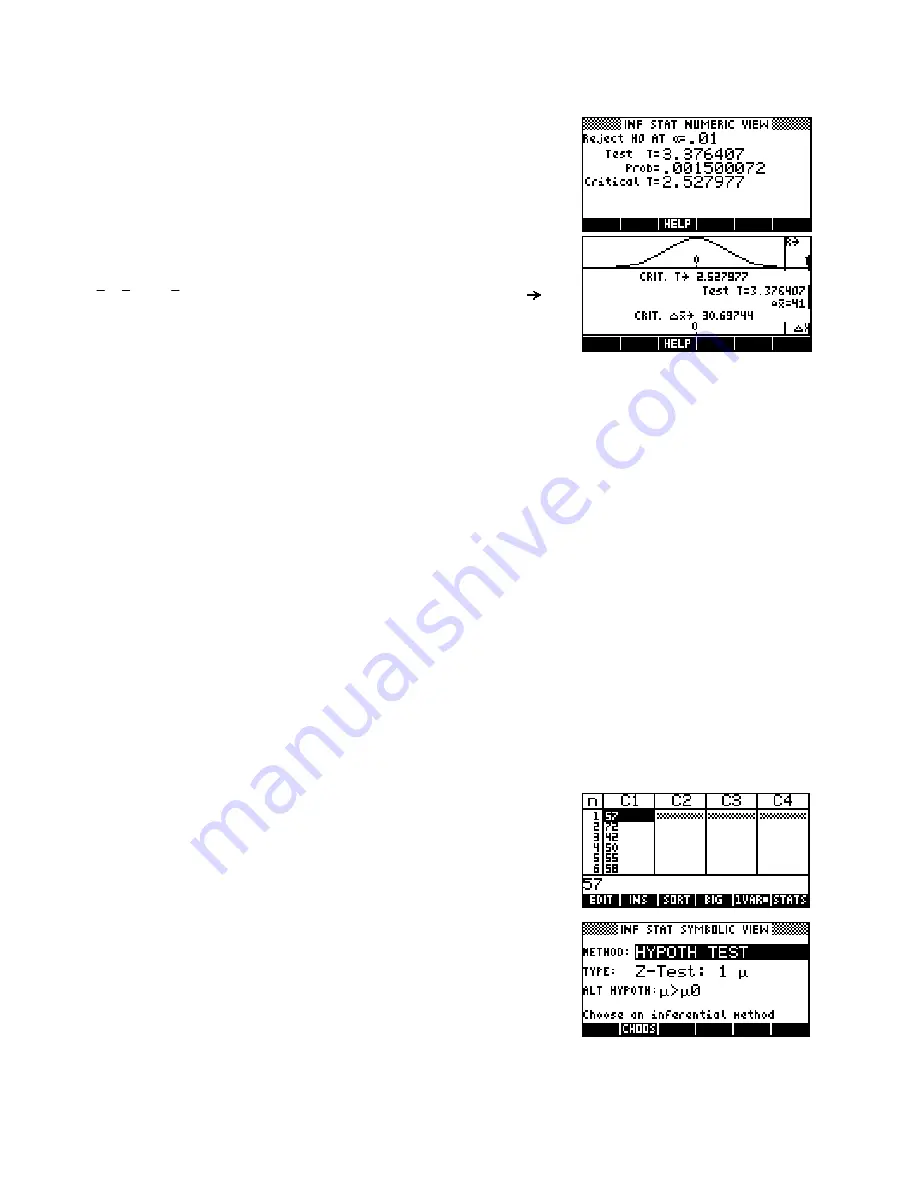
The
PLOT
.
probability of obtaining a test student-t value of 3.38 is 0.0015 and this
view also shows that the vertical line representing the value of
The
NUM
view shows the critical values. We can see that the
is well below the permitted test level of 1%.
x
1
−
x
2
or
∆
x
is well into the region of rejection indicated by the R
From the evidence we should reject the null hypothesis and accept instead that the average weight of the
group receiving supplement A is significantly higher than those receiving supplement C with only a 1% chance
of being incorrect.
Hypothesis test: Z-Test 1-
µ
A teacher has developed a new teaching technique for hearing-impaired students
which he believes is producing significantly better results. He wishes to publish a
paper on this and needs to check his results statistically.
A standardized test is available for which it is known that the normal performance of
hearing-impaired students at the same stage of study has a mean of 53.6% and a
standard deviation of 12.2%. When he applies this test to his class of 23 students
their scores are shown below. The teacher believes that this data shows that his
students are scoring significantly better and wishes to test this at a level of 5%.
Test results:
{57, 72, 42, 50, 55, 58, 59, 38, 45,53, 77,57, 52, 69, 50, 55, 59, 68, 62, 63, 53, 56}
As usual we begin by entering the data into column
C1
of the Statistics
aplet.
Changing to the Inference aplet, we choose a Hypothesis test using
Z-
test: 1
µ
, since we know the population standard deviation.
145






























