FujiFilm FRENIC5000G11S Series, Technical Information

Page: 163 / 236

Chapter 4
1. Inverter and Motor Selection
4-9
4
e e e e e
For a load running horizontally
As shown in Fig. 4.7, a carrier table can be driven by a
motor. If the table speed is
υ
[m/s] when the motor
rotation speed is N
M
[r/min], an equivalent distance
from the rotation axis is 60
υ
/(2
π
·N
M
) [m]. Then, the
moment of inertia of table and load to the rotation axis
is calculated as follows:
J =
60
υ
( 2
π
· N
M
)
2
· (W
0
+ W) [kg · m
2
] ................... (4.13)
r r r r r
For lifting and lowering load
As shown in Figures 4.8 and 4.9, two loads tied with
the rope move in different directions. The moment of
inertia can be calculated by obtaining the sum of the
moving object’s weight as follows:
60
υ
J = ( 2
π
· N
M
)
2 · (W
0
+ W + W
B
) [kg · m
2
] ............ (4.14)
2. Calculation of the acceleration time
Fig.4.10 shows a general load model. Here, the load is
tied via a reduction-gear with efficiency
η
G
.
The time required to accelerate this load to a speed of
N
M
[r/min] is calculated with the following expression:
t
ACC
= J
1
+ J
2
/
η
G
· 2
π
· (N
M
– 0)
τ
M
–
τ
L
/
η
G
60
[s] .............................. (4.15)
Where,
J
1
: Motor shaft moment of inertia [kg·m
2
]
J
2
: Load shaft moment of inertia converted to motor shaft
[kg·m
2
]
τ
M
: Minimum motor output torque in driving mode [N·m]
τ
L
: Maximum load torque converted to motor shaft [N·m]
η
G
: Reduction-gear efficiency
As clarified in the above expression, equivalent moment
of inertia becomes (J
1
+J
2
/
η
G
) considering the reduction
gear efficiency.
3. Calculation of the deceleration time
In Fig. 4.10, the time required to stop the motor rotating
at a speed of N
M
[r/min] is calculated with the following
expression:
t
DEC
= J 1
+ J
2
·
η
G
· 2
π
· (0 – N
M
)
τ
M
–
τ
L
·
η
G
60
[s] ....................... (4.16)
Where,
J
1
: Motor shaft moment of inertia [kg·m
2
]
J
2
: Load shaft moment of inertia converted to motor shaft
[kg·m
2
]
τ
M
: Minimum motor output torque in braking (deceleration)
mode [N·m]
τ
L
: Maximum load torque converted to motor shaft [N·m]
η
G
: Reduction-gear efficiency
In the above expression, generally output torque
τ
M
is
negative and load torque
τ
L
is positive. So, deceleration
time becomes shorter. However, in a lifted and lowered
load,
τ
L
may become a negative value in braking mode.
In this case, the deceleration time becomes longer.
* For lifting or lowering load
In inverter and motor capacity selection for lifted and
lowered load, the deceleration time must be calculated
by using the maximum value that makes the load torque
negative.
1.3.3 Heat energy calculation of braking resistor
Braking by an inverter causes mechanical energy to be
regenerated in the inverter circuit.
This regenerative energy is often discharged to the
resistor. In this section, braking resistor rating is explained.
Calculation of regenerative energy
Regenerative energy generated in the inverter operation
consists of kinetic energy of a moving object and its
potential energy.
q q q q q
Kinetic energy of a moving object
When an object with moment of inertia J [kg·m
2
] rotates
at a speed N
2
[r/min], its kinetic energy is as follows:
E = J · ( 2
π
· N
2
)
2
2 60
[J]
1
182.4 · J · N
2
2
[J = kWs]
The output energy when this object is decelerated to a
speed N
1
[r/min] is as follows:
E = J · [( 2
π
· N
2
)
2
– ( 2
π
· N
1
)
2
]
2 60 60 [J]
1
182.4 · J · (N
2
2
– N
1
2
) [J ]
The energy regenerated to the inverter as shown in Fig.
4.10 is calculated by considering the reduction-gear
efficiency
η
G
and motor efficiency
η
M
as follows:
E
1
182.4 · (J
1
+J
2
·
η
G
)·
η
M
· (N
2
2
–N
1
2
) [J]
w w
w w w
Potential energy of an object
When an object of W [kg] is lowered from height h
2
[m]
to h
1
[m], the output potential energy is expressed as
follows:
E = W · g · (h
2
– h
1
) [J ] .................................... (4.20)
Where, g 9.8065 [m/s
2
]
Regenerative energy to the inverter circuit is calculated
by considering the reduction-gear efficiency
η
G
and
motor efficiency
η
M
as follows:
E = W · g · (h
2
– h
1
) ·
η
G
·
η
M
[J ] ............................ (4.21)
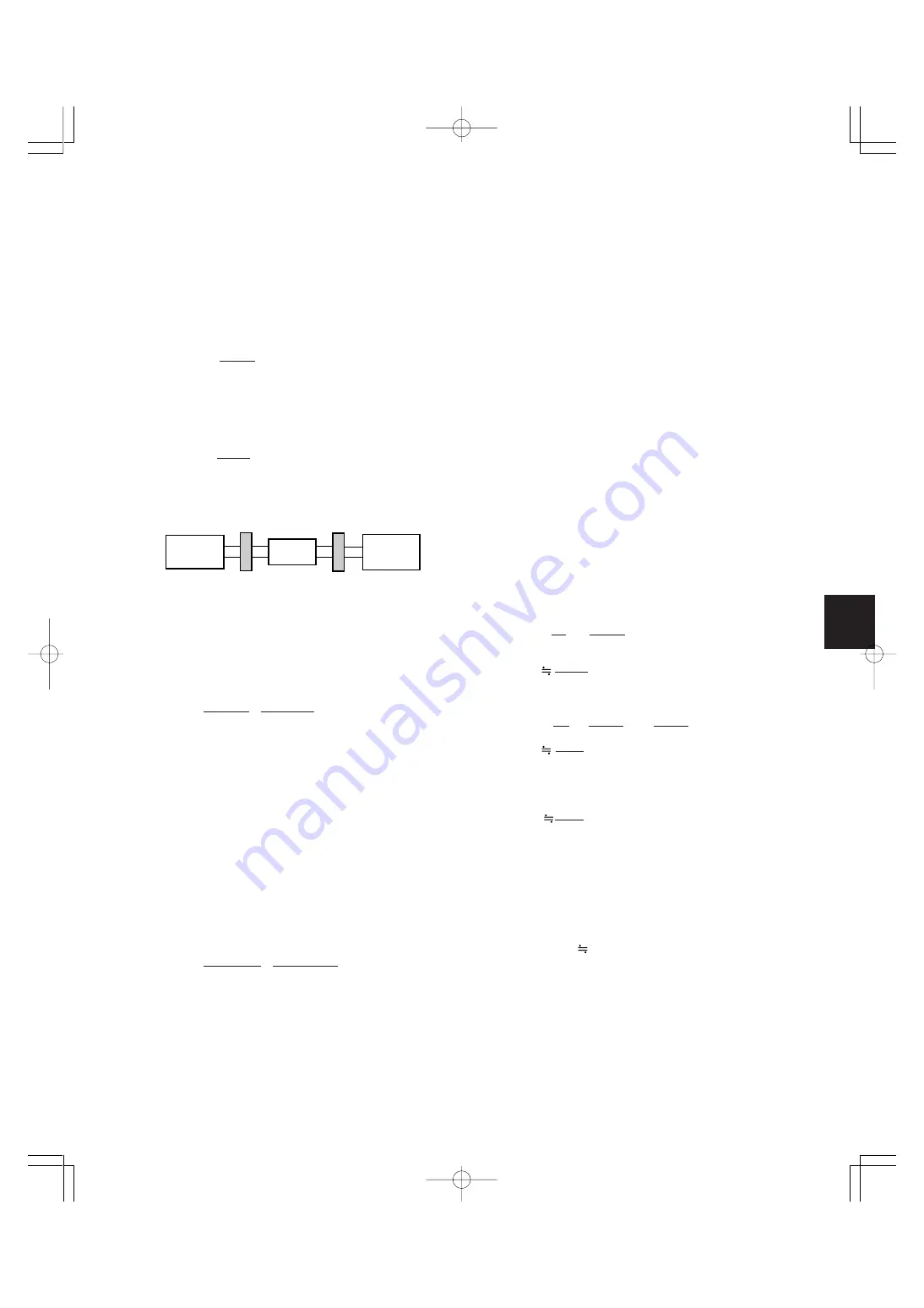
Moment of inertia
converted to motor shaft
Motor shaft moment
of inertia
Load
Reduction-
gear
Motor
J
1
[kg·m
2
] J
2
[kg·m
2
]
η G
Fig. 4.10 Load model including reduction-gear
.............. (4.18)
.............................. (4.18)’
............ (4.19)
......................... (4.17)’
..................................... (4.17)