Javad TRIUMPH-LS, User Manual

Page: 106 / 168

Coord. Sys
www.javad.com 106
Coordinate Systems
Coordinate System Basics
T
he simplest, most representative mathematical model
of the shape of the Earth, thus far conceived, is that
of an ellipsoid – similar to a sphere but slightly longer at
the equatorial axis than it is at the polar axis, the ellipsoid
is an ellipse, rotated about the minor polar axis. While
not perfectly representative of the Earth’s undulating
surface: mountainous,
oceanic, craggy, hilly, etc.,
the ellipsoid reasonably
approximates with a simple
mathematical model the
shape of the Earth.
Ellipsoid coordinates are
generally expressed as
latitude and longitude and
ellipsoid height. This type
of coordinate does not
lend itself to easy visualization on the ground nor simple
mathematical manipulation for inversing between geodetic
coordinates. Another geodetic coordinate system that
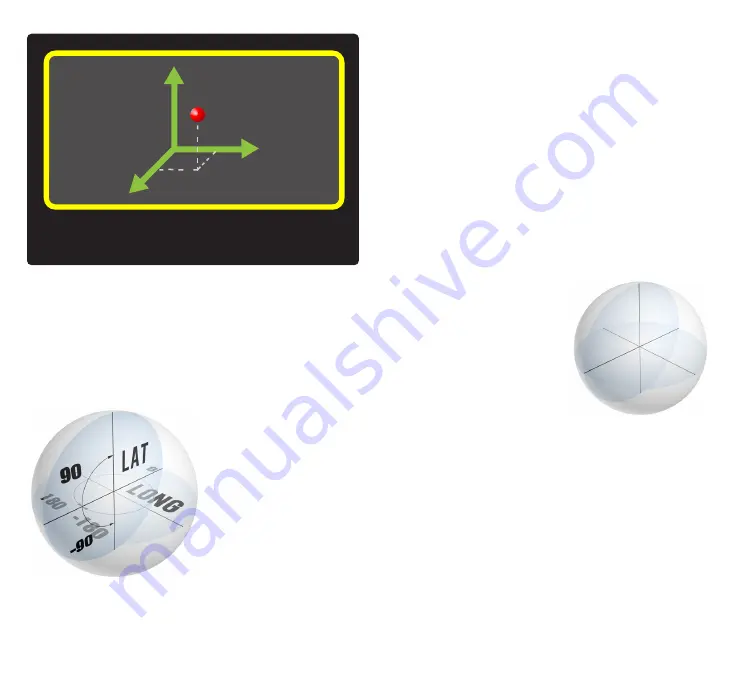
allows for simpler mathematics is Earth Centered, Earth
Fixed (ECEF). This Cartesian coordinate system has thee
axes (XYZ) extending from the origin which is located at the
Center of Mass of the Earth. The X axis is along the equatorial
plane, extending in a positive direction from the origin,
through 0° longitude. The Y axis is along the equatorial
plane, extending in a positive direction from the origin,
perpendicularly from the X axis, through 90° East longitude.
The Z axis extends in a positive direction from the origin,
through the North pole. For surveyors, challenges with this
system arise in that while the mathematics in determining
distances between points is a simple exercise of the 3D
Cartesian geometry (Pythagorean Theorem), the result
has little relevance to measurements along the surface
of the Earth where surveyors
generally work, as the results of
inverses between two points on
the surface will be chords, and
resulting directions will have
minimal apparent relationship to
North. Because of the limitations
of each of these systems for
cadastral surveying, projections,
such as the State Plane Coordinate System, have been
developed which project geodetic points on the Earth
(latitude and longitude) to a flat plane which allows for
simple planar geometry and trigonometry to be applied to
most calculations.
Most surveying software available today uses a point
database composed of a Cartesian 2D grid, Northings and
Eastings, with an optional third Height dimension allowing
for programs utilizing the same planar mathematics. The
difficulty in this approach, particularly with GNSS surveying,
is that the world we survey over, across, and under is
anything but flat, and projections, while useful tools for
Coordinate Systems