manualshive.com logo in svg

LeCroy WaveRunner 104MXI, Operator'S Manual

Share
Download
LeCroy WaveRunner 104MXI Operator'S Manual Download Page 168

W

AVE

R

UNNER 

X

I 

S

ERIES 

 

 

168

 

 

WRXi-OM-E Rev B

 

 

 

•

 

You can view the final result on the instrument display, and use all of the instrument's tools to understand the 
result.  

•

 

You can do additional processing on the result by applying either standard instrument parameters, functions, 
etc. to the returned result, or even more powerfully, adding chained customized functions. For example, you 
can do an Excel calculation on a result with a MATLAB function applied to it.  

Solutions 

Engineers do not buy equipment; they buy solutions. But what solutions can be reached from a set of instrument 
waveform data? The answer is, in principle, anything that can be logically derived from those data, given the 
limitations of signal-to-noise ratio and processing time. Here are some examples of what can be done with a 
customized instrument: 

•

 

Changing the units of a grid to joules, newtons, amps, etc.  

•

 

Creating a new waveform by manipulating the data of one or two input waveforms  

•

 

Creating a new waveform without using any of the input data  

•

 

Creating a new parameter by manipulating the data of one or two input waveforms  

•

 

Changing a vertical scale or a horizontal scale from linear to non-linear 

You don’t have to use all the data from the input waveforms: you can select data from one or more segments, 
which need not be aligned in the two-input waveforms. 

Examples 

E

XAMPLE 

1:

 

S

IMPLE MATH FUNCTIONS USING 

VBS

CRIPT

 

 

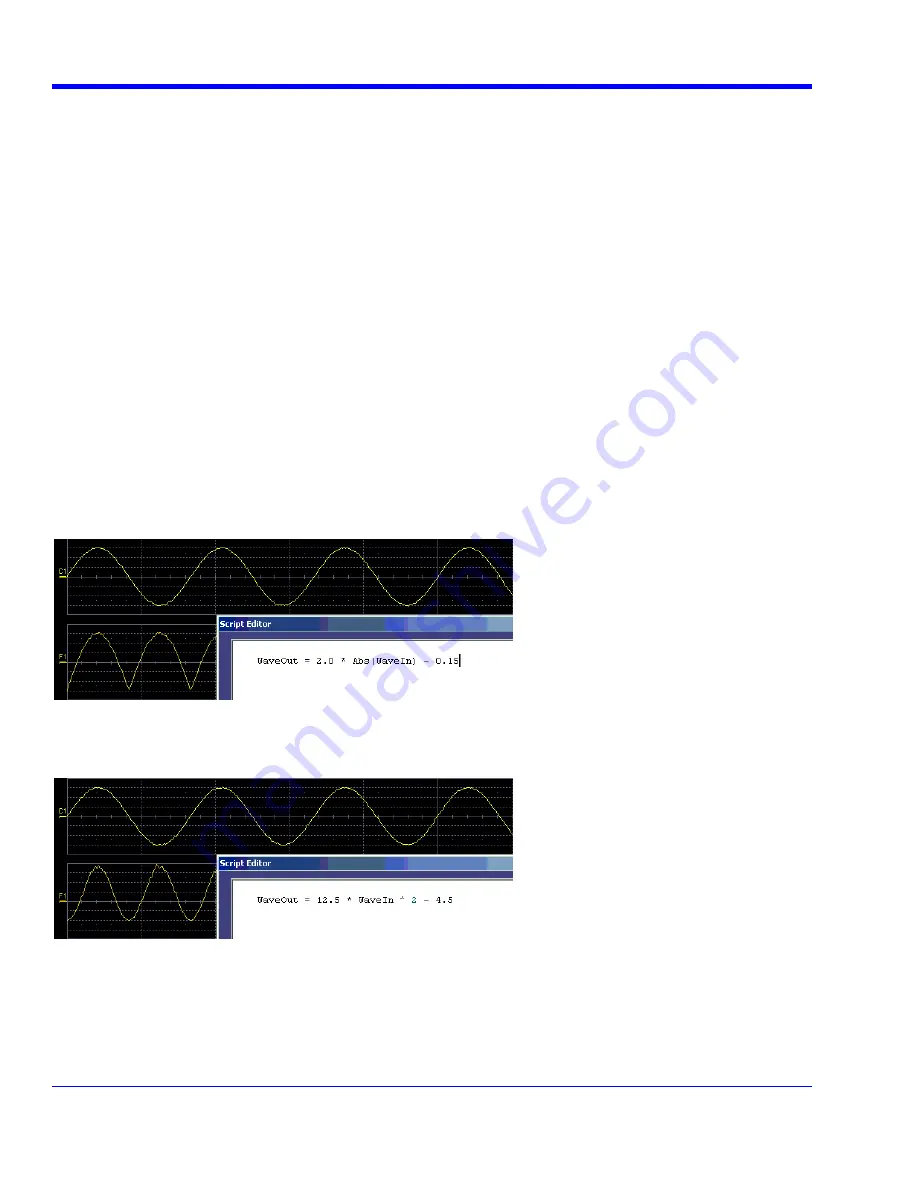
WaveOut is the waveform being returned to the instrument (F1 in this case). WaveIn is the input waveform (C1 in 
this case) You can see that the F1 result is displayed on the oscilloscope, and can be processed further. 

E

XAMPLE 

2:

 

A

NOTHER SIMPLE MATH FUNCTION USING 

VBS

CRIPT

 

 

Example 3 below doesn’t use the input data at all. The middle waveform (F2) is a "golden waveform", in this case 
a perfect sine (subject to 16-bit resolution), that was created using a VBScript. The lower trace (F3) is a 
subtraction of the acquired waveform (upper trace) and the golden waveform. The subtraction (of course) 
contains all the noise, but it also shows the presence of a very small square wave signal. 

Summary of Contents for WaveRunner 104MXI

Page 1: ...WAVERUNNER XI SERIES OSCILLOSCOPES Operator s Manual FEBRUARY 2008 ...

Page 2: ...eir respective holders Information in this publication supersedes all earlier versions Specifications subject to change without notice Manufactured under an ISO 9000 Registered Quality Management System Visit www lecroy com to view the certificate This electronic product is subject to disposal and recycling regulations that vary by country and region Many countries prohibit the disposal of waste e...

Page 3: ... Expansion Traces 18 Rapid Signal Processing 18 Internal Waveform Memory 18 Setup Storage 18 Interface 19 Auxiliary Input 19 Auxiliary Output 19 Math Tools standard 19 Measure Tools standard 20 Pass Fail Testing 20 General 20 Warranty and Service 21 Environmental Characteristics 21 Certifications 21 CE Declaration of Conformity 21 Warranty 22 Windows License Agreement 23 End User License Agreement...

Page 4: ...a New Option 42 Restoring Software 42 Restarting the Application 42 Restarting the Operating System 42 External Monitor 42 Monitor Hookup 43 Video Setup 43 Default Settings 44 Adding a New Option 44 Restoring Software 45 Restarting the Application 45 Restarting the Operating System 45 CONNECTING TO A SIGNAL 45 ProBus Interface 45 Auxiliary Output Signals 45 Auxiliary Output Setup 46 PROBES 46 Prob...

Page 5: ...Variable Gain 56 Channel Deskew 56 Channel Deskew Setup 56 TIMEBASE AND ACQUISITION SYSTEM 56 Timebase Setup and Control 56 Dual Channel Acquisition 56 Combining of Channels 56 Combining Channels 57 Autosetup 57 TRIGGERING 57 Trigger Setup Considerations 57 Trigger Modes 57 Trigger Types 57 Determining Trigger Level Slope Source and Coupling 59 Trigger Source 59 Level 60 Holdoff by Time or Events ...

Page 6: ...pe Settings 79 Saving Oscilloscope Settings 80 Recalling Oscilloscope Settings 80 Recalling Default Settings 80 Saving Screen Images 80 Saving and Recalling Waveforms 81 Saving Waveforms 81 Recalling Waveforms 82 Disk Utilities 82 Deleting a Single File 82 Deleting All Files in a Folder 83 Creating a Folder 83 PRINTING AND FILE MANAGEMENT 83 Print Plot or Copy 83 Printing 83 Printer Setup 83 Print...

Page 7: ...er Setup Dialogs 100 Status Symbols 101 Using X Stream Browser to Obtain Status Information 101 Statistics 102 Applying a Measure Mode 102 Measure Modes 102 Standard Vertical Parameters 103 Standard Horizontal Parameters 103 My Measure 103 Parameter Math WRXi XMATH or WRXi XMAP option required 103 Logarithmic Parameters 103 Parameter Script Parameter Math 104 Param Script vs P Script 104 Parameter...

Page 8: ... 134 Waveform Sparser Setup 135 Interpolation 135 Interpolation Setup 135 FFT 135 Why Use FFT 135 Power Density Spectrum 136 Memory for FFT 136 FFT Pitfalls to Avoid 136 Picket Fence and Scallop 136 Leakage 136 Choosing a Window 136 Improving Dynamic Range 137 Record Length 137 FFT Algorithms 138 FFT Glossary 139 FFT Setup 141 Setting Up an FFT 141 ANALYSIS 142 Pass Fail Testing 142 Comparing Para...

Page 9: ...e 162 E Mail 162 Aux Output 162 Setting the Date and Time 162 Manually Setting the Date and Time 163 Setting the Date and Time from the Internet 163 Setting the Date and Time from Windows 163 Options 163 Preferences 164 Audible Feedback 164 Auto calibration 164 Offset Control 164 Delay Control 164 Trigger Counter 165 Performance Optimization 165 E mail 165 Acquisition Status 166 Service 166 Show W...

Page 10: ...ample 5 186 Writing VB Scripts 186 Types of Scripts in VBS 186 Loading and Saving VBScripts 187 The default parameter function script explanatory notes 190 Scripting with VBScript 191 Variable Types 191 Variable Names 191 Arithmetic Operators 192 Results of Calculations 193 Order of Calculations 193 VBS Controls 194 IF Then Else End If 194 Summary of If Then Else 196 Select Case 196 Summary of Sel...

Page 11: ...tomDSO Setup File 217 Creating a CustomDSO Setup File 218 CustomDSO PlugIn Mode 219 Creating a CustomDSO PlugIn 219 Properties of the Control and its Objects 220 Removing a Plugin 222 First Example PlugIn Exchanging Two Traces on the Grids 222 Second Example PlugIn Log Log FFT Plot 225 Control Variables in CustomDSO 227 LABNOTEBOOK 227 Introduction to LabNotebook 227 Preferences 227 Miscellaneous ...

Page 12: ... Table of Contents Index displays an alphabetical listing of keywords Search locates every occurrence of the keyword that you enter www LeCroy com connects you to LeCroy s Web site where you can find Lab Briefs Application Notes and other useful information This feature requires that the instrument be connected to the internet through the Ethernet port on the oscilloscope s rear panel Refer to Rem...

Page 13: ...instrument s performance within specifications have us calibrate it at least once a year LeCroy offers state of the art performance by continually refining and improving the instrument s capabilities and operation We frequently update both firmware and software during service free of charge during warranty You can also install new purchased software options in your oscilloscope yourself without ha...

Page 14: ...104Xi 400 ps WaveRunner 204Xi 225 ps Bandwidth Limiters o Full o 200 MHz o 20 MHz Input Capacitance using PP008 probe 9 5 pF typical Input Capacitance of Channel 1 1 1 10 1 100 20 pF typical Input Impedance 1 Mohms 16 pF or 50 ohms WR104MXi WR104Xi WR204Xi 1 Mohms 20 pF or 50 ohms Input Coupling 50 ohms DC GND 1 Mohms AC DC GND Max Input Voltage 1 1 1 10 50 ohms 5 Vrms 1 microsecond pulse 50 duty ...

Page 15: ... 02 to 5 V div 100 mV 5 02 to 10 V div Offset Accuracy Fixed gain setting 2 V div 1 5 of offset value 0 5 of full scale value 1 mV Variable gain and settings 2 V div 1 5 of offset value 1 0 of full scale value 1 mV Probing System BNC or ProBus Horizontal System Timebases Internal timebase common to all input channels an external clock can be applied at the auxiliary input Time div Range Real time ...

Page 16: ...h 5 GS s WaveRunner 44Xi WaveRunner 64Xi WaveRunner 62Xi WaveRunner 104MXi Xi WaveRunner 204Xi All Channels 5 GS s 5 GS s 5 GS s 5 GS s 5 GS s Interleaved 5 GS s 10 GS s 10 GS s 10 GS s 10 GS s 2 Channel Max 10 GS s Maximum Acquisition Points Ch 2 Ch 4 Ch Standard 10M 20M VL Memory Option 12 5M 25M Random Interleaved Sampling RIS 200 GS s Trigger Rate 1 250 000 waveforms per second Acquisition Mod...

Page 17: ...n Width Triggers on positive or negative pulse widths selectable from 500 ps to 20 s or on intermittent faults subject to bandwidth limit of oscilloscope Pattern Logic combination AND NAND OR NOR of 5 inputs 4 channels and external trigger input 2 Ch EXT on WaveRunner 62Xi Each source can be high low or don t care The High and Low level can be selected independently Triggers at start or end of the...

Page 18: ... support to synchronize to precision internet clocks Number of Traces Maximum of eight traces simultaneously displays channel zoom memory and math traces Grid Styles Single Dual Quad Octal XY Single XY Dual XY Waveform Display Styles Sample dots joined or dots only Analog Persistence Display Analog and Color graded Persistence Variable saturation levels stores each trace s persistence data in memo...

Page 19: ...l or control signals output from rear panel BNC Calibrator Signal 250 Hz to 1 MHz square wave or DC level 50 mV to 1 0 V selectable into 1 kohms Control Signals trigger enabled trigger out pass fail status or off Math Tools standard Display up to four math function traces F1 to F4 The easy to use graphical interface simplifies setup of up to two operations on each function trace Function traces ca...

Page 20: ...vel rms std deviation time level top width width negative x minimum x maximum Pass Fail Testing Test multiple parameters against selectable parameter limits at the same time Pass or fail conditions can initiate actions including document to local or networked files email the image of the failure save waveforms send a pulse out at the front panel auxiliary BNC output or with GPIB option send a GPIB...

Page 21: ...000 m Storage non operating 12 192 m 40 000 ft RANDOM VIBRATION Operating 5 Hz to 500 Hz overall level 0 31 grms 15 minutes in each of 3 orthogonal axes Non operating 5 Hz to 500 Hz overall level 2 4 grms 15 minutes in each of 3 orthogonal axes SHOCK Functional Shock 20 g peak half sine 11 ms pulse 3 shocks positive and negative in each of 3 orthogonal axes 18 shocks total Certifications CE Compli...

Page 22: ...r intervention or system reset Low Voltage Directive EN 61010 1 2001 Safety requirements for electrical equipment for measurement control and laboratory use The oscilloscope has been qualified to the following EN 61010 1 limits Installation Categories II Mains Supply Connector I Measuring Terminals Pollution Degree 2 Normally only dry non conductive pollution occurs Occasionally a temporary conduc...

Page 23: ...TIONAL LICENSE TERMS YOU MAY NOT INSTALL COPY OR OTHERWISE USE SUCH UPDATES THE PARTIES CONFIRM THAT THIS AGREEMENT AND ALL RELATED DOCUMENTATION ARE AND WILL BE DRAFTED IN ENGLISH LES PARTIES AUX PRÉSENTÉS CONFIRMENT LEUR VOLONTÉ QUE CETTE CONVENTION DE MÊME QUE TOUS LES DOCUMENTS Y COMPRIS TOUT AVIS QUI S Y RATTACHÉ SOIENT REDIGÉS EN LANGUE ANGLAISE 1 GRANT OF LICENSE 1 1 License Grant Subject t...

Page 24: ...rade secrets in the Software Product or iii to otherwise circumvent any technological measure that controls access to the Software Product You may reverse engineer or otherwise circumvent the technological measures protecting the Software Product for the sole purpose of identifying and analyzing those elements that are necessary to achieve Interoperability the Permitted Objective only if A doing s...

Page 25: ...y the receiving party or destroy the same other than such Confidential Information as to which this EULA expressly provides a continuing right to the receiving party to retain at the time of the request Either party may only disclose the general nature but not the specific financial terms of this EULA without the prior consent of the other party provided either party may provide a copy of this EUL...

Page 26: ...ba Iran Iraq Libya North Korea Sudan and Syria or to any national of any such country wherever located who intends to transmit or transport the Restricted Components back to such country b to any end user who you know or have reason to know will utilize the Restricted Components in the design development or production of nuclear chemical or biological weapons or c to any end user who has been proh...

Page 27: ...or default of any provision of this EULA by you will be effective as to any other breach or default whether of the same or any other provision and whether occurring prior to concurrent with or subsequent to the date of such waiver If any provision of this EULA is declared by a court of competent jurisdiction to be invalid illegal or unenforceable such provision will be severed from this EULA and a...

Page 28: ...ate This symbol is used to denote Alternating Current CAUTION The CAUTION sign indicates a potential hazard It calls attention to a procedure practice or condition which if not followed could possibly cause damage to equipment If a CAUTION is indicated do not proceed until its conditions are fully understood and met WARNING The WARNING sign indicates a potential hazard It calls attention to a proc...

Page 29: ...ry conductivity caused by condensation must be expected Protection Class 1 refers to a grounded equipment in which protection against electric shock is achieved by Basic Insulation and by means of a connection to the protective ground conductor in the building wiring CAUTION Protect the DSO s display touch screen from excessive impacts with foreign objects CAUTION Do not exceed the maximum specifi...

Page 30: ...G Electric Shock Hazard Any interruption of the protective conductor inside or outside of the DSO or disconnection of the safety ground terminal creates a hazardous situation Intentional interruption is prohibited The DSO should be positioned to allow easy access to the socket outlet To completely remove power to the DSO unplug the instrument s power cord from the AC outlet after the DSO is placed...

Page 31: ...ument To avoid electrical shock unplug the power cord from the AC outlet before cleaning WARNING Electric Shock Hazard No operator serviceable parts inside Do not remove covers Refer servicing to qualified personnel Abnormal Conditions Operate the instrument only as intended by the manufacturer If you suspect the DSO s protection has been impaired disconnect the power cord and secure the instrumen...

Page 32: ...alog AUTO Triggers the oscilloscope after a time out even if the trigger conditions are not met NORMAL Triggers the oscilloscope each time a signal is present that meets the conditions set for the type of trigger selected SINGLE Arms the oscilloscope to trigger once single shot acquisition when the input signal meets the trigger conditions set for the type of trigger selected If the oscilloscope i...

Page 33: ...cal position of the selected zoom trace on the display Unlike Offset the position is not calibrated to the 0 V reference ZOOM Adjusts the vertical zoom magnification factor of the selected zoom trace on the display INDICATOR LAMPS The three lamps at the bottom of the panel are lit according to the kind of trace you are zooming or whose position you are adjusting channel trace math trace or memory ...

Page 34: ...rsor placement mode General Control Buttons AUTO SETUP Automatically sets the oscilloscope s horizontal timebase acquisition system vertical gain and offset as well as trigger conditions to display your signal TOUCH SCREEN Activates or deactivates the touch screen CLEAR SWEEPS Clears data from multiple sweeps acquisitions including persistence trace displays averaged traces parameter statistics an...

Page 35: ...s an Undo button after the Autosetup front panel button is pressed and after a zoom If you want to undo these operations you must select Undo immediately after you perform the Autosetup or zoom i e there can be no intervening operations For common oscilloscope operations you will not need to use the menu bar since you can access most dialogs from the front panel or from the descriptor labels Howev...

Page 36: ...nob is turned If you want to set delay Div to a fixed position on the grid and then have it stay fixed as the timebase changes go to Utilities Preferences Acquisition Post trigger Delay This is indicated by a left pointing arrow below left of the grid Pre trigger delay is indicated by a right pointing arrow below right of the grid Trigger Level This indicator is located at the right edge of the gr...

Page 37: ... averaging AVG These indicators have a long and short form Besides channel traces math and memory trace labels are also displayed Labels are displayed only for traces that are turned on As a visual aid an active channel i e the one whose settings can be adjusted by the front panel knobs is displayed in solid color The title bar of the TimeBase label shows the trigger delay setting Time per divisio...

Page 38: ... Calls up the Measure menu You can then select a parameter from this menu without leaving the channel setup dialog The parameter automatically appears below the grid Creates a zoom trace of the channel trace whose dialog is currently displayed Calls up the Math menu You can then select a math function from this menu without leaving the channel setup dialog A math trace of the channel whose dialog ...

Page 39: ...al position To Annotate a Waveform Touch the waveform you want to annotate then Set label in the pop up menu A dialog box opens in which to create the label If you are creating a label for the first time for this waveform Label1 is displayed with default text If you are modifying an existing label under Labels touch the label you want to change PLEASE NOTE THE FOLLOWING If the dialog for the trace...

Page 40: ... SERIES 40 WRXi OM E Rev B You may place a label anywhere you want on the waveform Labels are numbered sequentially according to the order in which they are added and not according to their placement on the waveform ...

Page 41: ...and turn on the channel To turn on a math function trace touch Math in the menu bar then Math Setup in the drop down menu Touch the On checkbox for the trace you want to activate You can also quickly create traces and turn on the trace label for math functions and memory traces without leaving the Vertical Adjust dialog by touching the icons at the bottom of the Vertical Adjust dialog Whenever you...

Page 42: ...cally If you exit the application and want to reload it touch the shortcut icon on the desktop If you minimize the application touch the desktop icon to maximize it Restarting the Operating System If you need to restart the Windows operating system you will have to reboot the oscilloscope by pressing the power switch then turning the power back on after a ten second wait External Monitor Your Wave...

Page 43: ...Notebook and the external monitor as Monitor 4 From the Primary Device drop down menu select which monitor you want to be the primary display that is which will be the one to show the oscilloscope UI If the external monitor is considerably larger than the oscilloscope s monitor you may want to make it the primary device Whichever monitor is not selected as primary will automatically appear in the ...

Page 44: ... 3 Then touch the on screen Recall Default button Adding a New Option To add a software option you need a key code to enable the option Call LeCroy Customer Support to place an order and receive the code Add the software option by doing the following 1 In the menu bar touch the button 2 In the dialog area touch the tab 3 Touch the button 4 Use the pop up keyboard to type the key code Touch O K on ...

Page 45: ...on from probe tip to oscilloscope display ProBus allows you to control transparent gain and offset directly from your front panel It is particularly useful for voltage differential and current active probes It uploads gain and offset correction factors from the ProBus EPROMs and automatically compensates to achieve fully calibrated measurements This intelligent interconnection between your instrum...

Page 46: ... LeCroy as optional accessories The PP008 is designed for use with 600 MHz and lower LeCroy WaveRunner Xi series oscilloscopes Refer to the PP008 Instruction Manual LeCroy also offers a variety of passive and active probes for use with your WaveRunner Xi Series oscilloscope Visit www lecroy com for specifications and ordering information Current Probes Current Probes measure the current passing th...

Page 47: ...OPERATOR S MANUAL WRXi OM E Rev B 47 Probes ...

Page 48: ...tion of phosphor on an analog screen WaveStream mode operates at up to 10 GS s with an update rate up to 8000 waveforms second for better capture of higher frequency abnormal events Real Time Mode A single shot real time acquisition is a series of digitized voltage values sampled on the input signal at a uniform rate Sequence Mode In sequence mode the complete waveform consists of a number of fixe...

Page 49: ...0 pre trigger and it allows you to see the waveform leading up to the point at which the trigger condition was met and the trigger occurred The instrument offers up to the maximum record length of points of pre trigger information Post trigger delay on the other hand allows you to sample the waveform starting at the equivalent of 10 000 divisions after the event occurred Because each instrument in...

Page 50: ...n menu 2 Touch the Sequence mode button 3 Touch the Sequence tab Under Sequence Options touch inside the Num Segments field and enter the number of segments you want to display using the pop up keypad 4 Touch inside the Timeout field and enter a timeout value Note The timeout period accounts for instances when a Num Segments miscount occurs for some reason and the oscilloscope waits indefinitely f...

Page 51: ...NT WATERFALL CASCADED MOSAIC TILED OVERLAY PERSPECTIVE The number of segments you choose to display 80 maximum can be less than the total number of segments in the waveform For example in the pop up images above the number of display segments is 10 but the total number of segments entered in the timebase dialog s Num Segments field is 100 ...

Page 52: ...higher than the maximum single shot sampling rate It is used on repetitive waveforms with a stable trigger The maximum effective sampling rate of 50 GS s can be achieved with RIS by making 100 single shot acquisitions at 500 MS s The bins thus acquired are positioned approximately 20 ps apart The process of acquiring these bins and satisfying the time constraint is a random one The relative time b...

Page 53: ... the roll mode buffer is updated as if new data is available This resets statistics on every step of Roll mode that is valid because of new data Note If the processing time is greater than the acquire time the data in memory gets overwritten In this case the instrument issues the warning Channel data is not continuous in ROLL mode and rolling will start over again VERTICAL SETTINGS AND CHANNEL CON...

Page 54: ...imit the coupling mode automatically switches from DC 50 to GROUND You will then have to manually reset the coupling to DC 50 ohms as described next Coupling Setup 1 In the menu bar touch the Vertical button then Channel X Setup in the drop down menu 2 Touch inside the Coupling field and select a coupling mode from the pop up menu Probe Attenuation Probe Attenuation Setup LeCroy s ProBus system au...

Page 55: ... Sinx x interpolation on the other hand is suitable for reconstructing curved or irregular waveshapes especially when the sample rate is 3 to 5 times the system bandwidth Interpolation Setup 1 Touch the button for the channel you want to set up for example 2 In the dialog area touch inside the Interpolation field under Pre Processing Pre Processing means before Math processing 3 Touch inside the I...

Page 56: ... timebase setups 1 Touch Timebase in the menu bar then Horizontal Setup in the drop down menu The Horizontal dialog appears 2 Touch inside the Time Division field and enter a value using the pop up numeric keypad or use the up down arrows to adjust the value 3 Touch inside the Delay field and type in a value using the pop up keypad Touch the Set To Zero button to set the delay to zero 4 Under Real...

Page 57: ...ou select one of the other three modes Trigger Types The triggers available to you are defined as follows Edge A simple trigger Edge trigger is activated by basic waveform features or conditions such as positive or negative slope and holdoff Width Width trigger allows you to define a positive or negative going pulse width bounded by a voltage level above or below which a trigger will occur Or you ...

Page 58: ...voltage logic level for each input independently TV TV triggers provide stable triggering on standard or custom composite video signals Use them on PAL SECAM or NTSC systems Optional HDTV trigger supports 1080i 1080p and 720p formats Serial Trigger and Decode Serial trigger allows a serial trigger condition to be set from within the oscilloscope using an easy to understand interface Slew Rate Slew...

Page 59: ...s you may switch the channel to ground coupling at any time while testing With DC coupling all of the signal s frequency components are coupled to the trigger circuit for high frequency bursts 3 Slope determines the direction of the trigger voltage transition used for generating a particular trigger event You can choose a positive negative or windowed slope Like coupling the selected slope is asso...

Page 60: ...casions on which the trigger condition is met The trigger will again occur when the holdoff has elapsed and the trigger s other conditions are met Use holdoff to obtain a stable trigger for repetitive composite waveforms For example if the number or duration of sub signals is known you can disable them by choosing an appropriate holdoff value Qualified triggers operate using conditions similar to ...

Page 61: ... into two major categories 1 Edge activated by basic waveform features or conditions such as a positive or negative slope and hold off 2 SMART Trigger sophisticated triggers that enable you to use basic or complex conditions for triggering Use Edge Triggers for simple signals and the SMART Triggers for signals with rare features like glitches Control Edge Triggering Horizontal Turn the Delay knob ...

Page 62: ... select Trigger Setup from the drop down menu 2 Touch the Edge trigger button under the Trigger tab 3 Touch inside the Trigger Source field and select an input from the pop up menu 4 Touch inside the Level field 5 In the pop up numeric keypad enter a value in millivolts or use the up down buttons to increase or decrease the value in increments of 1 mV Or touch one of the preset value buttons 6 Sel...

Page 63: ...rigger Source field and select a source on which to trigger 4 Touch inside the Level field and enter a value using the pop up numeric keypad 5 Select Positive or Negative polarity 6 Touch the Less Than button and enter a pulse width value in the Upper Limit field o Or touch the Greater Than button and enter a pulse width value in the Lower Limit field o Or touch the InRange button Touch the Delta ...

Page 64: ...s present a trigger can occur The timing for the delay is restarted when the selected pattern begins Events determines a minimum number of events of the trigger source An event is generated when a trigger source meets its trigger conditions On the selected event of the trigger source and while the pattern is present a trigger can occur The count is initialized and started whenever the selected pat...

Page 65: ... CH 1 CH 2 CH 3 CH 4 and EXT The combination of inputs is referred to as a pattern There are four logic gates available AND NAND OR NOR A trigger state is either high or low high when a trigger source is greater than the trigger level threshold and low when less than it For example an AND pattern could be defined as true when the trigger state for CH 1 is high CH 2 is low and EXT is irrelevant X o...

Page 66: ...e pop up menu Note The channel that you have the VT75 Module connected to is automatically designated TV Signal in the menu 5 Touch inside Polarity and select positive or negative polarity This selection is independent of the polarity selected for Edge trigger positive in the Trigger setup dialog 6 Check the Trigger on Any Line checkbox if you do not want to specify one In this case the oscillosco...

Page 67: ...t a maximum pulse width This glitch trigger is generated on the selected edge positive or negative when the pulse width is less than or equal to the set width The timing for the width is initialized and restarted on the opposite slope to that selected Note If the glitch s width is narrower than the signal s width set the trigger to a narrower width than that of the signal The signal s width as det...

Page 68: ...negative to negative Use Interval trigger to capture intervals that fall short of or exceed a given time limit In addition you can define a width range to capture any interval that is itself inside or outside the specified range an exclusion trigger by interval Interval Less Than For this Interval Trigger generated on a time interval smaller than the one that you set choose a maximum interval betw...

Page 69: ...terval Trigger is generated whenever an interval between two edges of the same slope falls within a selected range The instrument initializes and restarts the timing for the interval whenever the selected edge occurs Interval Trigger that triggers when the interval falls within the selected range t1 range s lower time limit t2 range s upper limit The broken upward pointing arrow indicates a potent...

Page 70: ...ues using the pop up numeric keypads Touch the Limits button to set up a precise range Touch inside the Lower Limit and Upper Limit data entry fields and enter values using the pop up numeric keypads o Or if available touch the Out Of Range button and perform the same Delta or Limits setup as for In Range triggering Dropout Trigger Used primarily in single shot applications and usually with a pre ...

Page 71: ...lse width range by setting Lower Limit and Upper Limit values using the pop up keypads o Or touch the Out Of Range button and perform the same range setups as for In Range triggering Slew Rate Trigger 1 Touch the Trigger descriptor label 2 If applicable touch the Smart trigger button then touch the Slew Rate trigger button 3 Touch inside the trigger Source field and select a source on which to tri...

Page 72: ...Persistence On checkbox 3 Select a display mode by touching one of the mode buttons 4 Touch inside the Num seg displayed field and enter a value using the pop up keypad The maximum number of segments that can be displayed is 100 5 Touch inside the Starting at field and enter a value Note The maximum value that you can enter for Starting at depends on the Num Segments value you entered in the Timeb...

Page 73: ...turation level as a percentage of the maximum population All populations above the saturation population are then assigned the highest color intensity that is they are saturated At the same time all populations below the saturation level are assigned the remaining intensities Data populations are dynamically updated as data from new acquisitions is accumulated Color mode persistence selected by to...

Page 74: ...s has been rotated 60 the Y axis has been rotated 15 Here is a monochrome analog view of the same waveform The lightest areas indicate highest intensity corresponding to the red areas in the solid view Here is a shaded projected light view of the same waveform This view emphasizes the shape of the pulses Here is a wire frame view of the same waveform in which lines of equal intensity are used to c...

Page 75: ...nside the Persistence Time field and make a selection from the pop up menu 7 If you selected Per Trace for each input channel touch its tab then make selections of mode saturation level persistence time and last trace display in the same way as for All Locked To create a 3 dimensional view touch the 3d button Then 1 Touch inside the Saturation field and enter a whole number integer using the pop u...

Page 76: ...traces a zoom control mini dialog is provided at the right of each math trace Fx setup dialog for precise control Alternatively you can touch and drag a rectangle around a portion of the math or memory trace to zoom it which can be done consecutively for higher and higher zooms Note If a histogram is applied the range of the histogram is limited to the portion of the trace that is visible on scree...

Page 77: ...er div value using the pop up numeric keypad 9 Then touch inside the Vertical Scale div field and enter a voltage value Reset the zoom to x1 magnification touch Reset Zoom in the dialog or press the front panel zoom button Zooming by Touch and Drag 1 Touch and drag a rectangle around any part of an input channel waveform math trace or memory trace If you have enclosed a combination of channel and ...

Page 78: ...elete the check mark and disable the zoom trace Multi Zoom The Multi zoom feature creates time locked zoom traces for only the waveforms that you choose to include The zooms are of the same X axis section of each waveform Thus as you scroll through a waveform all included zooms scroll in unison MULTI ZOOM SETUP 1 In the menu bar touch Math then Math Setup in the drop down menu 2 Verify that the ma...

Page 79: ...resulting pattern reveals information about phase difference and frequency ratio XY DISPLAY SETUP 1 In the menu bar touch Display then touch Display Setup in the drop down menu 2 Choose an XY display by touching one of the XY display mode buttons 3 You have the choice of showing the two waveforms on just the XY grid or you can also show the input waveforms on a single or dual grid 4 Touch inside t...

Page 80: ...Then touch To recall settings from folder D Internal Setups on the oscilloscope s hard drive touch alongside the file you want to recall Recalling Default Settings 1 In the menu bar touch File then touch Recall Setup in the drop down menu 2 Touch the button under Recall Default Setup Saving Screen Images You can send images to a hard copy printer or to storage media Both types of output are done f...

Page 81: ...ause by design the first waveform is automatically numbered 0 the second 1 etc For example if you want to use waveform name XYZ32 but it is not preceded by waveforms XYZ0 through XYZ31 the waveform will be renumbered with the next available number in the sequence If you need to use a number in your waveform s name it is recommended that you append an alpha character at the end of the number XYZ32a...

Page 82: ... memory 6 Touch inside the Recall files from directory field and enter the path using the pop up keyboard Or touch the Browse button to navigate to the file 7 Touch inside the Next file will be recalled from field and enter the path using the pop up keyboard Or touch the Browse button to navigate to the file 8 Touch Disk Utilities Use the Disk Utilities dialog to delete files or create folders Del...

Page 83: ...utput files to a printer or plotter to print to file or to e mail your files Any Windows 2000 supported printer is supported by your instrument Printing Printer Setup 1 In the menu bar touch File then Print Setup in the drop down menu The Utilities Hardcopy dialog opens 2 In the dialog area touch the Printer icon 3 Under Colors touch the Use Print Colors checkbox if you want the traces printed on ...

Page 84: ...ging Files Use the instrument s utilities to create waveform files on USB memory internal hard drive or network drives You can copy files from your hard drive to floppy disk You also can give your files custom names and create directories for them Hard Disk Partitions The instrument s hard disk is partitioned into drive C and drive D Drive C contains the Windows operating system and the instrument...

Page 85: ...e packs from Microsoft please be advised that LeCroy cannot guarantee trouble free operation afterwards Avoid modifying Control Panel settings Do not change the color resolution 24 bit or screen size 800 x 600 pixel settings After you load third party software applications if your oscilloscope does not work properly try reloading the instrument software from the CD shipped with the oscilloscope Do...

Page 86: ...Measure Setup in the drop down menu 2 Touch one of parameter tabs P1 through Px 3 Touch inside the Source1 field and select an input waveform from the pop up menu 4 Touch inside the Measure field and select a parameter from the pop up menu 5 Touch the Trend button at the bottom of the dialog then from the Math selection for Trend menu select a math function location F1 to Fx The number of math tra...

Page 87: ... newly displayed Track math function trace label if you want to change any settings in the Track dialog HISTOGRAMS Creating and Viewing a Histogram Note The number of sweeps comprising the histogram will be displayed in the bottom line of the trace descriptor label Single Parameter Histogram Setup FROM MEASURE DIALOG 1 In the menu bar touch Measure then Measure Setup 2 Touch the My Measure button ...

Page 88: ... Width button to center the histogram Or touch inside the Center then the Width fields and enter a value using the pop up numeric keypad 11 Touch inside the Vertical Scale field and select Linear or Linear Constant Max from the pop up menu Viewing Thumbnail Histograms Histicons are miniature histograms of parameter measurements that appear below the grid These thumbnail histograms let you see at a...

Page 89: ...uch inside the Slice Direction field and select Horizontal or Vertical slice from the pop up menu 6 Touch inside the Slice Center field and enter a value using the pop up keypad 7 Touch inside the Slice Width field and enter a value using the pop up keypad Note You can use the front panel Adjust knobs to move the Slice Center line and the Slice Width boundary lines Persistence Trace Range This mat...

Page 90: ...ts of these lines with the 50 height value is then determined The length of a line connecting the intersection points is the value for fwhm Example fwxx Full Width at xx Maximum Definition Determines the width of the largest area peak measured between bins on either side of the highest bin in the peak that have a population of xx of the highest s population If several peaks have an area equal to t...

Page 91: ...se see hbase While that of the rightmost is the histogram top see htop The parameter is then calculated as hampl htop hbase Example In this histogram hampl is 152 mV 150 mV 2 mV hbase Histogram Base Definition The value of the leftmost of the two most populated peaks in a histogram This parameter is primarily useful for waveforms with two primary parameter values such as TTL voltages where hbase w...

Page 92: ...ighest population bin in a histogram Description Each bin between the parameter cursors is examined for its population count The leftmost bin with the highest count found is selected Its center value is returned as mode Example Here mode is 150 mV hist range Histogram Range Definition Computes the difference between the value of the rightmost and that of the leftmost populated bin Description The ...

Page 93: ...ple Using the histogram shown here the value for hrms is hrms 2 87 hist top Histogram Top Definition The value of the rightmost of the two most populated peaks in a histogram This parameter is useful for waveforms with two primary parameter values such as TTL voltages where htop would indicate the binary 1 voltage value Description The two highest histogram peaks are determined The rightmost of th...

Page 94: ...peaks in a histogram Description The instrument analyzes histogram data to identify peaks from background noise and histogram binning artifacts such as small gaps Peak identification is a 3 step process 1 The mean height of the histogram is calculated for all populated bins A threshold T1 is calculated from this mean where T1 mean 2 sqrt mean 2 A second threshold is determined based on all populat...

Page 95: ... Definition Returns the value of the xxth peak that is the largest by area in a histogram Description First the peaks in a histogram are determined and ranked in order of total area for a discussion on how peaks are identified see the description for the pks parameter The center of the nth ranked peak the point where the area to the left is equal to the area to the right where n is selected by you...

Page 96: ... between 1 6 V and 1 7 V and so on After a process of several thousand events the bar graph of the count for each bin its histogram provides a good understanding of the distribution of values Histograms generally use the x axis to show a bin s sub range value and the Y axis for the count of parameter values within each bin The leftmost bin with a non zero count shows the lowest parameter value mea...

Page 97: ...as a powerful range finding function If required it will examine the values in the parameter buffer to calculate an optimal range and redisplay the histogram using it The instrument will also give a running count of the number of parameter values that fall within below or above the range If any values fall below or above the range the range finder can then recalculate to include these parameter va...

Page 98: ...ost of two largest peaks max populate population of most populated bin in histogram mode data value of most populated bin in histogram percentile data value in histogram for which specified x of population is smaller peaks number of peaks in histogram pop x population of bin for specified horizontal coordinate range difference between highest and lowest data values total pop total population in hi...

Page 99: ... bins to be used An optimal bin number is one where the change in parameter values is insignificant and the histogram distribution does not have a jagged appearance With this buffer a histogram can be dynamically redisplayed as the number of bins is modified by the user In addition depending on the number of bins selected the change in waveform parameter values can be seen WAVEFORM MEASUREMENTS Me...

Page 100: ...also use the Cursors knobs on the front panel to place the cursors If you chose an Absolute mode do the same for your single cursor 6 If you chose a Relative mode and you would like both cursors to move in unison as you adjust the position touch the Track check box to enable tracking Overview of Parameters Parameters are measurement tools that determine a wide range of waveform properties Use them...

Page 101: ...rrow indicates an underflow condition An upward pointing arrow indicates an overflow condition An upward and downward pointing arrow indicates an underflow and overflow condition Using X Stream Browser to Obtain Status Information Example Here is a case of an overflow condition in which the amplitude of the waveform cannot be determined 1 Minimize the oscilloscope display by selecting File Æ Minim...

Page 102: ... waveform like edge level mean minimum maximum etc the value displayed represents the number of sweeps For any parameter that computes on every event the value displayed is equal to the number of events per acquired waveform If x waveforms were acquired the value represents x times the number of cycles per waveform Also the value is equal to the measurement of the last cycle on the last acquisitio...

Page 103: ...WRXi XMAP option required The instrument gives you the ability to perform arithmetic operations addition subtraction multiplication division on the results of two parameter measurements Alternatively you can apply math to a single parameter for example invert By customizing parameters in this way you can effectively extend the range of parameter measurements based on your particular needs Logarith...

Page 104: ...ur needs Code entry is done in the Script Editor window directly on the instrument You can also import an existing script Param Script vs P Script Param Script is a VBScript or JavaScript that operates on one or two waveforms and outputs a parameter measurement as shown in the following figure P Script on the other hand is another VBScript or JavaScript that takes as input one or two parameters an...

Page 105: ...that requires two input parameters the Source field will expand to two fields Parameter Script Math Setup 1 Touch Measure in the menu bar then Measure Setup in the drop down menu 2 Touch the My Measure button in the Measure dialog 3 Touch the Px tab for the parameter to which you want to apply parameter math 4 In the Px dialog touch the math on parameters button The Source field will expand to two...

Page 106: ...gate posts in their default position at each end of the grid But if you touch either end of the grid a drag cursor will appear indicating that you have control of the post and can now drag it In this example you can see that the Measure Gate includes only five rising edges Therefore parameter calculations for rise time are performed only on the five pulses bounded by the gate posts The position of...

Page 107: ...waveform intersects the chosen level This feature also displays the hysteresis band that you have set about that level You also have the option by means of an Always On checkbox to leave the Help Markers displayed after you have closed the Help Markers setup dialog You have a choice of Simple or Detailed views of the markers The Simple selection produces cursors and Measure Gate gate posts The gat...

Page 108: ...ff Help Markers for individual parameters touch the Px tab for the parameter in question Then uncheck the Always On checkbox When you close this dialog the Help Markers for this parameter will no longer be displayed Customizing a Parameter From the Measure Dialog 1 Touch the My Measure button in the Measure dialog The dialog presents you with a panel of eight preset parameters 2 For each parameter...

Page 109: ...eter or the last one if all are used 3 Select another parameter or touch Close Parameter Calculations Parameters and How They Work DETERMINING TOP AND BASE LINES Proper determination of the top and base reference lines is fundamental for ensuring correct parameter calculations The analysis begins by computing a histogram of the waveform data over the time interval spanned by the left and right tim...

Page 110: ...d to yield the rise or fall time These results are averaged over the number of transition edges that occur within the observation window Rising Edge Duration Falling Edge Duration Where Mr is the number of leading edges found Mf the number of trailing edges found the time when rising edge i crosses the x level and the time when falling edge i crosses the x level Determining Time Parameters Time pa...

Page 111: ...he level and type percent or absolute List of Parameters The following table describes the instrument parameters Availability of some parameters depends on the options installed See the comments in the Notes column of the table Parameter Description Definition Notes Amplitude Measures the difference between upper and lower levels in two level signals Differs from pkpk in that noise overshoot under...

Page 112: ...rvals Data value for which 50 of values are above and 50 below Choose this parameter by selecting Median from the parameter table then touching the Cyclic checkbox Standard parameter cyclic RMS Cyclic root mean square Computes square root of sum of squares of data values divided by number of points Contrary to rms calculation is performed over an integral number of cycles eliminating bias caused b...

Page 113: ...nate levels from noise in data Standard parameter Duration For single sweep waveforms dur is 0 for sequence waveforms time from first to last segment s trigger for single segments of sequence waveforms time from previous segment s to current segment s trigger for waveforms produced by a history function time from first to last accumulated waveform s trigger Time from first to last acquisition for ...

Page 114: ...m giving however less predictable results Standard parameter Fall 80 20 Fall 80 20 Duration of pulse waveform s falling transition from 80 to 20 averaged for all falling transitions between the cursors Average duration of falling 80 20 transition On signals not having two major levels triangle or saw tooth waves for example top and base can default to maximum and minimum giving however less predic...

Page 115: ...P options Standard in DDA 5005A FWxx Measures the width of the largest area histogram peak at xx of the population of the highest peak Available with DDM2 JTA2 and XMAP options Standard in DDA 5005A Half period Half period of a waveform Reference levels and edge transition polarity can be selected Hysteresis argument used to discriminate levels from noise in data Available with JTA2 and XMAP optio...

Page 116: ...ou can set levels slope and hysteresis independently for Hold Clock and Hold Data See also Setup parameter Reference levels and edge transition polarity can be selected Hysteresis argument used to discriminate levels from noise in data Available with JTA2 and XMAP options Last Time from trigger to last rightmost cursor Time from trigger to last cursor Indicates location of right cursor Cursors are...

Page 117: ...m noise in data Available with DDM2 option Standard in DDA 5005A Local pkpk Vertical difference between the peak and trough of a local feature lmax lmin Hysteresis argument used to discriminate levels from noise in data Available with DDM2 option Standard in DDA 5005A Local tbe Time between events between local peak and next trough or local trough and next peak Hysteresis argument used to discrimi...

Page 118: ...al feature peak and trough Hysteresis argument used to discriminate levels from noise in data Available with DDM2 option Standard in DDA 5005A Local ttp Time between local feature trough and the next local peak Hysteresis argument used to discriminate levels from noise in data Available with DDM2 option Standard in DDA 5005A Local tut Time a local feature spends under a user specified percentage o...

Page 119: ...main waveform or histogram of data of same waveform But with histograms result may include contributions from more than one acquisition Standard parameter Median The average of base and top values Average of Base and Top Standard parameter Minimum Measures the lowest point in a waveform Unlike base does not assume waveform has two levels Lowest value in waveform between cursors Gives similar resul...

Page 120: ...contain at least one rising edge On signals not having two major levels triangle or saw tooth waves for example may not give predictable results Standard parameter Overwrite Ratio of residual to original power of a low frequency waveform overwritten by a higher frequency Available with DDM2 option Standard in DDA 5005A Param Script Visual Basic or Java script that produces a measurement from one o...

Page 121: ...ou can set the output type to percent degrees or radians After setting up the reference touch the More tab for signal setups Phase difference between signal and reference Standard parameter Pop X Population at bin for specified horizontal coordinate You can place the cursor at any bin and use either Absolute Reference or Difference cursor shape Available with DDM2 JTA2 and XMAP options Standard in...

Page 122: ... On signals not having two major levels triangle or saw tooth waves for example top and base can default to maximum and minimum giving however less predictable results Standard parameter Rise 20 80 Rise 20 to 80 Duration of pulse waveform s rising transition from 20 to 80 averaged for all rising transitions between the cursors Average duration of rising 20 80 transition On signals not having two m...

Page 123: ...ata Available with JTA2 and XMAP options Skew Time of clock1 edge minus time of nearest clock2 edge Reference levels and edge transition polarity can be selected Hysteresis argument used to discriminate levels from noise in data Hysteresis on a measurement if set to 500 mdiv requires that the signal must transition one way 1 2 division total swing across the threshold for the measurement to be val...

Page 124: ...ing a given slope and level and the ideal expected time For Slope you can choose positive negative or both For output units you can choose time or unit interval UI A unit interval equals one clock period The Virtual Clock setup gives you a choice of Standard 1 544 MHz or Custom reference clocks You can also use a mathematically derived Golden PLL to filter low frequency jitter The cutoff frequency...

Page 125: ...is considered to consist of positive pulses and width the time between adjacent rising and falling edges Width of first positive or pulse averaged for all similar pulses Similar to fwhm though unlike width that parameter applies only to histograms Standard parameter Width level Width measured at a specific level Reference levels and edge transition polarity can be selected Hysteresis argument used...

Page 126: ...For example you could set up F1 as the difference between Channels 1 and 2 F2 as the average of F1 and F3 as the integral of F2 You could then display the integral of the averaged difference between Channels 1 and 2 Any trace and function can be chained to another trace and function For example you could make F1 an average of Channel 1 F2 an FFT of F1 and F3 a zoom of F2 Refer to the Specification...

Page 127: ...ope See Specifications 3 Touch the single function button 4 Touch inside the Source1 field and select a source channel math trace memory location 5 Touch inside the Operator1 field and select Deskew from the Functions category 6 In the dialog on the right touch the Deskew tab 7 Touch inside the Delay by field and type in a time value using the pop up keypad Rescaling and Assigning Units This featu...

Page 128: ... Meter FT Foot IN Inch YARD yard MILE mile N Newton OHM Ohm PAL Pascal PCT Percent POISE Poise PPM parts per million RAD Radian DEG Degree of arc MNT Minute of arc SAMPLE sample SWEEP sweeps SEC Second of arc S Second SIE Siemens T Tesla UI Unit interval V Volt VA Volt amps W Watt WB Weber MIN min HOUR Hour DAY Day WEEK Week ...

Page 129: ... Operator1 field and select Rescale from the Functions category 6 In the dialog on the right touch the Rescale tab 7 Touch inside the First multiply by checkbox and enter a value for a the multiplication factor 8 Touch inside the then add field and enter a value for b the additive constant 9 Touch inside the Override units checkbox to disregard the source waveform s units using the pop up keyboard...

Page 130: ...seful for reducing noise on signals that drift very slowly in time or amplitude The most recently acquired waveform has more weight than all the previously acquired ones the continuous average is dominated by the statistical fluctuations of the most recently acquired waveform The weight of old waveforms in the continuous average gradually tends to zero following an exponential rule at a rate that ...

Page 131: ...ng you to distinguish closely spaced voltage levels The functioning of the instrument s ERES is similar to smoothing the signal with a simple moving average filter However it is more efficient concerning bandwidth and pass band filtering Use ERES on single shot waveforms or where the data record is slowly repetitive when you cannot use averaging Use it to reduce noise when your signal is noticeabl...

Page 132: ... filters have a precisely constant zero phase response This has two benefits First the filters do not distort the relative position of different events in the waveform even if the events frequency content is different Second because the waveforms are stored the delay normally associated with filtering between the input and output waveforms can be exactly compensated during the computation of the f...

Page 133: ...a 2 bit enhanced resolution filter The result left bottom shows a smooth trace where most of the noise has been eliminated Note Enhanced resolution can only improve the resolution of a trace it cannot improve the accuracy or linearity of the original quantization The pass band will cause signal attenuation for signals near the cut off frequency The highest frequencies passed may be slightly attenu...

Page 134: ...at is no calculations need to be undone on the copy before additional math can be calculated This benefit of faster throughput however comes at the expense of memory usage Waveform Sparser The Sparse math function allows you to thin out an incoming waveform by skipping points at regular intervals and by starting acquisition at a particular offset point The Sparsing factor specifies the number of s...

Page 135: ...ch inside the Algorithm field and select an interpolation type 8 Touch inside the Upsample by Upsampling is the factor by which sampling is increased field and enter a value using the pop up numeric keypad if you want to enter a specific value Otherwise use the Up Down buttons to increment the displayed value in a 1 2 5 sequence FFT Why Use FFT For a large class of signals you can gain greater ins...

Page 136: ...components that are not exact multiples of that frequency will fall within two consecutive filters This results in an attenuation of the true amplitude of these components Picket Fence and Scallop The highest point in the spectrum can be 3 92 dB lower when the source frequency is halfway between two discrete frequencies This variation in spectrum magnitude is the picket fence effect The correspond...

Page 137: ...reduce leakage and improve amplitude accuracy However frequency resolution is also reduced Flat Top This window provides excellent amplitude accuracy with moderate reduction of leakage but with reduced frequency resolution Blackman Harris It reduces the leakage to a minimum but with reduced frequency resolution FFT Window Filter Parameters Window Type Highest Side Lobe dB Scallop Loss dB ENBW bins...

Page 138: ...ectrum peaks The real part of Xn is symmetric around the Nyquist frequency that is Rn RN n while the imaginary part is asymmetric that is In IN n The energy of the signal at a frequency n is distributed equally between the first and the second halves of the spectrum the energy at frequency 0 is completely contained in the 0 term The first half of the spectrum Re Im from 0 to the Nyquist frequency ...

Page 139: ...lected so that the resulting Nyquist frequency is higher than the highest significant component in the time domain record Coherent Gain The normalized coherent gain of a filter corresponding to each window function is 1 0 0 dB for a rectangular window and less than 1 0 for other windows It defines the loss of signal energy due to the multiplication by the window function This loss is compensated f...

Page 140: ...the bin frequencies the spectrum contains a sharp component whose value accurately reflects the source waveform s amplitude For intermediate input frequencies this spectral component has a lower and broader peak The broadening of the base of the peak stretching out into many neighboring bins is termed leakage It is due to the relatively high side lobes of the filter associated with each frequency ...

Page 141: ...waveform and k is the time index The table of Coefficients of Window Functions lists the coefficients am The window functions seen in the time domain are symmetric around the point k N 2 Coefficients of Window Functions Window Type a0 a1 a2 Rectangular 1 0 0 0 0 0 von Hann 0 5 0 5 0 0 Hamming 0 54 0 46 0 0 Flat Top 0 281 0 521 0 198 Blackman Harris 0 423 0 497 0 079 FFT Setup Setting Up an FFT 1 I...

Page 142: ...h operation that shortens the record This is commonly encountered in filtering operations like enhanced resolution The missing data points are replaced by data values whose amplitudes are interpolated to fit between the last data point and the first data point in the record This guarantees that there is not a first order discontinuity in the filled data Since the data at the end of the record is f...

Page 143: ...the mask Masks that you create from your waveform can be confined to just a portion of the trace by use of a measure gate See Measure Gate for an explanation of how this feature works Actions By touching the Stop Test checkbox in the Actions dialog you can set up the test to end after a predetermined number of sweeps that you decide You can also decide the actions to occur upon your waveforms pass...

Page 144: ...result emit pulse or save the waveform If you want to have the results printed and your oscilloscope is not equipped with a printer be sure that the it is connected to a local or network printer See Printing If you want to save your waveform automatically touch the Save Setup This will take you out of the current dialog and will open the Save Waveform dialog See Saving and Recalling Waveforms Test...

Page 145: ... at the bottom of the ParamCompare dialog Comparing Dual Parameters 1 Touch Analysis in the menu bar then Pass Fail Setup in the drop down menu 2 Touch a Qx tab a setup dialog for that position will open 3 Touch inside the Condition field in the main dialog and select DualParamCompare 4 Touch inside the Source1 and Source2 fields and select a source from the pop up menu 5 Touch inside the ParamCom...

Page 146: ...g and select Mask Test 5 From the Test mini make a selection in the Test is True when group of buttons This selection means for example that if you select All In the test will be False if even a single waveform falls outside the mask 6 From Show Markers choose whether or not to have mask violations displayed 7 If you are loading a pre existing mask touch the Load Mask tab then the File button You ...

Page 147: ...you can select them for viewing individually You can also set actions to occur automatically when unusual events are found stop the acquisition emit an audible beep output a pulse print the screen save the waveform or create a LabNotebook entry Signal Views WaveScan provides distinct views of your signal Source view highlights all occurrences of edges that meet your criteria Scan Overlay places al...

Page 148: ...a Sampling Mode Whenever WaveScan is enabled the instrument reverts to Real time sampling mode Source View The top trace on the screen is the source channel math memory trace This trace shows all captured edges and highlights those that fit your search criteria For example in this figure we are searching for slow rising edges that fall outside a time window In this acquisition WaveScan has located...

Page 149: ...log controls the same settings in the Display Æ Persistence setup dialog and vice versa In the acquisition shown below we have scanned for rise times greater than 1 4 ns WaveScan has located six edges that meet this criteria one of which is greater than 2 ns This slowest edge which appears in the persistence display has been selected for zooming from the table Note that it is also highlighted in t...

Page 150: ...a delta of 50 ps are accumulated in the histogram Another feature of WaveScan is that you can select a single bin of the histogram for analysis by touching or clicking it A confirmation pop up button then appears After Find Feature is confirmed only the single bin of the histogram remains and information about the contents of the bin is displayed in the Filter Method area You can recall the origin...

Page 151: ...oomed by selecting it from the table of found events at the left of the screen You can also scroll through the table using the Prev Next scroll buttons in the Search dialog or select an event by touching inside the Idx field and entering an index number using the pop up keypad ...

Page 152: ...o quickly scroll through the table In this case however the selection of edge will not change Note Press the Adjust knob for fine resolution green LED lights This will enable you to scroll through the table one line at a time In the coarse resolution mode the edge selection jumps several lines at once for each click of the Adjust knob Front panel Zoom controls can be used to vary the magnification...

Page 153: ...in a table You can select positive negative or both edges When the acquisition is stopped scan filters contained in Measurements mode can be applied to the edges to find specific characteristics A level marker corresponding to 50 in this case is displayed in the source trace at top As with other search modes a trigger action can be set for Edge mode also ...

Page 154: ...level are presented in a table and highlighted in the source trace The value displayed in the table is the difference of the max and min of the non monotonicity This can be confirmed with cursors The hysteresis value is used to eliminate noise A non monotonicity is detected only when its amplitude is greater than the hysteresis Therefore when setting a hysteresis level set a value that is greater ...

Page 155: ...g runts or both An adjustable hysteresis band is provided to eliminate noise In the case of negative going runt pulses the value displayed in the table is the difference delta of the high level of the signal and the runt amplitude i e where the runt bottoms out This can be confirmed by placing cursors on the runt pulse and reading the delta Y value in the trace labels ...

Page 156: ...WAVERUNNER XI SERIES 156 WRXi OM E Rev B In the case of positive going runt pulses the value displayed in the table is the absolute value of the amplitude of the runt pulse ...

Page 157: ... you can apply other filters to the acquisition or make different parameter measurements on it For example in this acquisition a Rise time 10 90 parameter measurement is applied to fast edges during acquisition We are interested only in edges with a rise time slower than 1 ns Therefore the Greater Than filter method is selected with a value of 1 ns four edges pass during acquisition ...

Page 158: ... times greater than 3 ns fifteen falling edges from the same acquisition pass this new filter You can also decide how anomalous the features located should be by using the Filter Wizard to select the average value as the limit to search for rarest events or to apply statistical criteria ...

Page 159: ...ere several slow edges in a repetitive waveform For each filtering method selectable from the wizard the rise time used as the filter limit and delta are calculated automatically Filter Methods While the Filter Wizard provides a quick way to apply filtering criteria automatically the Filter Method menu and related fields let you manually set up filtering criteria And unlike the Filter Wizard selec...

Page 160: ...ation between your PC and oscilloscope when you are operating the instrument remotely You can log all events or errors only This log can be invaluable when you are creating and debugging remote control applications Remote Communication Setup If you are connecting the oscilloscope to a network first contact your Information Systems administrator If you are connecting the oscilloscope directly to yo...

Page 161: ...name using the pop up keyboard Then touch the Export to Text File button Hardcopy Printing For print setup refer to Printing Clipboard This selection prints to the clipboard so you can paste a file into another application like MS Word for example PRINTING FROM THE CLIPBOARD 1 In the menu bar touch Utilities then Utilities Setup in the drop down menu 2 Touch the Hardcopy tab 3 Under Colors touch t...

Page 162: ...tion to e mail your screen images using either the MAPI or SMTP protocols Before you output to e mail from the Utilities dialog you first have to set up the e mail server and recipient address in Preference Setup SENDING E MAIL 1 In the menu bar touch Utilities then Utilities Setup in the drop down menu 2 Touch the Hardcopy tab then the E mail button 3 Touch inside the File Format field and select...

Page 163: ...lities Setup from the menu bar 4 Touch the Date Time tab 5 Touch the Set from Internet button Setting the Date and Time from Windows 1 Touch Utilities Æ Utilities Setup from the menu bar 2 Touch the Date Time tab 3 Touch the Windows Date Time button Use the Date and Time Properties window to configure the time including time zone Options Use this dialog to add or remove software options For inform...

Page 164: ... the waveform could move off the grid Note Regardless of whether you select Volts or Div the Offset shown in the channel setup dialog always indicates volts However when Div is selected for the Offset Control the offset in volts is scaled proportional to the change in gain thereby keeping the division on the grid constant 1 In the menu bar touch Utilities then touch Preferences in the drop down me...

Page 165: ...oose an e mail server protocol MAPI Messaging Application Programming Interface is the Microsoft interface specification that allows different messaging and workgroup applications including e mail voice mail and fax to work through a single client such as the Exchange client included with Windows 95 and Windows NT MAPI uses the default Windows e mail application usually Outlook Express SMTP Simple...

Page 166: ...ovides access to service dialogs which are for the sole use of LeCroy service personnel A security code is required to gain access Show Windows Desktop Touching the Show Windows Desktop button in the main Utilities dialog minimizes the instrument application to reveal the underlying desktop To maximize the application touch the appropriate shortcut icon Touch Screen Calibration Touching the Touch ...

Page 167: ... The reason for this is simple the oscilloscope contains a complex algorithm to determine in which order results are computed This algorithm ensures that all inputs required by a processor are computed before the processor itself If a VBScript processor decides to access via automation results other than those supplied to its inputs the oscilloscope s dependency algorithm cannot be used Therefore ...

Page 168: ... or two input waveforms Creating a new waveform without using any of the input data Creating a new parameter by manipulating the data of one or two input waveforms Changing a vertical scale or a horizontal scale from linear to non linear You don t have to use all the data from the input waveforms you can select data from one or more segments which need not be aligned in the two input waveforms Exa...

Page 169: ...ta SampleTime InResult HorizontalPerStep Omega 2 0 3 1416 Frequency SampleTime Amplitude 0 15 Amplitude of real data For K 0 To LastPoint newDataArray K Amplitude Sin Omega K Next OutResult DataArray True newDataArray Data in volts OutResult DataArray is the waveform returned to the oscilloscope and displayed on the oscilloscope as the F2 waveform ...

Page 170: ...to create a clock waveform of the appropriate frequency waveform F1 the customer was able to display and measure data clock skew using a LeCroy instrument function and parameter EXAMPLE 5 Next is a logarithmic vertical scale for which the script can be found here Most scripts would be far simpler than this one Frequency response curves are frequently drawn on a logarithmic scale The upper trace is...

Page 171: ...s like vertical scale and offset and horizontal scale and offset These functions then return a waveform or a parameter as required In addition you can view the calculated data directly in Excel MATLAB or Mathcad if you desire What is Excel Excel is a program within Microsoft Office With it you can place data in the cells of a spreadsheet calculate other values from them prepare charts of many kind...

Page 172: ... of useful variables from the oscilloscope including waveform data and useful variables such as volts per division and time per division The output from a script can be a waveform or a parameter and you can choose your own values for variables such as volts per division The idea of a VBS function is that you start with an input waveform operate on some or all of the values with a script and show t...

Page 173: ...nd vertical scales by manipulating the data Logarithmic scales in amplitude and frequency are often required Squaring and taking square roots are needed in certain applications Here is a picture showing some graphs related to white noise showing ways of detecting small deviations from the true distribution The lower two graphs were generated and placed in one trace using a VBScript In the next exa...

Page 174: ... 000 points 32 000 if you have created a chart in Excel Therefore make sure that your acquisition has less than this number of points if you are going to use an Excel calculation To use this capability you must have the LeCroy XMAP software option and Excel loaded in your instrument Select Minimize from the instrument s File menu to access the Excel program directly Selecting a Math Function Call ...

Page 175: ...cel and create as many charts as you want Organizing Excel sheets The Cells tab allows you to organize your Excel chart When placing the components in the sheet be careful to avoid over writing needed information especially when you are using multiple input waveforms As depicted here the instrument panel has been pasted over the Excel sheet There are three arrays of data for the three waveforms up...

Page 176: ... Inputs and Outputs If you invoke two or more instrument parameter functions or waveform functions that call Excel you will find that they all refer to the same spreadsheet by default Thus your spreadsheet can use the data from several waveforms and you can derive many different combinations of output parameters and waveforms including some of each from your spreadsheet You only have to be careful...

Page 177: ...dialog is the one that controls the location of the data in the Excel worksheet The input data are in columns A and B though only the first is used and the output is in column C All have been set to start at row 2 allowing space for a title in row 1 Columns D E and F contain the headers for the three waveforms These are the set of numbers that provide the description of the oscilloscope settings s...

Page 178: ...the following figure the panel has been pasted onto the Excel sheet for comparison To get the output values in column C we set C2 A2 and copy this formula down the column This is the only action needed in Excel and can be seen in the next figure ...

Page 179: ... columns A and B though by default only a single input column is used and the output is in column C All have been set to start at row 2 which allows for a header in row 1 Create this waveform by doing the following 1 Ensure that your acquisition has no more than 64 kpts the Excel calculation limit 2 Choose a function and select ExcelMath as Operator1 for the function Excel will open automatically ...

Page 180: ...t automatically opens Excel puts the waveform data in the correct columns and returns the calculated data back to the display as the chosen F trace This Excel calculated trace can have further measurements or math calculations performed on it if desired You can also create a chart of the data in Excel automatically and view the data there Simply press the Add Chart button in the instrument s Excel...

Page 181: ...is to measure the time constant TC2 using the portion of the trace where TC1 has negligible effect This was done using Function F1 which is not a part of the measurement process For the actual measurement Parameter P1 was set up as an Excel call In Excel the selected portion of the trace was converted to logarithms and the Excel function SLOPE was used as shown here Here we see the input data in c...

Page 182: ...cripts to ensure that successive acquisitions produced different data Script F1 generates pulses with widths that are multiples of a set clock period F2 generates one pulse in the first half of the time window and one pulse in the second half Both pulses are constrained to coincide with the clock pulses of F1 F1 and F2 are used here only as simulations and are not part of the measurement process w...

Page 183: ...in column C the cell C10 was given the following formula IF B10 B9 A 4 1 C9 C9 This was copied down the column Column D the output column is simply A C The output was defined as cell H3 The required mean in cell H3 is given by SUM D10 D509 SUM C10 C509 for a 500 point waveform Correlation Excel Waveform Function Excel Example 3 This example uses an Excel waveform function to examine the cross corr...

Page 184: ... traces in one picture with only two operations in an Excel sheet Depicted below is an example from an Excel spreadsheet Here is an original instrument trace The method is very simple First the waveform is transferred to an Excel spreadsheet by means of an instrument Excel call Second two operations are needed in Excel placing a simple formula in one cell and copying that formula into a range of c...

Page 185: ...required to start at cell B134 The traces repeat at intervals of 250 cells Let us call this interval R If we require a horizontal displacement D then in cell CN we write B N R D In this example D is 10 Finally we may want a vertical displacement V and we write B N R D V In this example V is 0 02 D and V can be zero if required as depicted below All that remains is to copy the formula to the requir...

Page 186: ...s of script The Waveform Function script allows you to take the data from one or two traces and make a new trace whose values may depend on the values of the input trace The Parameter Function script also takes in the data from one or two traces but it only has one output This output is the zeroth element in the output array It appears as a parameter value on the instrument s screen The remainder ...

Page 187: ...isible trace length 1 Note that a trace of nominal length 1000 comprises data numbered from 0 to 1001 The 1001st point is not visible so you normally use points 0 to 1000 giving 1001 points and 1000 intervals between points startData 0 endData OutResult Samples LastPoint endData 1 because the last point is invisible ReDim newArray OutResult Samples to store the results unscaledData InResult DataAr...

Page 188: ... OutResult Samples is the number of data in the output trace and is set to be the same as the number of data in the input trace If you set the output length less than the input length you get a shorter trace the remainder being made of zeroes If you try to set the output values to something illegal you may find that a part of the trace retains the values from a previous acquisition If you try to s...

Page 189: ...lt DataArray 257 Z Some parts of the default script must not be changed because they are a part of the interface These are highlighted in the following script TODO add your custom code here accessing OutResult and InResult objects Here s a small example that just inverts the waveform OutResult Samples InResult Samples startData 0 endData OutResult Samples newNumPoints endData startData ReDim newDa...

Page 190: ... to edit a parameter script click here The default parameter script is shown below TODO add your custom code here accessing OutResult and InResult objects Here s a small example that just inverts the waveform numParam InResult Samples ReDim newValueArray numParam scaledData InResult DataArray For i 0 To numParam 1 newValueArray i scaledData i _ Change this to do something useful Next OutResult Val...

Page 191: ...s equality is valid You can use variables in VBS without declaring the type The context may force an implicit type assignment For example if the result of a calculation is of a different type from the defined type the type may be changed Always set out calculations in such a way that type changes will not affect the final result in an undesirable or unpredictable way If you want to change the type...

Page 192: ...r B Division A B A divided by B Integer division A B A divided by B truncated to next integer below Multiplication A B A multiplied by B Addition A B B added to A Subtraction A B B subtracted from A PLEASE NOTE THE FOLLOWING If there is any possibility that you will be taking the exponent of a negative number make sure to trap any possible errors arising from such operations as trying to take the ...

Page 193: ... brackets Exponentiation Division and multiplication Addition and subtraction If there is any doubt as to how the calculation will be done use brackets These will also make the order of the calculations clear to any reader of the program which is desirable if you are to give it to a customer who will want to know what was intended Here are some examples of the uses of brackets Brackets are worked ...

Page 194: ... Else End If Select Case End Select While Wend Choose the construction that best satisfies the requirements of speed and clarity The construction GoTo LabelledStatement is available in many languages including VBA but not in VBS GOTO is not allowed in VBS IF Then Else End If A very simple example If A 0 Then B Sqr A Take the square root of A if A is not negative If A B C D Then E F G H_ No End Is ...

Page 195: ...statements are Boolean logic statements which can take the values True or False You may even see things like If A Then B which means that if A is True B gets done In the first example if A is negative we might want to write something like this If A 0 Then B Sqr A Else B 0 End If and in fact you can make some very complex constructions using If as in the examples below If A 0 Then If A 1 Then Z 17 ...

Page 196: ...hat should be equal may differ minutely after a computation It is safer with Real numbers to test using a tolerance band File for this example IfThenElse xls If you find that you are building up a rather complicated set of Ifs you might want to consider the Select Case construction Select Case This is a very powerful construction which is also easy to understand when written out It is best for Int...

Page 197: ...uction is useful when you do not know at programming time how many times the loop will be executed Here are some examples Do AnyVBSCalculation Loop Until D Pi Do Until Z Y AnyVBSCalculation Loop Do AnyVBSCalculation Loop While D Pi Do While Y Z AnyVBSCalculation Loop These constructions enable you to make the test before or after the calculation If before the calculation might not be done even one...

Page 198: ...n Omega N Next Be careful about changing the counting variable in any loop You can do this to terminate the loop early but Exit For is better but you could also prevent it from terminating at all For emergency exit you can use Exit For For example For K 0 To Total If HistogramBin K 0 Then Exit For AnyVBScripting Next It is possible to make a For loop with steps greater than 1 as in the following e...

Page 199: ...cosine of an angle expressed in radians Csng Convert a number to single precision floating point Cstr Convert a variable to a string Exp Raise e to the power of the input Get Get a value from a file Input Get some ASCII data from a file Instr Find the position of a string in a longer string Int Convert to nearest integer below the input value Left Take some characters at the left end of a string L...

Page 200: ...as If A B Then Functions These are mainly of the form C F A where A is the argument or input to the function Abs Abs A calculates the absolute value of an integer or a real number so the result is always positive or zero A can be any number in the range of the VB system Atn Atn A calculates the angle of which A is the tangent Because infinitely many angles can have the same tangent the output of A...

Page 201: ... You can get some very frustrating problems if you are in the wrong mode You can cut and paste the VBS statements if you discover this error If your calculation requires a long memory development might be quicker if you test the principles on a shorter trace at first Note that the pseudo random number generator is reset at the start of a script If you want a different set of pseudo randoms every t...

Page 202: ...ll need to unzoom it to see it all This feature can be used to avoid compaction problems with non linear horizontal scales It can also be used to show several versions of a function at the same time without having to set up a separate script for each one If your program structure is complicated consider typing all the IFs ELSEIFs ENDIFs FORs NEXTs etc and then clicking Apply You wont get any outpu...

Page 203: ... errors and exceptions You must be aware of all possibilities at all points in your program and you must either be certain that errors will not occur or you must take action to ensure that they do not Examples Sqr You cannot take the square root of a negative number Log You cannot take the log of zero or of a negative number A B You cannot divide by zero Array You cannot use an index outside the b...

Page 204: ...Please note that VBS is much slower than the internal computations of the instrument so do everything you can to save time unless time is irrelevant to the application Using an array element takes longer than using a single variable Here is an example For K 1 to Total If X K X K 1 Then Y Cos X K Sin X K Sqr X K End If Next To do the same thing we could also write this using the index only once Old...

Page 205: ...requency domain for example Example Waveform Script Custom Window Creating a window function for FFT calculations Example Parameter Scripts Decay Parameter Calculating the rate of decay of a damped sine Locating Pulses Finding pulses in a pulse train Debugging Scripts Until we have integrated a more comprehensive debugger for VBScript there is a workaround Download the Windows Scripting Debugger f...

Page 206: ...rticalPerStep Double precision Difference between successive possible levels in the output waveform memory 1 65536 of vertical full scale InResult VerticalResolution Double precision Difference between successive possible physical levels in the input waveform OutResultVerticalResolution Double precision Difference between successive possible physical levels in the output waveform 1 256 of vertical...

Page 207: ...le pVal HorizontalFrameStop in double newVal VerticalFrameStart out retval double pVal VerticalFrameStart in double newVal VerticalFrameStop out retval double pVal VerticalFrameStop in double newVal VerticalResolution out retval double pVal VerticalResolution in double newVal VerticalPerStep out retval double pVal VerticalPerStep in double newVal VerticalOffset out retval double pVal VerticalOffse...

Page 208: ...TLAB Note Load MATLAB version 6 5 just as you would on any PC Once it is loaded open MATLAB from the desktop then close it again before you attempt to open it from the instrument application This is to update the registry MATLAB can be directly called from the instrument in two ways Using a function F1 through Fx The number of math traces available depends on the software options loaded on your os...

Page 209: ...on MATLAB Wave there will be a slight pause before MATLAB starts Source 1 and Source 2 are the waveforms that MATLAB will use The MATLAB Waveform Control Panel Once you have invoked a MATLAB waveform call you will see the zoom dialog at the right of the screen Touch the MATLAB tab to see a panel like this Touch Find Scale to make your output fit the grid or use the text boxes to choose a scale ...

Page 210: ...r because the output values will not be shown in MATLAB Response With a long waveform the time needed to display it could be quite long The response values can be useful during development and debugging Any line without a semicolon will produce a visible MATLAB Response From this panel you can save your code load a previous code and edit your function A powerful feature of MATLAB is that you can r...

Page 211: ... Rev B 211 This very simple example adds a rescaled copy of Channel 2 to a copy of Channel 1 and then rescales the result MATLAB Example Waveform Plot If you touch the MATLAB Plot checkbox you will see a MATLAB plot like this one ...

Page 212: ...B parameter call in Select Measurement menu The MATLAB Parameter Control Panel Once you have invoked a MATLAB parameter call a mini dialog to the right of the main dialog will appear You can touch the MATLAB Plot checkbox if you want to see a plot in MATLAB as well as getting a result in the instrument ...

Page 213: ... be shown in the MATLAB Response pane for an amplitude of 0 15 volt You can load an existing MATLAB program using the Load Code button and you can save the current program using the Save Code button If you are familiar with MATLAB you might prefer to launch MATLAB and create a MATLAB function that performs your task Your program in the instrument could then be a one line call of your MATLAB functi...

Page 214: ...the ratio of the number of data points that are above a given level to the number of points below the level in this case one half of the amplitude Further Examples of MATLAB Waveform Functions Negate the input signal Square the input signal Create pulses from a sinusoid ...

Page 215: ...The procedure is simple Create a MATLAB function using any text editor and save it as a MATLAB m file by giving it a name of the form Filename m Call the function using the MATLAB math editor or the MATLAB parameter editor as appropriate A simple example is shown below function out negatewf wf1 NEGATEWF changes the sign of all the data out wf1 ...

Page 216: ...ered by routines written in Visual Basic With ActiveX controls you can create your own user interfaces to suit your own preferences A large number of interactive devices are available button checkbox radio button list box picture box and common dialogue box Invoking CustomDSO CustomDSO can be invoked from the Analysis drop down menu If CustomDSO is already in Basic mode the following dialog will b...

Page 217: ...163 CustomDSO ActionEnable1 False 1164 CustomDSO ActionScript1 c LeCroy XStream CustomDSO B lss 1165 CustomDSO ActionEnable1 True 1166 CustomDSO ActionScript1 c LeCroy XStream CustomDSO C lss 1167 CustomDSO ActionEnable1 True 1168 CustomDSO ActionScript1 c LeCroy XStream CustomDSO A lss 1169 CustomDSO ActionEnable1 False 1160 Set CustomDSO XStreamDSO CustomDSO 1161 CustomDSO Setup B lss 1162 Custo...

Page 218: ...de all the information needed to set the DSO to the required state But if you want to make a very short file that changes only a few variables for example the action button settings you can make a file that includes only the relevant instructions This usage assumes that the remainder of the DSO is already in the required state This is an example of the complete compatibility of the instrument s so...

Page 219: ...properties to Single and Auto respectively Create code for the buttons A Double click on the Single button B In the resulting code window insert code to make the following subroutine Private Sub SingleButton_Click Dim app as Object Set app CreateObject LeCroy XStreamApplication app Acquistion TriggerMode Stopped End Sub Double click on the Auto button In the resulting code window insert code to ma...

Page 220: ...r exact requirements Among the most useful properties are the following Height Width BackColor Name Caption You can gain access to the properties of your objects by Clicking View Properties Positions and sizes of objects can be changed from View Object by dragging the object or one of its handles You can insert any available control into your plug in The basic control set is shown in a toolbar at ...

Page 221: ...ow select the Microsoft Tabbed Control as shown below and click on Apply The control will be added into the toolbox at the left of the screen where you can double click on it as usual The new control is shown arrow The system is very versatile and you can place controls on the tabs of the Tabbed Control Look in the properties window to see how you can customize your tabs as illustrated below ...

Page 222: ...mber of math traces available depends on the software options loaded on your oscilloscope See Specifications and it exchanges the first two it finds whenever the button is pressed Note that arrays of objects can be constructed allowing numerous objects to be accessed in simple loops Private Sub Command1_Click Dim wm As Object Set wm CreateObject LeCroy XStreamApplication Set acq wm Acquisition To ...

Page 223: ...If Loop Until trace 12 Or views 2 Show the parity of the last swap If Command1 Caption Swap A Then Command1 Caption Swap B Else Command1 Caption Swap A End If Dim TextString As String TextString Text1 Text Dim TextValue As Integer TextValue Val TextString 1 TextString Str TextValue TextString Trim TextString Text1 Text TextString End Sub This routine exchanges the first two traces that it finds Yo...

Page 224: ...lue Here is the result after seven swaps The counting method could be useful in any routine where numerous operations such as triggers have to be performed In fact the caption of the button could have been used to show the number of operations ActiveX offers a large range of standard controls including list boxes for selection from a list and picture boxes for drawing graphs and charts ...

Page 225: ...s This has great potential for testing the transmission characteristics of amplifiers and filters since the output can be compared with a theoretical curve Furthermore if the output is divided by the curve the result for a perfect DUT would be a horizontal line which is easy to inspect The example below has been magnified vertically by a factor of ten The rise at the right side occurs because the ...

Page 226: ...1 Out Result DataArray Dim Black White Blue Red As Long Black 0 White HFFFFFF Blue HFF4444 Red HFF Draw a theoretical curve for the peaks StartPoint Top 20 EndPoint 54 5 Picture1 Line 0 StartPoint Samples EndPoint Red Draw the plot with linear interpolation between points For X 1 To Samples LogX XScale Log X Y Wave X If X 1 Then Picture1 Line LogX Y OldLogX OldWave Black End If OldLogX LogX OldWav...

Page 227: ...e is shared among several users for example or used for different projects the data can be kept separately Similarly hardcopy reports can be stored in different folders Preferences You should set your preferences before creating notebook entries Miscellaneous Settings You can elect to name notebook entries with the default date and time by leaving the top box unchecked Check the box if you want th...

Page 228: ...ms will appear on a black background A drawing toolbar appears at top The pen tool enables you to write or draw in freehand You can use a mouse or a stylus to do this using the touch screen Once you click off you can drag your note anywhere on your waveform The circle tool enables you to create a circle around a waveform feature that you want to point out Once you click off the circle is drawn and...

Page 229: ... to enable it and touch OK The next object that you create will be in that color If you want to erase a drawing object touch it to select it then touch Erase Selected Touch Erase All to erase all drawn objects and text Touch Undo to discard the last object drawn The Move Toolbar button enables you to place the toolbar anywhere on the screen Touch the button a second time to return it to its origin...

Page 230: ... Certain math operations result in the creation of floating point waveforms with much higher resolution than can be stored in a 16 bit waveform file This extra resolution will not be preserved when traces are recalled using flashback Cumulative Measurements Any measurements that are on when the Lab Notebook entry is created are not saved individually in the database other than being embedded in th...

Page 231: ...st box Creating the Report 1 Select a notebook entry in the list box 2 Touch inside the Format field and select a report format from the pop up menu 3 Touch the Create Report button A dialog box opens in which to name the report and select a folder to contain the report Touch inside the File name field and enter a name using the pop up keyboard If you want to e mail or print the data to a network ...

Page 232: ...ide the Logo field and enter the name and path to the file using the pop up keyboard Otherwise touch the Use Default checkbox to use LeCroy s logo Note If you elect to use your own logo bit map do not use a bit map larger than 180 pixels height x 100 pixels width Managing Notebook Entry Data Adding Annotations You can add annotations to your notebook entry at any time 1 Touch the LabNotebook tab 2...

Page 233: ...ase You can begin a new database for your notebook entries at any time back up the current one or compress the data TO SELECT A DATABASE FOR BACKUP OR COMPRESSION 1 Touch the Advanced tab 2 Touch the Browse button A navigation window opens Navigate to the database you want to work on 3 Touch Compact to reduce the size of a database This function defragments the notebook after a large amount of ent...

Reviews:

No comments

Brands by name

Popular brands

Load more brands