20090601
I
Dirac Delta Function
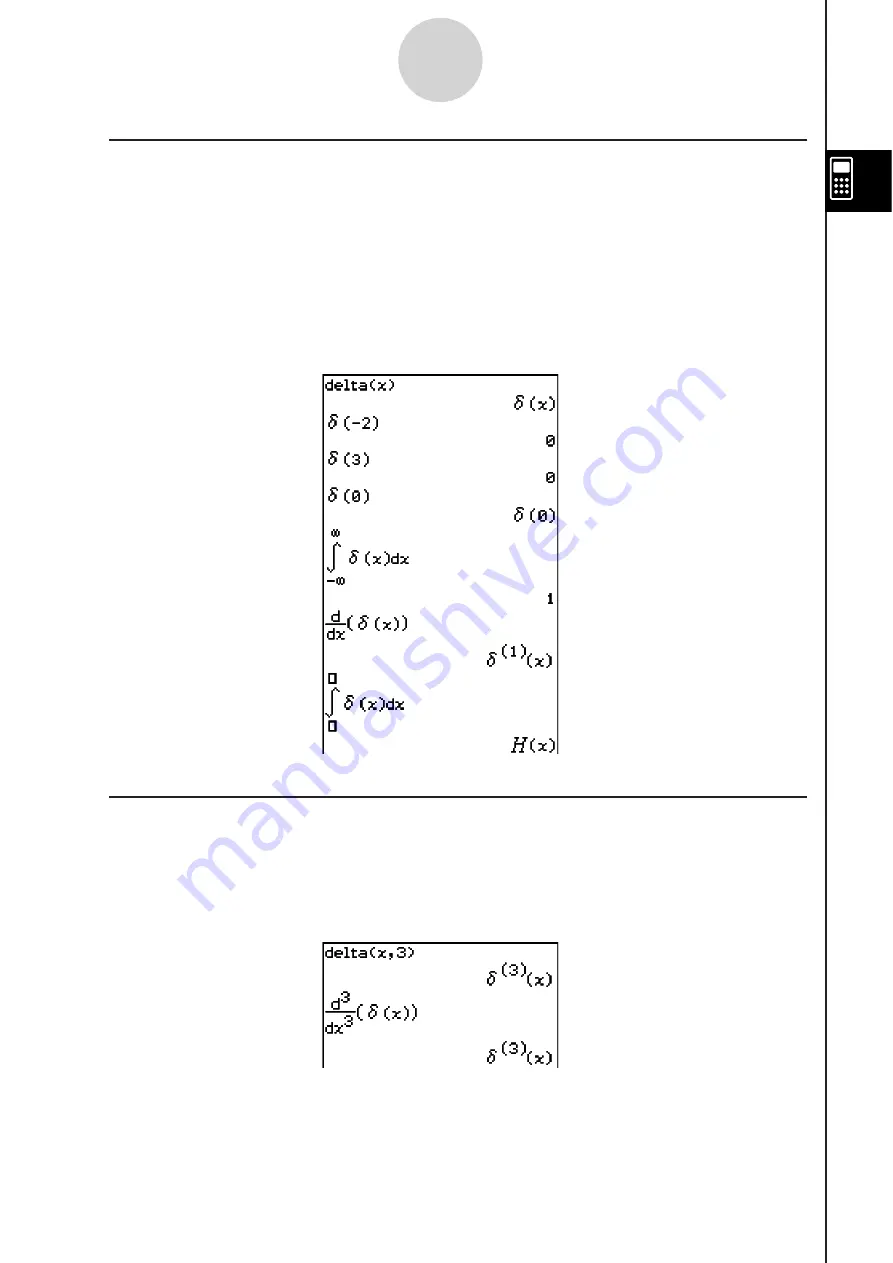
“delta” is the Dirac Delta function. The delta function evaluates numerically as shown below.
0,
x
x
0
D
(
x
) =
{
D
(
x
),
x
= 0
Non-numeric expressions passed to the delta function are left unevaluated. The integral of a
linear delta function is a Heaviside function.
Syntax:
delta(
x
)
x
: variable or number
Example
s
:
I
n
th
Delta Function
The
n
th
-delta function is the
n
th differential of the delta function.
Syntax:
delta(
x
,
n
)
x
: variable or number
n
: number of differentials
Example
s
:
2-4-16
Function Calculations
Содержание ClassPad 330
Страница 11: ...20060301 20090601 ClassPad 330 ClassPad OS Version 3 04 ...
Страница 277: ...20060301 3 3 10 Storing Functions 3 Tap AND Plot OR Plot ...
Страница 779: ...20090601 S Graph Line Clustered C S Graph Line Stacked E 13 9 2 Graphing ...
Страница 780: ...20090601 S Graph Line 100 Stacked F S Graph Column Clustered G 13 9 3 Graphing ...
Страница 781: ...20090601 S Graph Column Stacked I S Graph Column 100 Stacked J 13 9 4 Graphing ...
Страница 782: ...20090601 S Graph Bar Clustered K S Graph Bar Stacked 9 13 9 5 Graphing ...





































