System Overview
R&S
®
ZVA
72
Quick Start Guide 1145.1090.62 ─ 10
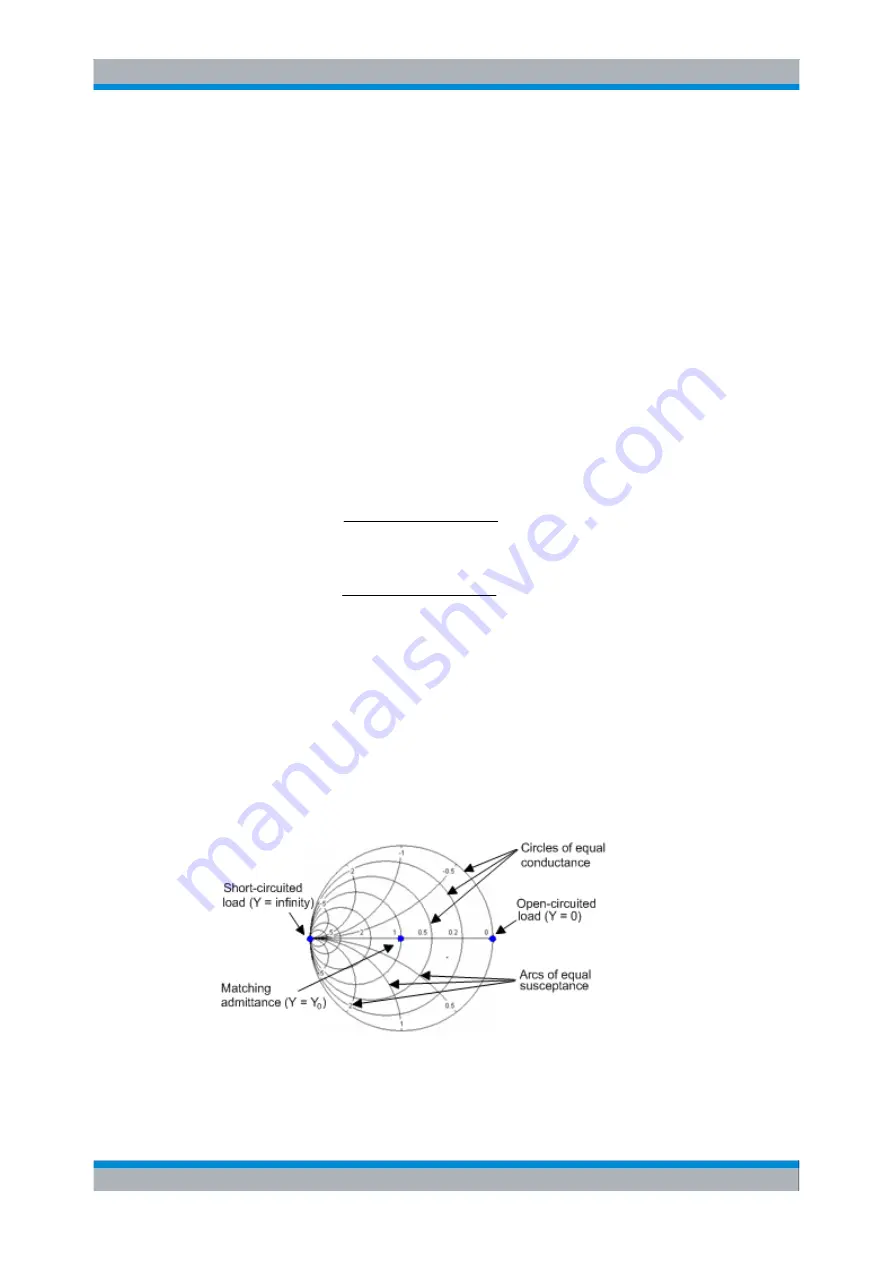
the system (zero reflection). At the left and right intersection points between the hor-
izontal axis and the outer circle, the admittance is infinity (short) and zero (open).
●
The outer circle corresponds to zero conductance (purely imaginary admittance).
Points outside the outer circle indicate an active component.
●
The upper and lower half of the diagram correspond to negative (inductive) and pos-
itive (capacitive) susceptive components of the admittance, respectively.
Example: Reflection coefficients in the inverted Smith chart
If the measured quantity is a complex reflection coefficient G (e.g. S
11
, S
22
), then the unit
inverted Smith chart can be used to read the normalized admittance of the DUT. The
coordinates in the normalized admittance plane and in the reflection coefficient plane are
related as follows (see also: definition of matched-circuit (converted) admittances):
Y / Y
0
= (1 -
Γ
) / (1 +
Γ
)
From this equation it is easy to relate the real and imaginary components of the complex
admittance to the real and imaginary parts of
Γ
:
2
2
2
2
0
)
Im(
)
Re(
1
)
Im(
)
Re(
1
)
/
Re(
Y
Y
G
,
)
Im(
)
Re(
1
)
Im(
2
)
/
Im(
2
2
0
Y
Y
B
According to the two equations above, the graphical representation in an inverted Smith
chart has the following properties:
●
Real reflection coefficients are mapped to real admittances (conductances).
●
The center of the
Γ
plane (
Γ
= 0) is mapped to the reference admittance Y
0
, whereas
the circle with |
Γ
| = 1 is mapped to the imaginary axis of the Y plane.
●
The circles for the points of equal conductance are centered on the real axis and
intersect at Y = infinity. The arcs for the points of equal susceptance also belong to
circles intersecting at Y = infinity (short circuit point (–1, 0)), centered on a straight
vertical line.
Examples for special points in the inverted Smith chart:
●
The magnitude of the reflection coefficient of a short circuit (Y = infinity, U = 0) is one,
its phase is –180 deg.
Screen Elements






























