Page 19-1
Chapter 19
Numbers in Different Bases
In this Chapter we present examples of calculations of number in bases other
than the decimal basis.
Definitions
The number system used for everyday arithmetic is known as the
decimal
system for it uses 10 (Latin, deca) digits, namely 0-9, to write out any real
number. Computers, on the other hand, use a system that is based on two
possible states, or
binary
system. These two states are represented by 0 and
1, ON and OFF, or high-voltage and low-voltage. Computers also use
number systems based on eight digits (0-7) or
octal
system, and sixteen digits
(0-9, A-F) or
hexadecimal
. As in the decimal system, the relative position of
digits determines its value. In general, a number n in base b can be written
as a series of digits n = (a
1
a
2
…a
n
.c
1
c
2
…c
m
)
b
. The “point” separates
n
“integer” digits from m “decimal” digits. The value of the number, converted
to our customary decimal system, is calculated by using
n = a
1
⋅
bn
-1
+ a
2
⋅
b
n-2
+ … + a
n
b
0
+ c
1
⋅
b
-1
+ c
2
⋅
b
-2
+ … +c
m
⋅
b
-m
. For example, (15.234)
10
= 1
⋅
10
1
+
5
⋅
10
0
+ 2
⋅
10
-1
+ 3
⋅
10
-2
+ 4
⋅
10
-3
, and (101.111)
2
= 1
⋅
2
2
+ 0
⋅
2
1
+ 1
⋅
2
0
+
1
⋅
2
-1
+ 1
⋅
2
-2
+ 1
⋅
2
-3
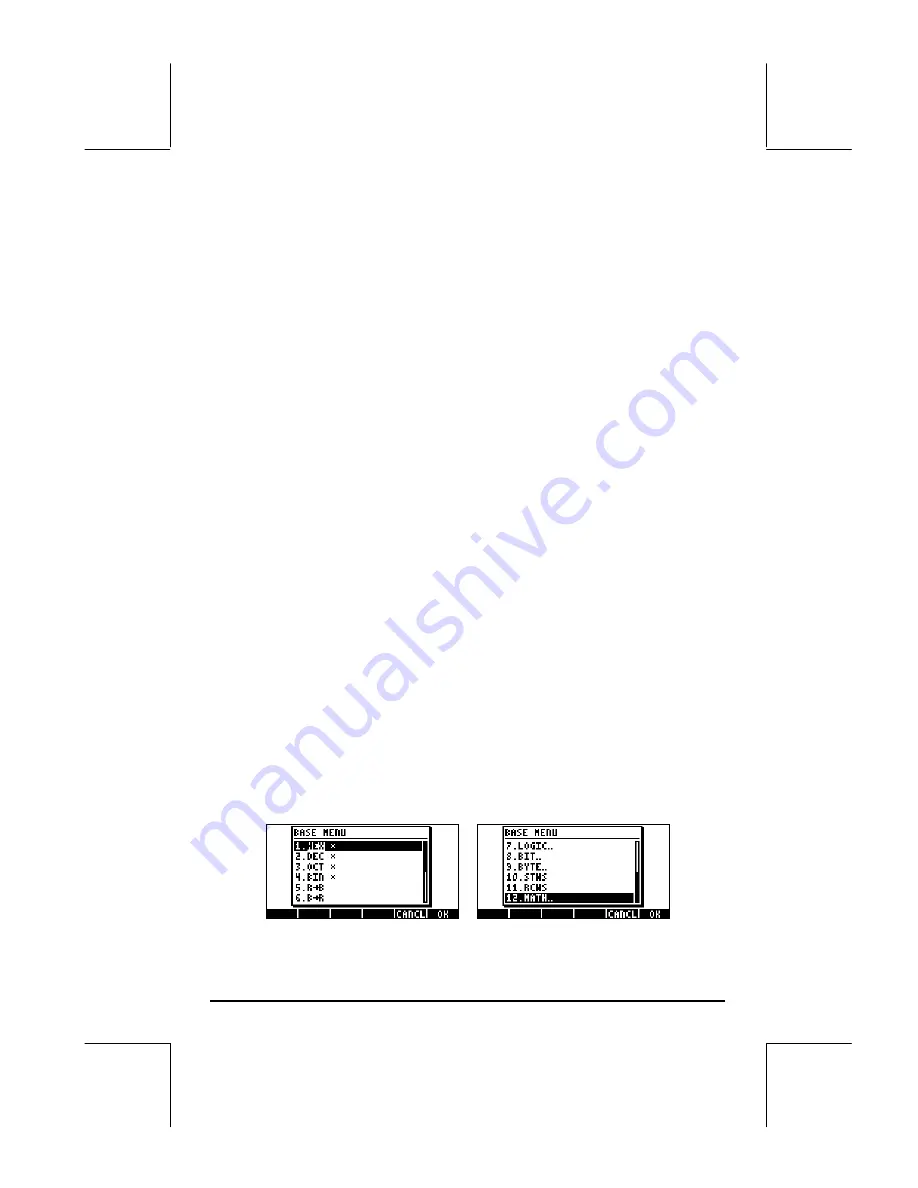
The BASE menu
While the calculator would typically be operated using the decimal system,
you can produce calculations using the binary, octal, or hexadecimal system.
Many of the functions for manipulating number systems other than the decimal
system are available in the BASE menu, accessible through
‚ã
(the
3
key). With system flag 117 set to CHOOSE boxes, the BASE menu shows
the following entries:
















































