Page 16-12
function LAP you get back a function of X, which is the Laplace transform of
f(X).
Example 2 – Determine the Laplace transform of f(t) = e
2t
⋅
sin(t). Use:
‘EXP(2*X)*SIN(X)’
`
LAP The calculator returns the result: 1/(SQ(X-2)+1).
Press
µ
to obtain, 1/(X
2
-4X+5).
When you translate this result in paper you would write
5
4
1
}
sin
{
)
(
2
2
+
⋅
−
=
⋅
=
s
s
t
e
s
F
t
L
Example 3 – Determine the inverse Laplace transform of F(s) = sin(s). Use:
‘SIN(X)’
`
ILAP. The calculator takes a few seconds to return the result:
‘ILAP(SIN(X))’, meaning that there is no closed-form expression f(t), such that f(t)
= L
-1
{sin(s)}.
Example 4 – Determine the inverse Laplace transform of F(s) = 1/s
3
. Use:
‘1/X^3’
`
ILAP
µ
. The calculator returns the result: ‘X^2/2’, which is
interpreted as L
-1
{1/s
3
} = t
2
/2.
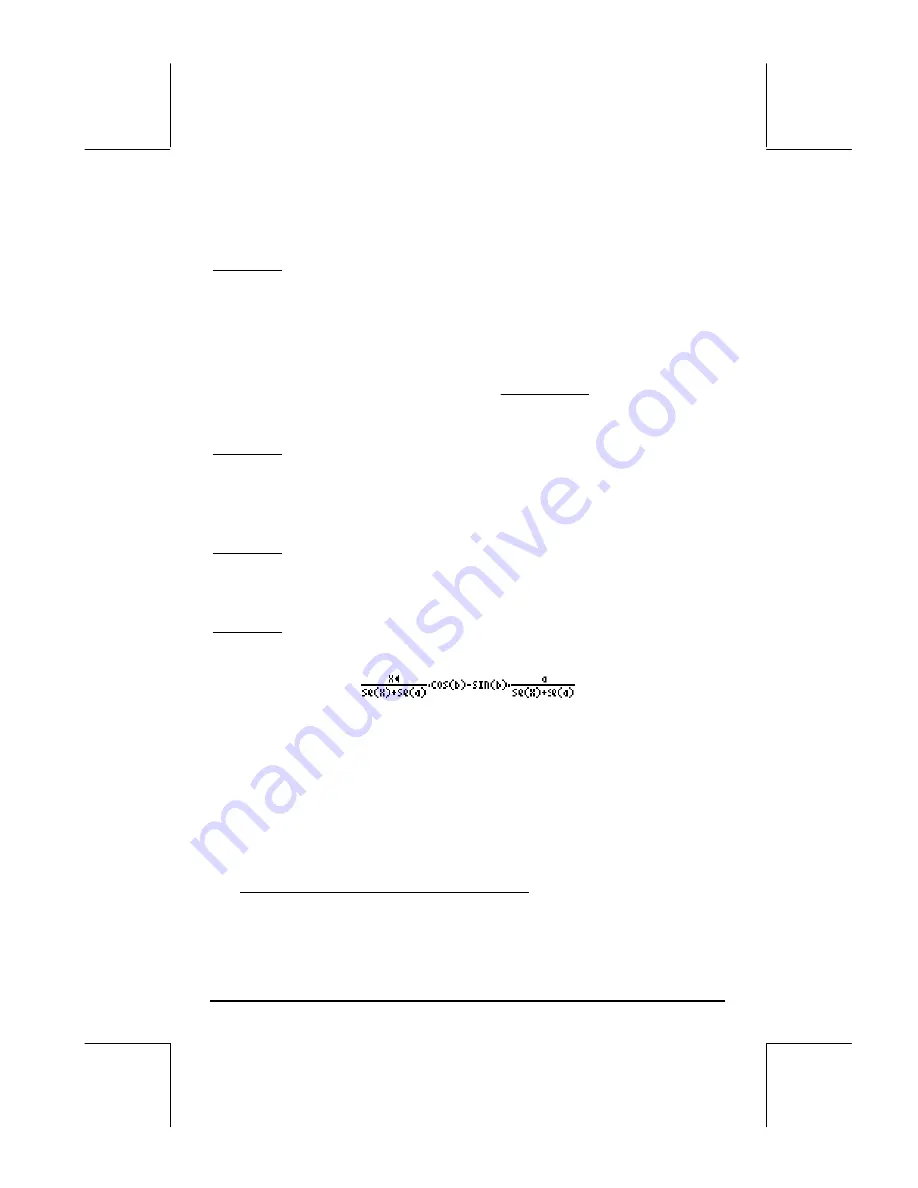
Example 5 – Determine the Laplace transform of the function f(t) = cos (a
⋅
t+b).
Use: ‘COS(a*X+b)’
`
LAP . The calculator returns the result:
Press
µ
to obtain –(a sin(b) – X cos(b))/(X
2
+a
2
). The transform is
interpreted as follows: L {cos(a
⋅
t+b)} = (s
⋅
cos b – a
⋅
sin b)/(s
2
+a
2
).
Laplace transform theorems
To help you determine the Laplace transform of functions you can use a
number of theorems, some of which are listed below. A few examples of the
theorem applications are also included.
•
Differentiation theorem for the first derivative. Let f
o
be the initial condition
for f(t), i.e., f(0) = f
o
, then






























