HP 48GII, User Manual, Page: 563 / 864

Page 17-10
],
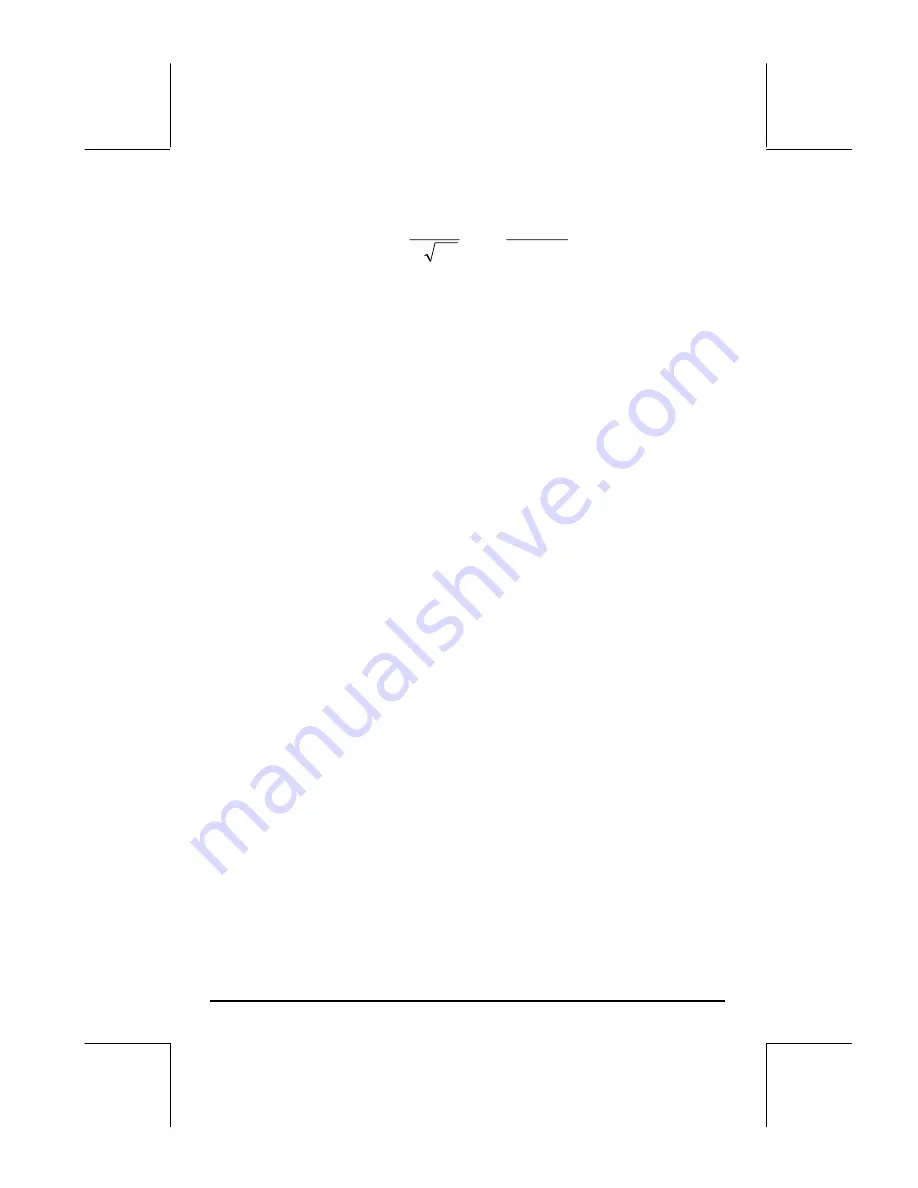
2
) (
exp[
2
1
) (
2
2
σ
µ
π σ
−
− =
x
x f
where
µ
is the mean, and
σ 2
is the variance of the distribution. To calculate
the value of f(
µ
,
σ
2
,x) for the normal distribution, use function NDIST with the
following arguments: the mean,
µ
, the variance,
σ
2
, and, the value x , i.e.,
NDIST(
µ
,
σ 2
,x). For example, check that for a normal distribution,
f(1.0,0.5,2.0) = 0.20755374.
Normal distribution cdf
The calculator has a function UTPN that calculates the upper-tail normal
distribution, i.e., UTPN(x) = P(X>x) = 1 - P(X<x). To obtain the value of the
upper-tail normal distribution UTPN we need to enter the following values: the
mean,
µ
; the variance,
σ
2
; and, the value x, e.g., UTPN((
µ
,
σ
2
,x)
For example, check that for a normal distribution, with
µ
= 1.0,
σ 2
= 0.5,
UTPN(0.75) = 0.638163. Use UTPN(1.0,0.5,0.75) = 0.638163.
Different probability calculations for normal distributions [X is N(
µ
,
σ 2
)] can be
defined using the function UTPN, as follows:
•
P(X<a) = 1 - UTPN(
µ, σ 2
,a)
•
P(a<X<b) = P(X<b) - P(X<a) = 1 - UTPN(
µ, σ 2
,b) - (1 - UTPN(
µ, σ 2
,a))
= UTPN(
µ, σ 2
,a) - UTPN(
µ, σ 2
,b)
•
P(X>c) = UTPN(
µ, σ
2
,c)
Examples: Using
µ
= 1.5, and
σ 2
= 0.5, find:
P(X<1.0) = 1 - P(X>1.0) = 1 - UTPN(1.5, 0.5, 1.0) = 0.239750.
P(X>2.0) = UTPN(1.5, 0.5, 2.0) = 0.239750.
P(1.0<X<2.0) = F(1.0) - F(2.0) = UTPN(1.5,0.5,1.0) - UTPN(1.5,0.5,2.0)
= 0.7602499 - 0.2397500 = 0.524998.