Page 18-45
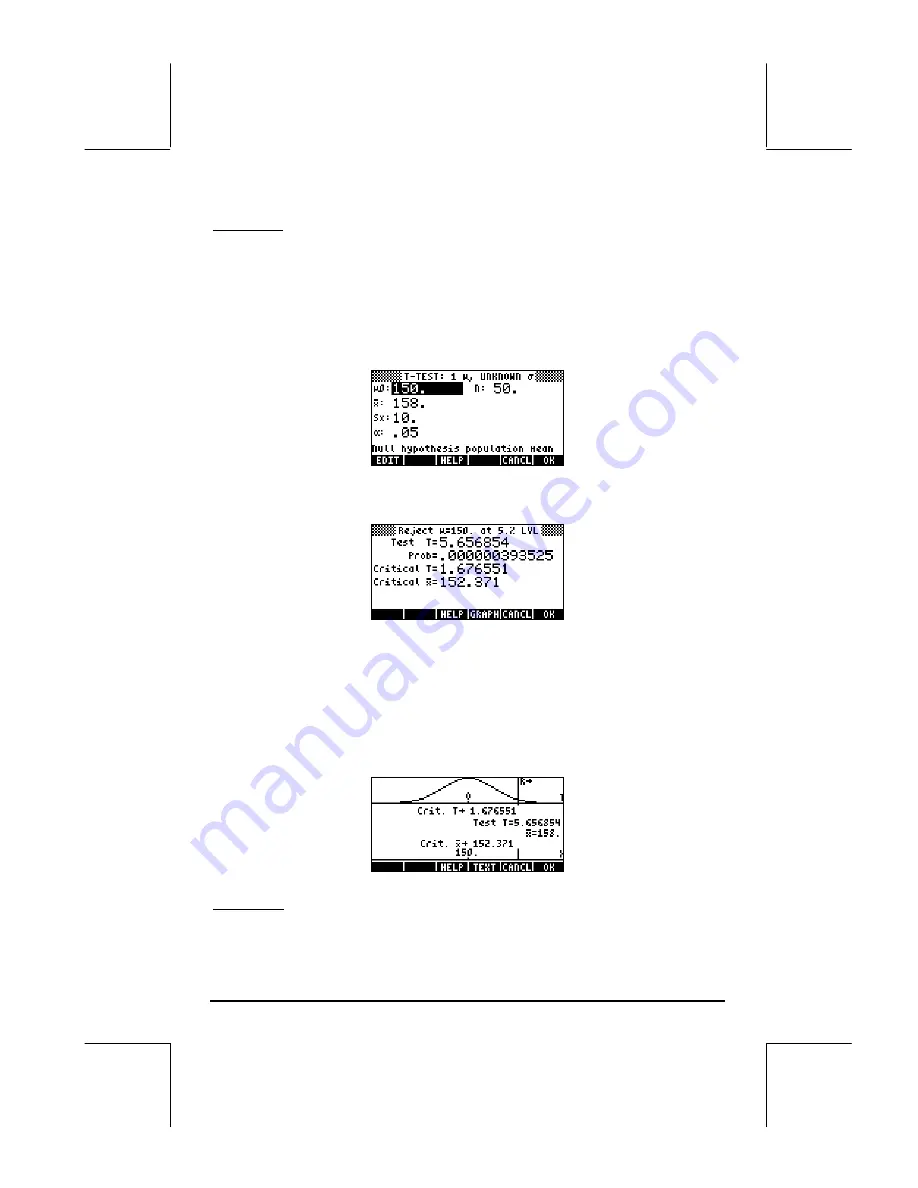
Example 2 -- For
µ
0
= 150,
x = 158, s = 10, n = 50, for
α
= 0.05, test the
hypothesis H
0
:
µ
=
µ
0
, against the alternative hypothesis, H
1
:
µ
>
µ
0
. The
population standard deviation,
σ
, is not known.
Press
‚Ù——
@@@OK@@@
to access the hypothesis testing feature in the
calculator. Press
——
@@@OK@@@
to select option 5. T-Test: 1
µ
.:
Enter the following data and press
@@@OK@@@
:
Select the alternative hypothesis, H
1
:
µ
> 150, and press
@@@OK@@@
. The result is:
We reject the null hypothesis, H
0
:
µ
0
= 150, against the alternative hypothesis,
H
1
:
µ
> 150. The test t value is t
0
= 5.656854, with a P-value =
0.000000393525. The critical value of t is t
α
= 1.676551, corresponding to
a critical
x = 152.371.
Press
@GRAPH
to see the results graphically as follows:
Example 3 – Data from two samples show that
x
1
= 158,
x
1
= 160, s
1
=
10, s
2
= 4.5, n1 = 50, and n
2
= 55. For
α
= 0.05, and a “pooled”






























