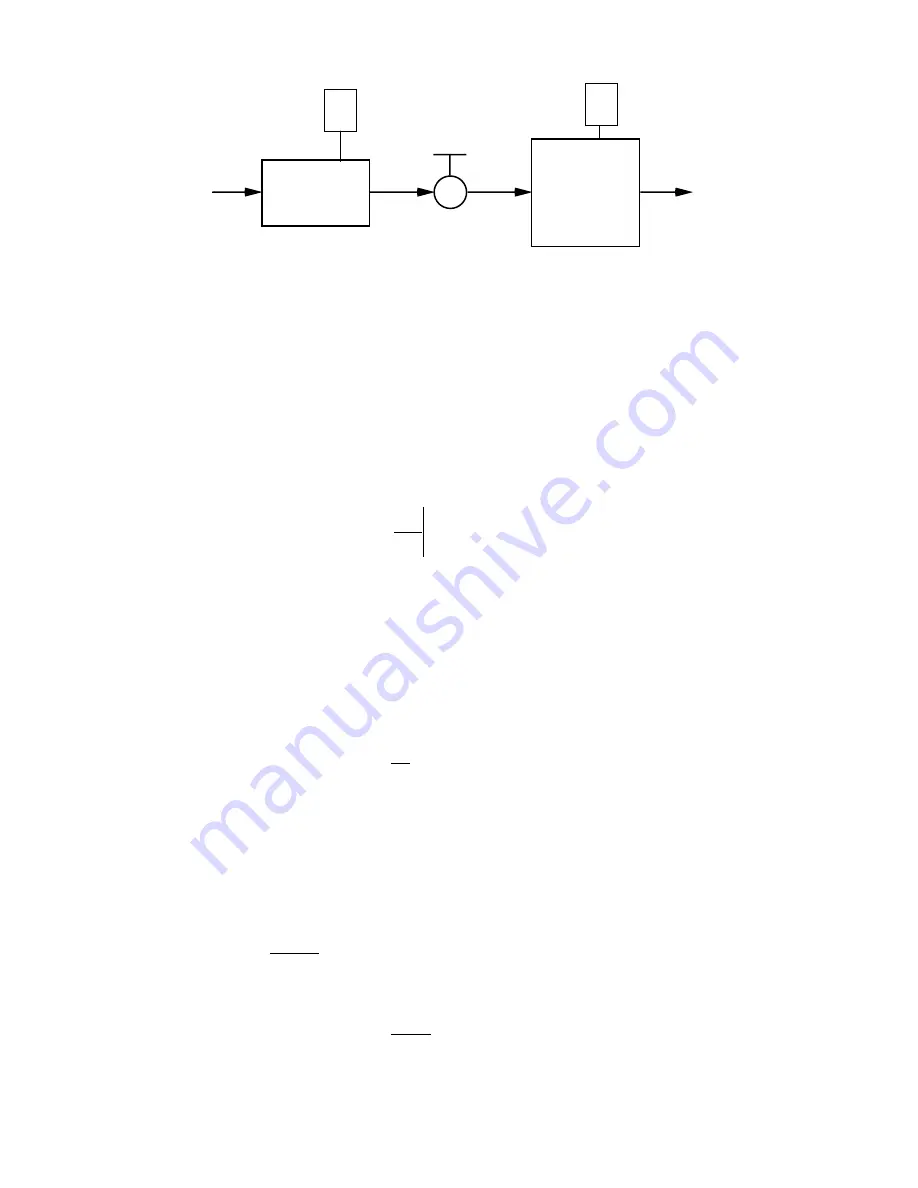
1-2
Figure 1-1
1.2.2 RH Formula For Ideal Gas
The relative humidity formula, equation 1 given in section 1.2.1, is a correct relationship
between pressures and relative humidity when dealing with perfectly isothermal conditions
and perfectly ideal gases. However, under dynamic conditions where some slight
temperature differences do exist and since gases do not behave ideally, any expectation of
this equation to accurately represent the actual relative humidity would be overly optimistic.
In its strictest form, relative humidity is defined in terms of mole fractions and is given as
%RH
=
w
v
X
X
P,T
·100
where
X
v
= the mole fraction of water vapor in a sample of
moist air at a specific pressure,
P
, and temperature,
T
,
and
X
w
= the mole fraction of water vapor which would exist in
a sample of air if it were saturated with water vapor at
the same pressure,
P
, and temperature,
T
, as the
unsaturated sample
Xv
.
The mole fraction of water vapor in a sample of gas is given by
X
=
P
v
P
where
P
v
= the partial pressure of the gas which is exerted by the
water vapor constituent alone, and
P
= the absolute (or total) pressure of the gas, which is
also equal to the sum of the partial pressures exerted
by the water vapor and dry air constituents.
When a gas is fully saturated with water vapor, the partial pressure,
P
v
, exerted by the water
vapor constituent is a known quantity,
e
w
(T)
, and is termed "the saturation vapor pressure of
air with respect to water". Since, at saturation,
P
v
= e
w
(T)
, the mole fraction equation of a
saturated gas may be written as
X
=
e
w
(T)
P
TEST CHAMBER
SATURATOR
AIR SUPPLY
EXHAUST
EXPANSION
VALVE
P
s
P
c














































