Gx
Series
EN - 159
13.14. VOLTAGE AND CURRENT HARMONICS
Any periodical non-sine wave can be represented as a sum of sinusoidal waves having
each a frequency that corresponds to an entire multiple of the fundamental, according to
the relation:
)
t
sin(
V
V
v(t)
k
k
1
k
k
0
(1)
where: V
0
= average value of v(t)
V
1
= amplitude of the fundamental of v(t)
V
k
= amplitude of the k
th
harmonic of v(t)
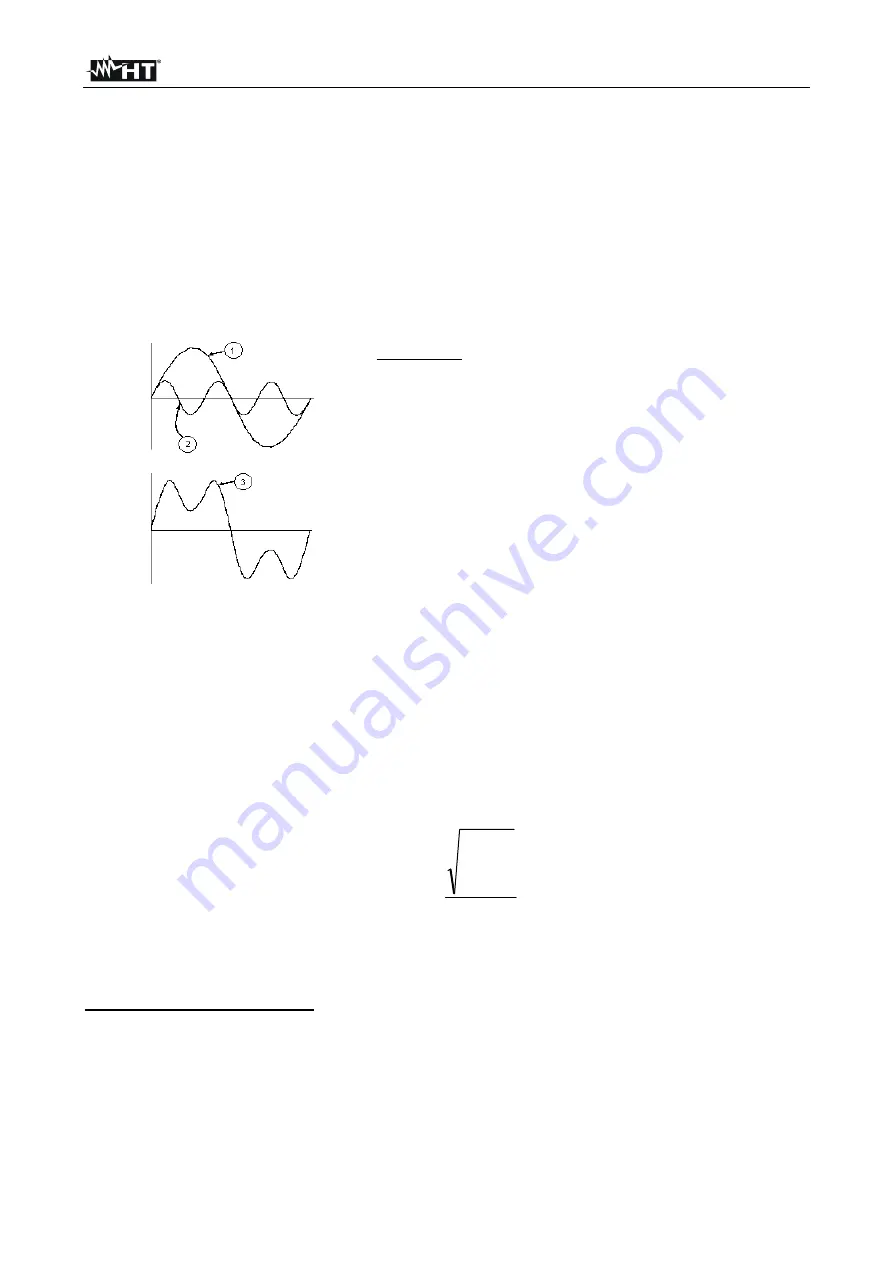
CAPTION:
1. Fundamental
2. Third harmonic
3. Distorted waveform
Fig. 51: Effect of the sum of two multiple frequencies
In the mains voltage, the fundamental has a frequency of 50 Hz, the second harmonic has
a frequency of 100 Hz, the third harmonic has a frequency of 150 Hz and so on. Harmonic
distortion is a constant problem and should not be confused with short events such as
sags, surges or fluctuations.
It can be noted that in (1) the index of the sigma is from 1 to the infinite. What happens in
reality is that a signal does not have an unlimited number of harmonics: a number always
exists after which the harmonics value is negligible. The EN 50160 standard recommends
to stop the index in the expression (1) in correspondence of the 40
th
harmonic. A
fundamental element to detect the presence of harmonics is THD defined as:
1
40
2
2
V
V
THDv
h
h
This index takes all the harmonics into account. The higher it is, the more distorted the
waveform gets.
Limit values for harmonics
EN 50160 guideline fixes the limits for the harmonic voltages, which can be introduced into
the network by the power supplier. In normal conditions, during whatever period of a week,
95% if the RMS value of each harmonic voltage, mediated on 10 minutes, will have to be
inferior than or equal to the values stated in Table 10. The total harmonic distortion (THD)
of the supply voltage (including all the harmonics up to 40
th
order) must be inferior than or
equal to 8%.



















