14
Theoretical Background
Theoretical basis of 2D-LC
2D-LC User Guide
368
Peak Capacity
Peak capacity may be differently defined:
•
As the maximum number of peaks that can be resolved in the available
separation space (
Geometrical Definition
), or
•
As the ratio of the total area of the chromatogram to the area required for the
resolution of any zone (
General Definition
)
Geometrical
Definition
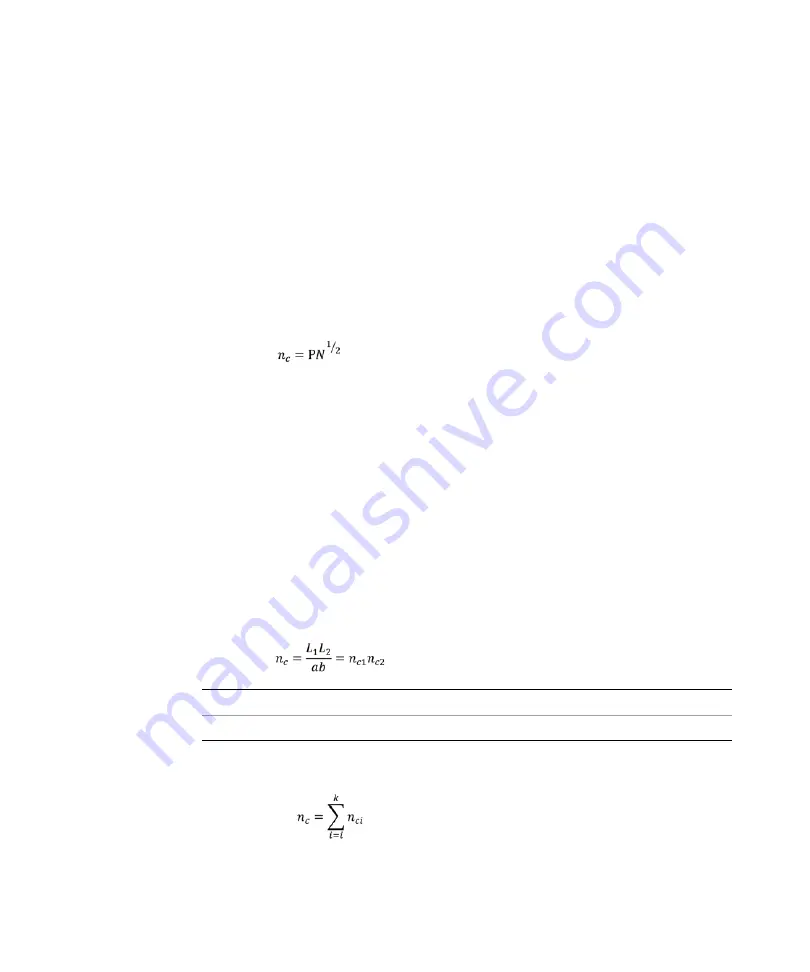
The peak capacity may be defined as the maximum number of peaks that can be
resolved in the available separation space. So peak capacity n
c
is related to the
number of theoretical plates N:
(
depends on the retention time range)
In practice peaks are usually not distributed randomly over the chromatogram
and often overlap. Or in other words: In practice peaks don't fill the available
separation space evenly. This is the reason, why the number of detectable
components of a sample in 1D-LC is relatively small.
2D-LC separation offers an alternative possibility for increasing n
c
: Orthogonal
retention mechanisms generate a separation plane. Thus, the peak capacity in
2D-LC is the product of the peak capacities of the individual columns. Due to
peak broadening in 1
st
and 2
nd
dimension, components in 2D-LC are present as
two-dimensional ellipses on the retention plane.
How to calculate n
c
depends on the method:
•
For comprehensive 2D-LC:
•
For heart-cutting 2D-LC:
L = Separation space for dimension
ab = Area for rectangle circumscribing the ellipse on the separation plane






























