Casio FX-CG10, User Manual

Page: 105 / 608

2-56
u
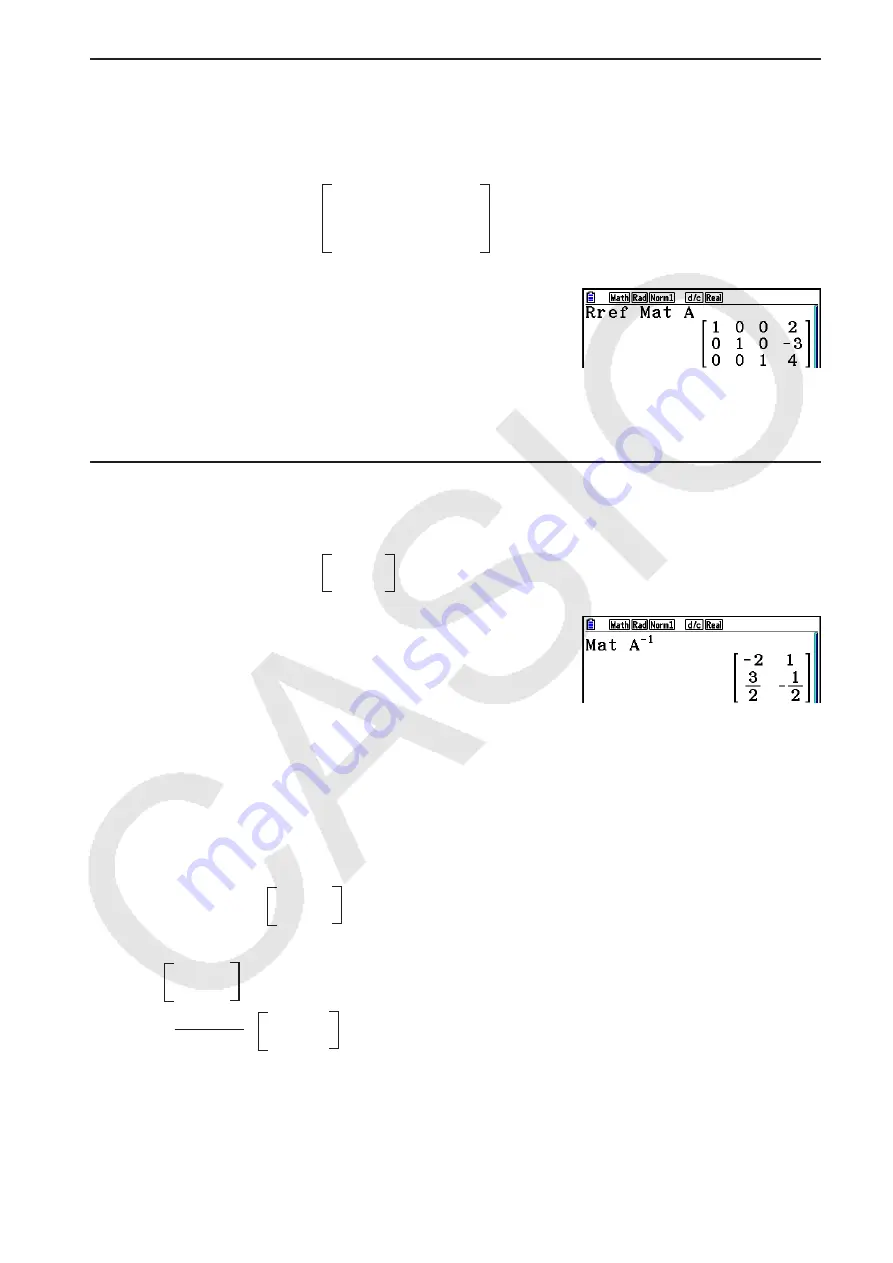
Reduced Row Echelon Form
[OPTN] - [MAT/VCT] - [Rref]
This command finds the reduced row echelon form of a matrix.
Example To find the reduced row echelon form of the following matrix:
Matrix A =
K
2
(MAT/VCT)
6
(
g
)
5
(Rref)
6
(
g
)
1
(Mat)
av
(A)
w
• The row echelon form and reduced row echelon form operation may not produce accurate
results due to dropped digits.
u
Matrix Inversion
[
x
–1 ]
Example To invert the following matrix:
Matrix A =
K
2
(MAT/VCT)
1
(Mat)
av
(A)
!
)
(
x
–1 )
w
• Only square matrices (same number of rows and columns) can be inverted. Trying to invert a
matrix that is not square produces an error.
• A matrix with a determinant of zero cannot be inverted. Trying to invert a matrix with
determinant of zero produces an error.
• Calculation precision is affected for matrices whose determinant is near zero.
• A matrix being inverted must satisfy the conditions shown below.
A A
–1
= A
–1
A = E =
1 0
0 1
The following shows the formula used to invert Matrix A into inverse matrix A –1 .
A =
a b
c d
A
–1
=
1
ad – bc
d –b
–c a
Note that ad – bc
≠
0.
2 −1 3 19
1 1 −5 −21
0 4 3 0
2 −1 3 19
1 1 −5 −21
0 4 3 0
1 2
3 4
1 2
3 4