60
••••
LAI theory
Document code: SS1-UM-1.05
Accuracy of LAI calculations
When used to predict LAI from transmitted fraction, the functions used in the
SunData software are accurate to within ±10% ±0.1 over the range of LAI less than
10 and Zenith Angle less than 60° when compared to the output of the full model.
The errors become larger for highly vertical leaves with a strong low sun, and users
should avoid these conditions if possible.
In practice, the greatest errors are likely to follow from the differences between the
real canopy and the idealised assumptions in the model.
Functions used to model canopy transmission
Diffuse light - cosine response sensor
The transmission of diffuse light through a canopy, as measured by a cosine
corrected sensor, can be modelled by the following functions:
Given:
These curves give maximum errors of 0.009 in
ττττ
diff
, with a maximum 6% error for
ττττ
diff
greater than 0.01 over the range
L
= 0 to 10 and
x
= 0 to 1000.
Diffuse light - hemispherical response sensor.
The previous analysis of diffuse light transmission is appropriate for a cosine-
corrected sensor as it only considers the vertical component of the incident and
transmitted light. This works as long as the leaf absorption in the PAR band is
complete, and there is no scattering of the incoming light.
When we consider leaves with incomplete PAR absorption, some of the absorbed
light is re-emitted in different directions to the original incoming light. Because of
this we have to account for all of the incoming light, both horizontal and vertical
components, and also be aware that the spatial intensity distribution of the light is
modified by the canopy and varies through the canopy depending on the canopy leaf
angle distribution.
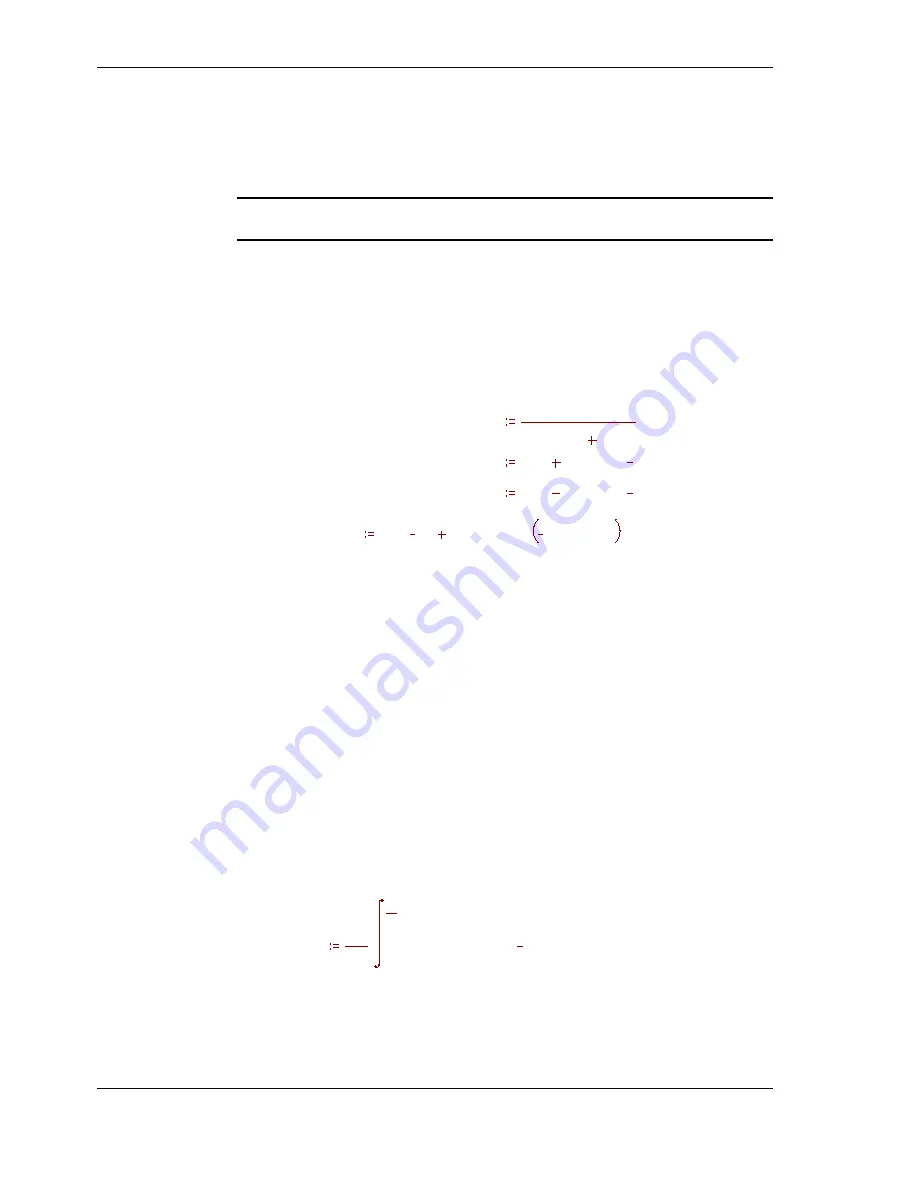
The above analysis is now repeated to find the transmission of uniform diffuse light
as measured by a sensor with a hemispherical response. The equivalent equation for
the transmission fraction is:
τ
spher(
)
,
x L
.
1
.
2
π
d
0
π
2
θ
.
.
.
2
π
sin ( )
θ
exp(
)
.
K (
)
,
x
θ
L
A ( )
x
1
.
0.15 x
1.38
0.007
B ( )
x
4.32
.
2.60 exp(
)
.
2.75 x
C( )
x
0.57
.
0.23 exp(
)
.
1.40 x
τ
diff(
)
,
x L
exp(
)
L
.
.
A ( )
x
L
3
exp
.
B ( )
x
L
C( )
x
















































