LTC3115-1
23
Rev. C
APPLICATIONS INFORMATION
The denominator of the buck mode transfer function
exhibits a pair of resonant poles generated by the LC
filtering of the power stage. The resonant frequency of
the power stage, f
O
, is given by the following expression
where L is the value of the inductor:
f
O
=
1
2π
R +R
S
LC
O
R +R
C
(
)
≅
1
2π
1
LC
O
The quality factor, Q, has a significant impact on compen-
sation of the voltage loop since a higher Q factor produces
a sharper loss of phase near the resonant frequency. The
quality factor is inversely related to the amount of damp-
ing in the power stage and is substantially influenced
by the average series resistance of the power stage, R
S
.
Lower values of R
S
will increase the Q and result in a
sharper loss of phase near the resonant frequency and will
require more phase boost or lower bandwidth to maintain
an adequate phase margin.
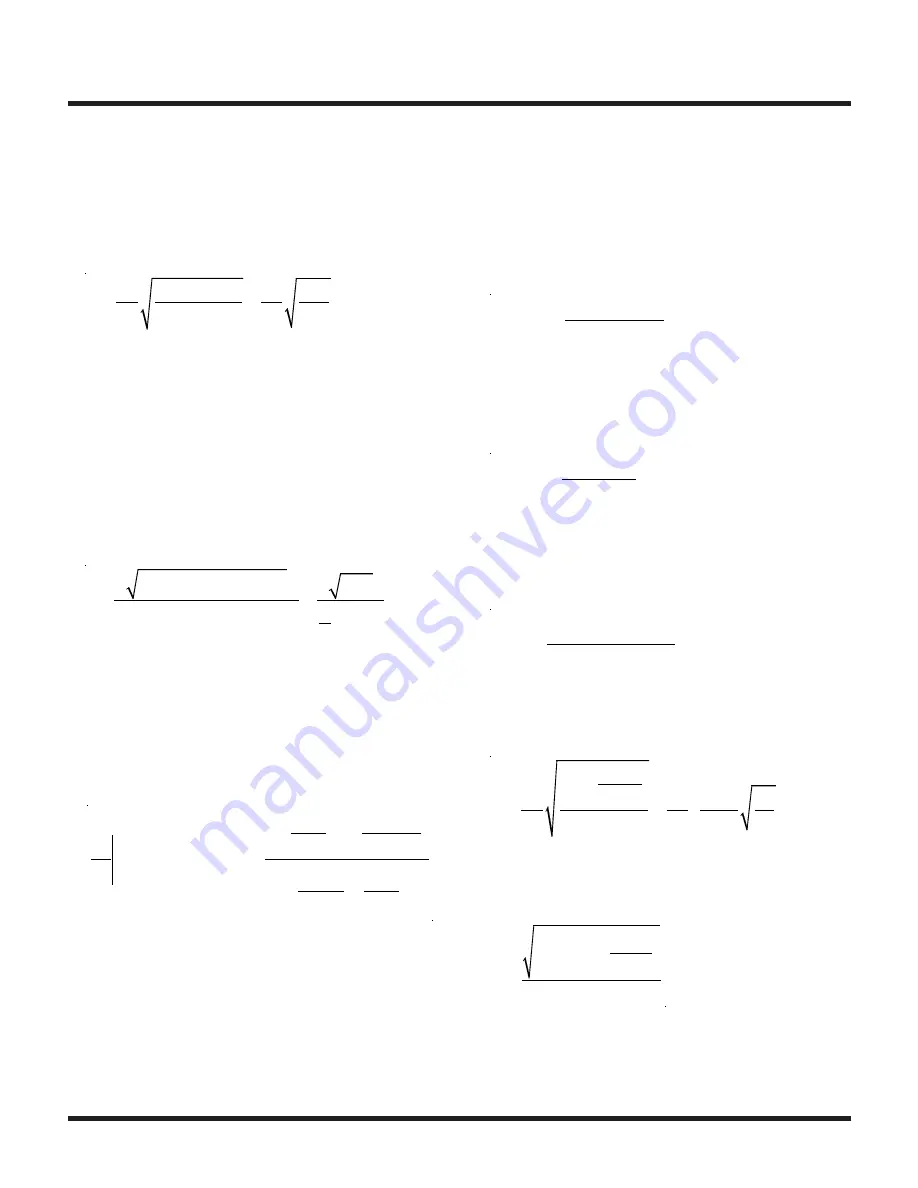
Q =
LC
O
R +R
C
(
)
R +R
S
(
)
RR
C
C
O
+L +C
O
R
S
R +R
C
(
)
≅
LC
O
L
R
+C
O
R
S
Boost Mode Small-Signal Model
When stepping up from a lower input voltage to a higher
output voltage, the buck-boost converter will operate in
boost mode where the small-signal transfer function from
control voltage, V
C
, to the output voltage is given by the
following expression.
V
O
V
C BOOST MODE
=G
BOOST
1+
s
2πf
Z
1–
s
2πf
RHPZ
1+
s
2πf
O
Q
+
s
2πf
O
2
In boost mode operation, the transfer function is charac-
terized by a pair of resonant poles and a zero generated by
the ESR of the output capacitor as in buck mode. However,
in addition there is a right half plane zero which generates
increasing gain and decreasing phase at higher frequen-
cies. As a result, the crossover frequency in boost mode
operation generally must be set lower than in buck mode
in order to maintain sufficient phase margin.
The boost mode gain, G
BOOST
, is comprised of three com-
ponents: the analog divider, the pulse width modulator
and the power stage. The gain of the analog divider and
PWM remain the same as in buck mode operation, but
the gain of the power stage in boost mode is given by the
following equation:
G
POWER
≅
V
OUT2
1– t
LOW
f
(
)
V
IN
By combining the individual terms, the total gain in boost
mode can be reduced to the following expression. Notice
that unlike in buck mode, the gain in boost mode is a
function of both the input and output voltage.
G
BOOST
≅
29.7V
OUT2
V
IN2
In boost mode operation, the frequency of the right half
plane zero, f
RHPZ
, is given by the following expression.
The frequency of the right half plane zero decreases at
higher loads and with larger inductors.
f
RHPZ
=
R 1– t
LOW
f
(
)
2
V
IN
2
2π L V
OUT2
In boost mode, the resonant frequency of the power
stage has a dependence on the input and output voltage
as shown by the following equation.
f
O
=
1
2π
R
S
+
RV
IN2
V
OUT2
L C
O
R +R
C
(
)
≅
1
2π
•
V
IN
V
OUT
1
LC
Finally, the magnitude of the quality factor of the power
stage in boost mode operation is given by the following
expression.
Q =
LC
O
R R
S
+
RV
IN
2
V
OUT2
L+C
O
R
S
R






























