Waldorf QUANTUM, Руководство пользователя

Страница: 209 / 231

Appendix
209 Quantum Manual
Waveform Oscillator Introduction
The oscillator is the core block of a synthesizer. It delivers
the signal that is transformed by all other components of
the synthesizer. In the early days of electronic synthesis,
Robert A. Moog found out that most real acoustic instru-
ment waveforms could be reproduced by using abstracted
electronic versions of these waveforms. He wasn’t the first
who came to that conclusion, but he was the first to recrea-
te them electronically and build them into a machine that
could be used commercially. What he implemented into his
synthesizer were the still well-known waveforms
sawtooth, square and triangle. For sure, this is only a mi-
nimal selection of the endless variety of waveforms, but
the Waldorf Quantum provides all those waveforms, plus
other classic waveforms like square (which is the father of
the pulse waveform) and the sine wave (also part of every
other waveform).
You probably know how these waveforms look and sound,
but the following chapter gives you a small introduction
into the deeper structure of these waveforms. Let’s start
with the most basic one.
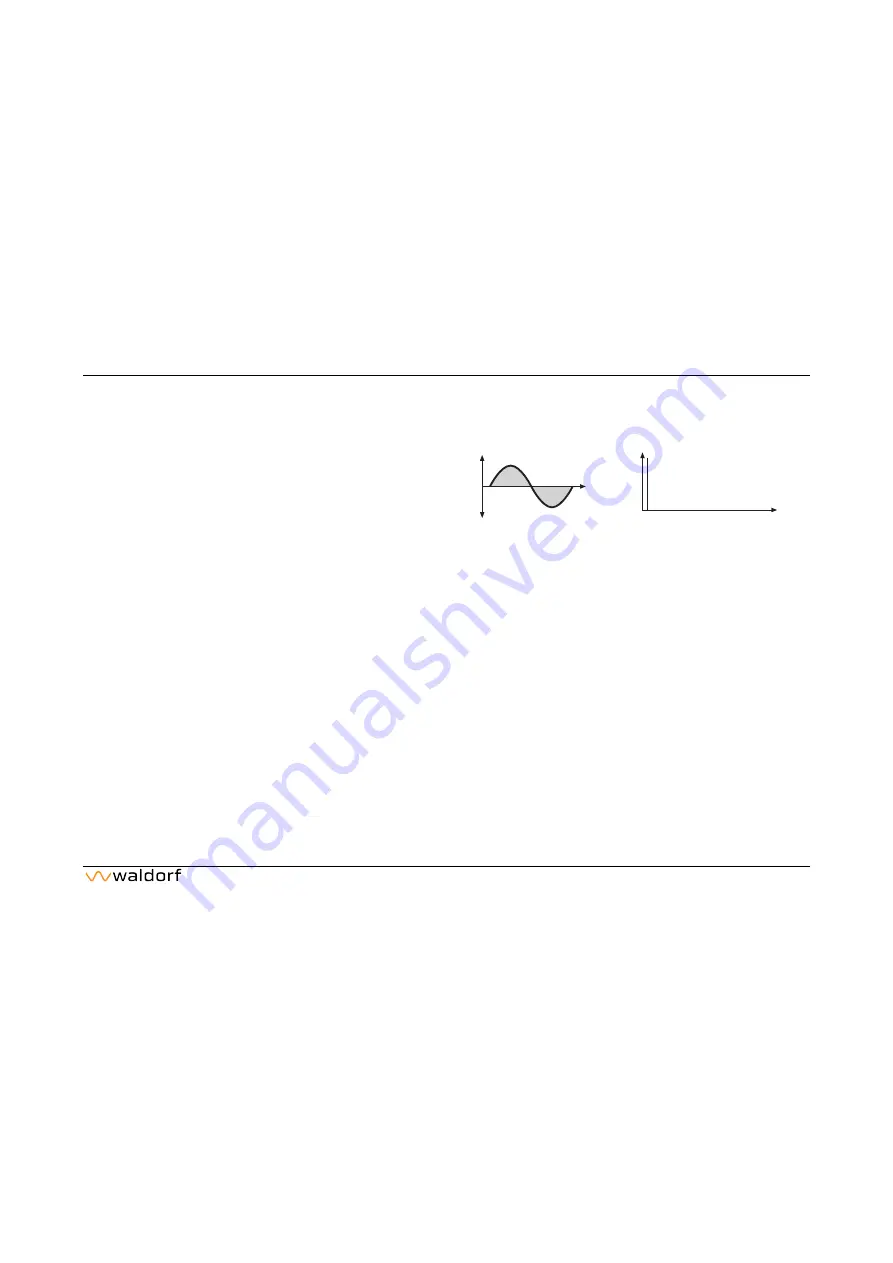
The Sine Wave
The Sine Wave is the purest tone that can be generated. It
consists of only one harmonic, the fundamental, and has no
overtones. The following picture shows the sine wave and
its frequency representation:
The Sine Wave
There is no acoustic musical instrument that generates a
pure sine wave; the only instrument that comes close to it
is the pitch fork. Therefore, the sine wave sounds a little
artificial to the ear. However, the sine wave can be an
interesting oscillator waveform to emphasize a certain
harmonic while other oscillators are playing more complex
waveforms, or as an FM source for frequency modulation.
The sine wave is the most basic building block of each
waveform. Any waveform can be broken down into several
(or many) sine waves that are arranged with different
frequencies and magnitudes. These sine waves are called
partials . In most waveforms the partial with the lowest
frequency is dominant, meaning that this partial is used by
the ear to determine the pitch of the tone. This partial is
called the
fundamental . All other partials are called overto-
nes . So the second partial is the first overtone.
! " # !$ !%
&'()*(+,-
./0+12*3(
415(
657812*3(
9/'5:+1,;