V1.02
Thom Hogan’s Complete Guide to the Nikon D300
Page 63
Tonal Range
The sensor in the D300 can capture images with either 12-bit
or 14-bits of data for each photosite position. There’s a drop
in frame per second shooting performance if you choose 14-
bits, but unless you’re shooting action, you might want to
select the higher value when you shoot NEF images
21
22
.
14 bits-per-pixel tonal range may not seem like much, but it
translates into the ability to render 16384 shades (using 14
bits) of an individual color versus 4096 (using 12 bits) or 256
(using 8 bits)
23
. While the capability of most human eyes is
close to what an 8-bit capture contains (our eyes are usually
said to distinguish about 16 million colors, which is
approximately what 8-bit RGB produces; 256 x 256 x 256 =
16,777,216), the extra tonality of 14 bit captures is still useful.
When we “sharpen” and apply other corrections to an image
in post-processing, it is usually easier to keep such
manipulations from becoming visible with the extra bits (i.e.
we can “hide” some of our manipulation in the extra tonality,
and rounding errors have less visible consequences).
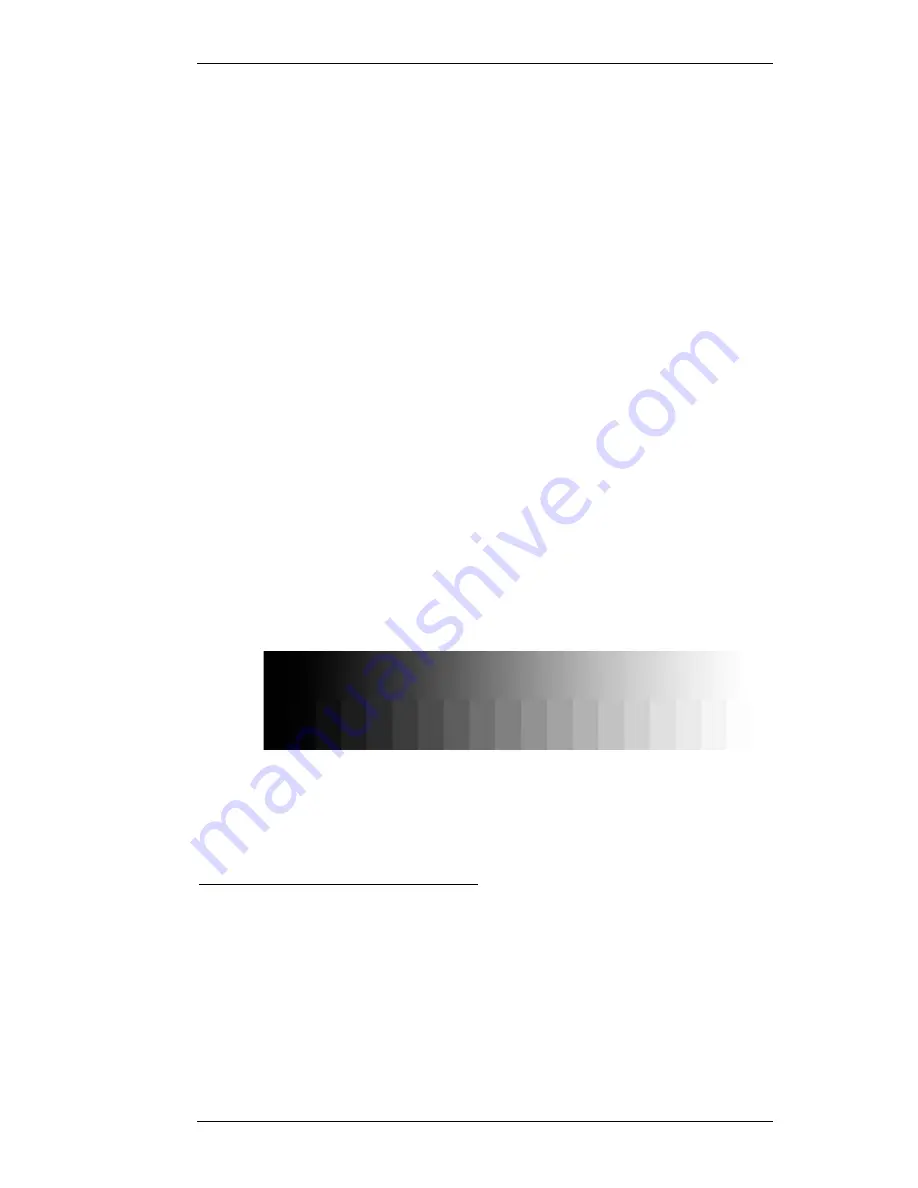
Here’s a tonal ramp rendered two ways. On the top, it’s rendered
as a continuous spectrum from black to white. On the bottom, I’ve
arbitrarily separated it into 19 different tones (slightly better than a
4-bit value can contain). The more tones we use to go from black
to white, the more subtle transitions like this look. This is one
21
You don’t set bit depth for JPEG and TIFF images, which are reduced by the camera
to 8-bits from 12-bit data collected by the sensor (yes, 12-bit; only NEF does 14-bit).
22
I know I’m going to get grief on this one, as most people can’t
see
any difference
between 12-bit and 14-bit data (indeed, you need a great deal of image training in
order to do so). Heck, most people can’t see the difference between 8-bit and 14-bit
data. The usual logic these people then try to use is this: because I can’t see it, it
doesn’t exist. Hmm. Can you see atoms? Maybe
you
don’t exist! Differences in bit
depth become more important with each additional post processing step that gets
applied to the data.
23
n
bits can enumerate 2^
n
values.
















































