7-3
PEAK
CURRENT
PEAK
CURRENT
PEAK
POTENTIAL
PEAK
POTENTIAL
BASELINE
BASELINE
PEAKAREA
PEAKAREA
Ap
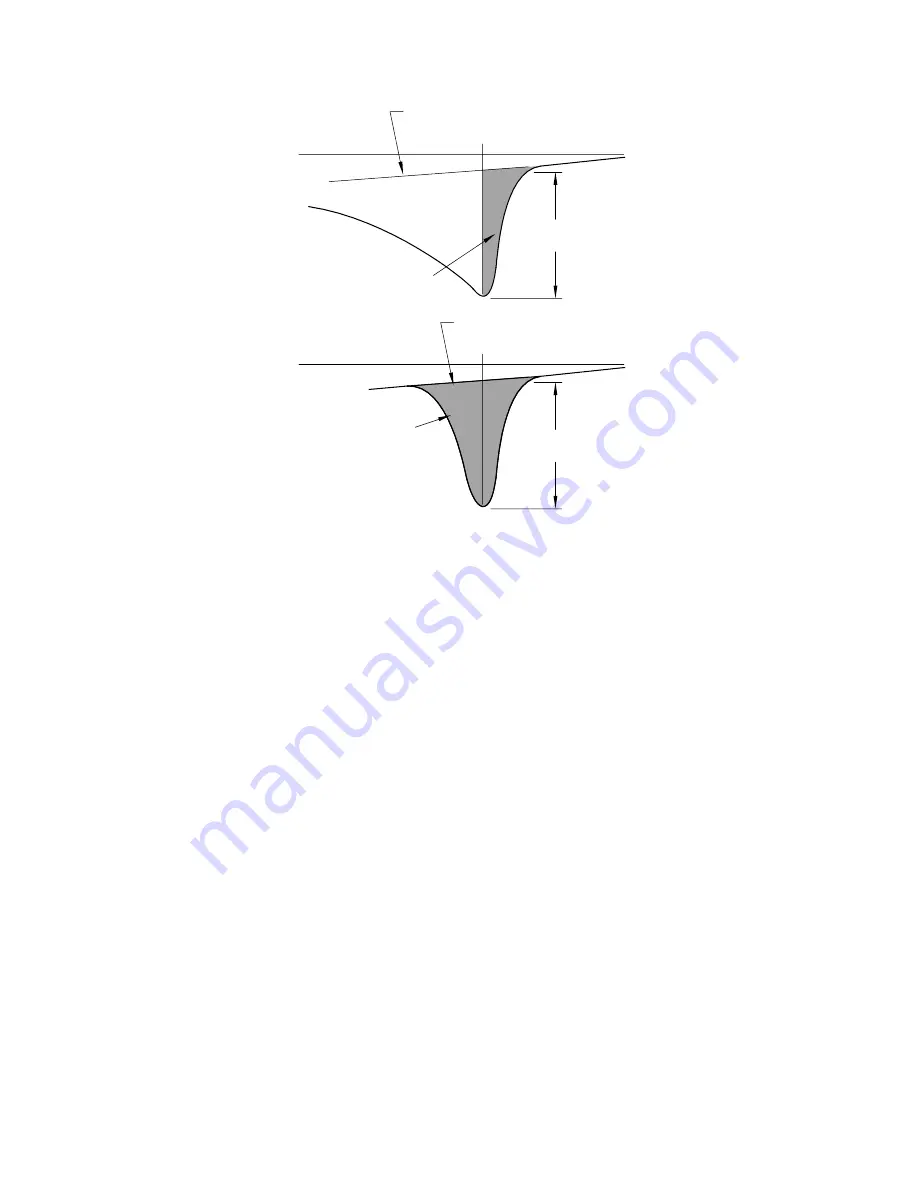
Figure 7-1. Peak area for symmetric and tailed curves.
Determination of Peak Potentials and Inflection Points
NOTE: If there are fewer than 30 points available in a data set of segment, peak
finding is not possible and a warning message is shown.
The peak potentials of tailed and symmetric current-potential curves and
inflection points of sigmoidal current-potential curves are determined by the
manipulation of the first or second derivatives respectively. First derivatives are
calculated as differentials across a potential window,
∆
E, of about 25 mV; that is,
∆
I is calculated as I(E-25 mV)-I(E). This order is used to produce a consistent
sign convention. The 25 mV interval is not rigid. The actual value is chosen such
that an odd number of data points intervene, so that the
∆
I value can be assigned
to the middle potential of the interval. The derivative plots are actually plots of
these
∆
I's vs. assigned median
∆
E values, not
∆
I/
∆
E vs.
∆
E, so the vertical axis
has dimensions of current on the same scale used for the
∆
I vs.
∆
E plot. The
derivative values for the first 12 mV and the last 12 mV in a scan are simply
equated to the values assigned to the first and last potentials at which
∆
I values
could be assigned. The second derivative works in the same way, but it operates
on the first derivative array.
Peak potentials are determined by examining the first derivative plots. The peak
potential value, E
p
, is determined by first looking for a sign reversal; that is, plus
to minus or minus to plus. The first
∆
I sign reversal becomes the E
p
window (note
















































