51
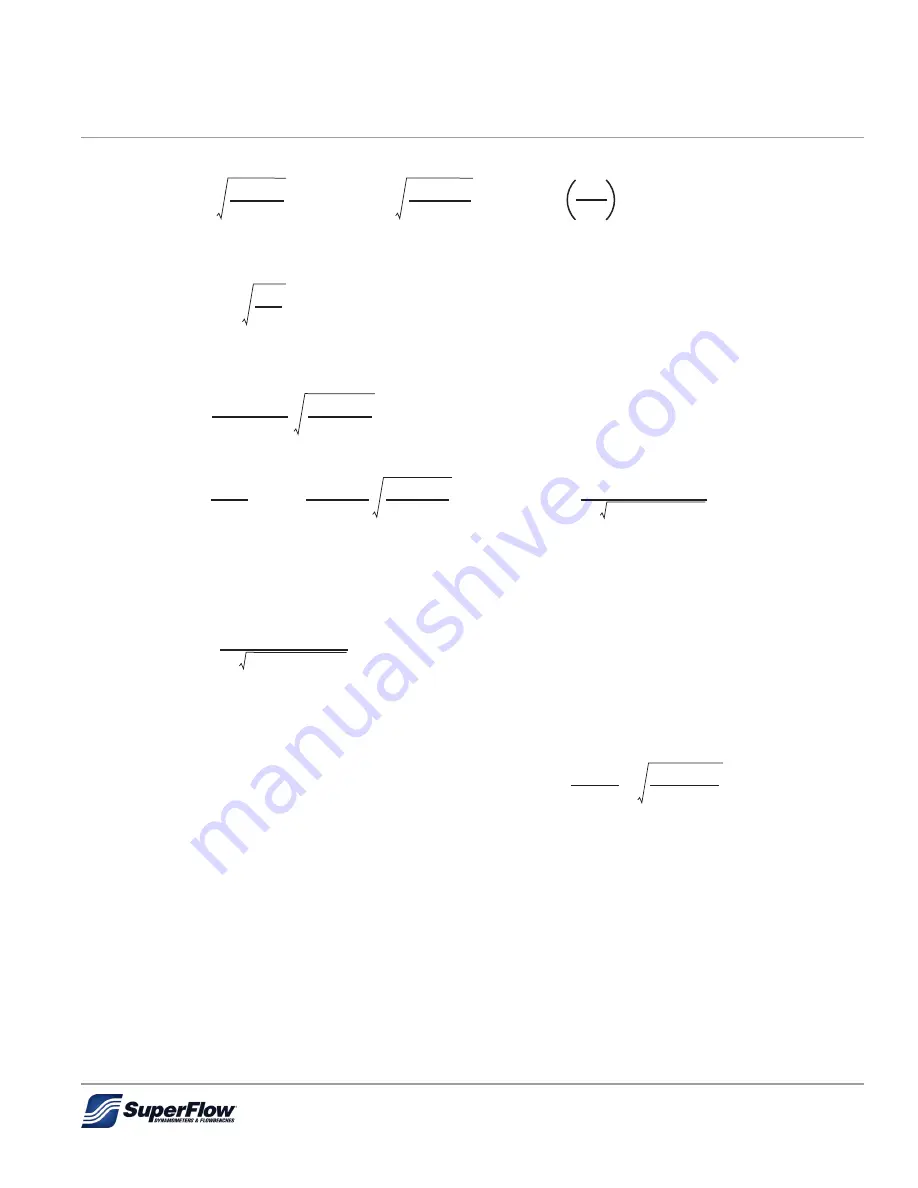
Equation 2:
Q K
f
P
flow
P
ambient
=
Q
1
K
f
P
fl
P
0
-
TP
1
=
P
fl
Q
1
K
f
=
X
(P
0
-
TP
1
)
The flowbench flow is calculated from the flow pressure (P
f1
) with this formula, where
FSF
is the full-scale
flow for the current flow range.
FBF FSF P
fl
P
fs
=
Where
P
fs
is the full-scale flow pressure—the pressure at which full-scale flow is reached.
When substituting for
P
f1
from
Equation 2
, we get:
FBF
1
P
0
-
TP
1
P
fs
FSF
x
Q
1
K
f
=
When substituting for
Q
1
from
Equation 1
:
FBF
1
Q
0
P
0
-
TP
1
P
fs
FSF
K
f
=
X
P
0
P
0
-
TP
1
FBF
1
FSF x Q
0
P
0
K
f
P
fs
(P
0
- TP
1
)
=
This last equation expresses flowbench flow as a function of constants: the intake flow, the atmospheric
pressure, and test pressure.
We can perform the exact same steps for the diagram on the right-hand side in figure 6.12, where the test
pressure is
TP
2
instead of
TP
1
. When we do, we get a similar equation for flowbench flow:
FBF
2
FSF x Q
0
P
0
K
f
P
fs
(P
0
- TP
2
)
=
In reality, the two flowbench readings (FBF) should be the same, but they are not. FBF
1
is correct
because the bench was calibrated at TP
1
. We can define a correction factor that will yield FBF
1
even when the test pressure is TP
2
.
FBF
2
FBF
1
P
0
- TP
2
P
0
- TP
1
=
X
corr(TP
1
,TP
2
)
=
=
corr(TP
1
,TP
2
)
FBF
1
FBF
2
This correction factor has been verified experimentally.
7.0 Flowbench Theory
















































