88
Problems when evaluating limits
In evaluating limits to infinity using substitution, problems can be encountered
if values are used which are too large.
For example:
lim
x
x
x
e
e
→∞
+
2
6
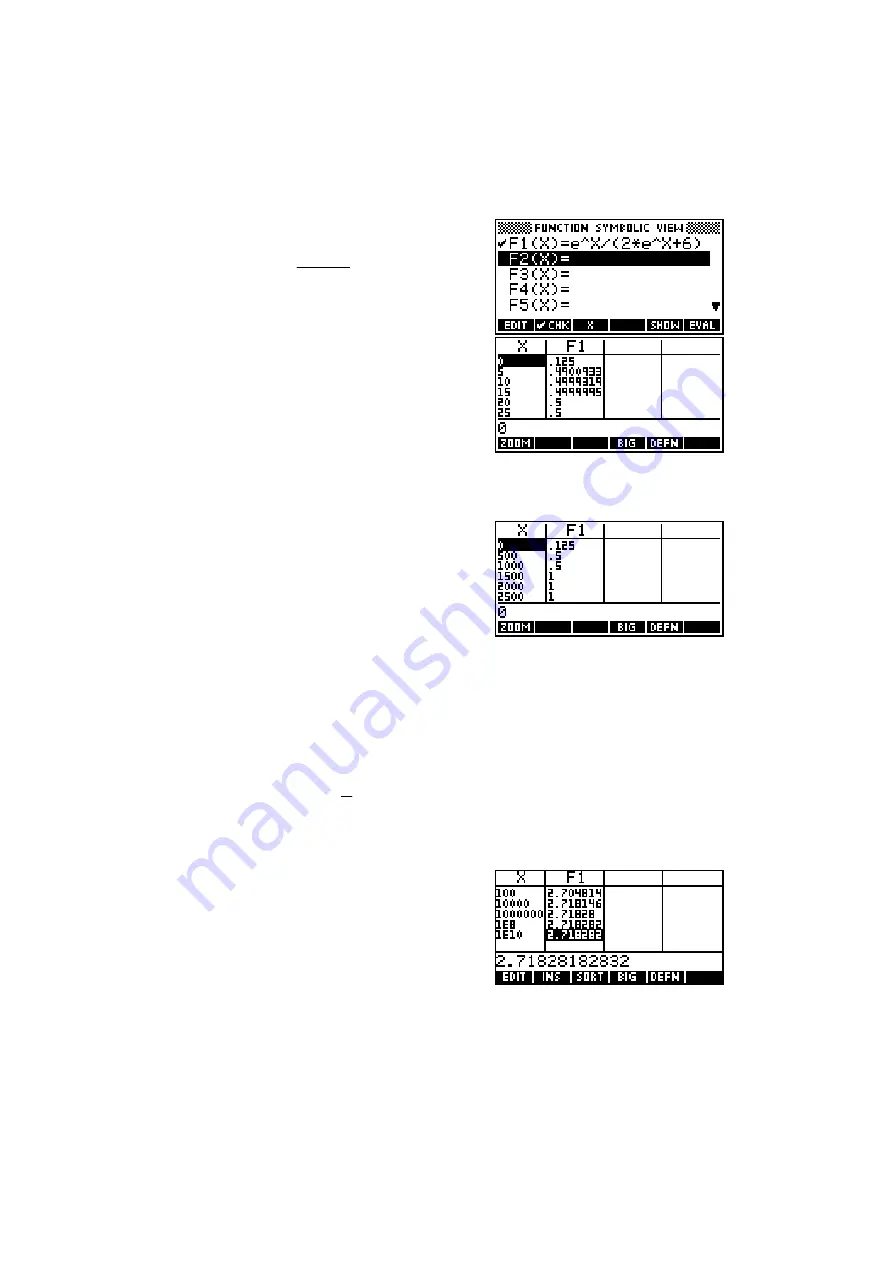
It is possible to gain a good idea of the value of
this limit by entering the function
F1(X)=e^X/(2*e^X+6) into the Function aplet,
changing to the NUM view and then trying
increasingly large values. As you can see
(right) the limit appears to be 0.5, which is correct.
However
, if you continue to use larger values
then the limit appears to change to 1 (see
right). The reason for this is that the value of
e
x
passes the limit of the capacity of the
calculator (10
500
), and so the top and bottom of
the fraction become equal (both at a value of
10
500
) instead of the true situation of the bottom being roughly twice the top.
This is most likely to happen with limits involving power functions as they will
overflow for smaller values of x.
A related effect happens when investigating the behavior of the commonly
used calculus limit of
1
lim 1
n
n
n
→∞
+
.
Suppose that you wish to use the Function
aplet to evaluate what happens to
F1(X)=(1+1/X)^X by changing to the NUM view
and choosing the ‘Build Your Own’ facility (see
right). The convergence towards e can also be
seen graphically in the PLOT view but is very
slow to reach high accuracy.






























