©2005 Nordic ID Oy
Nordic ID PL3000 user manual, v. 2.4, March 2006
78
������������
������������
������������
��������
��������
��
������
��������
��
������
��������
��
������
���������
��������
���������
��������
���������
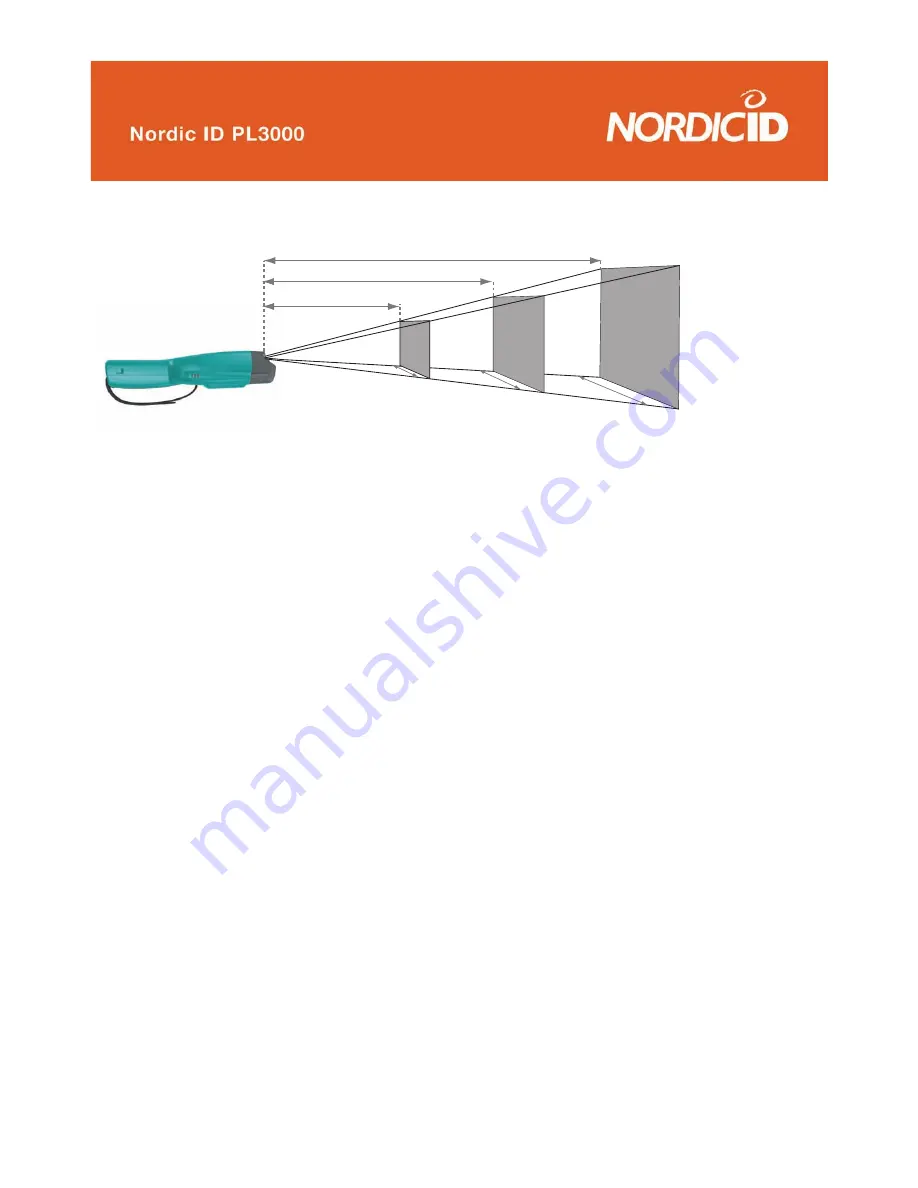
Figure: The PL3000 Field of View in given ranges
ID Symbology:
A symbology (or code) is a protocol for arranging the bars and spaces or blacks and whites that make up a
particular kind of ID symbology. Often 1D symbologies are referred as bar codes. There is no one standard
bar code; instead, it’s estimated that there are currently over 400 barcode symbologies that serve different
uses, industries, or geographic needs.
Symbologies can be designed to encode numbers, letters, special character control characters, or
a combination of any of the three. There are generally two kinds of barcode symbologies; discrete and
continuous. A discrete symbology is one where every character in the bar code can be interpreted
individually without referencing the rest of the bar code. A continuous symbology is one where individual
characters in the bar code cannot be interpreted individually. Most barcode readers have a feature called
“auto-discrimination”, which allows them to be configured to automatically recognize and read different
barcode symbologies, much the same way a human reader can interpret and read different font types.
However the 1D (one-dimensional) barcodes can encode only very limited number of symbols, a 2D code
is actually a portable data file that travels with the product it is affixed to, and provides large amounts of
machine-readable information. In this way all 2D symbologies are fundamentally different from the traditional
1D bar codes.
2D symbologies can encode any data such as ASCII, alphanumeric, or binary data. They also utilize
sophisticated error/erasure correction algorithms to compensate for lost or missing data, extraneous marks,
or label damage. Currently there are approximately two dozen 2D symbologies in the market place. They
can be divided into two main categories: Stacked Linear Codes and Matrix Codes.

































