Appendix
E:
Mechanical dynamic loading
DLoG MPC 6
Manual
161
20.4.3.
Approximate solution for determining insulating effects
A transmission function can be used to reach an exact calculation. However, we will not
detail this function here. The following equation is based on this transmission function
(very small damping factors of approx. 0.05) and is good for making estimates:
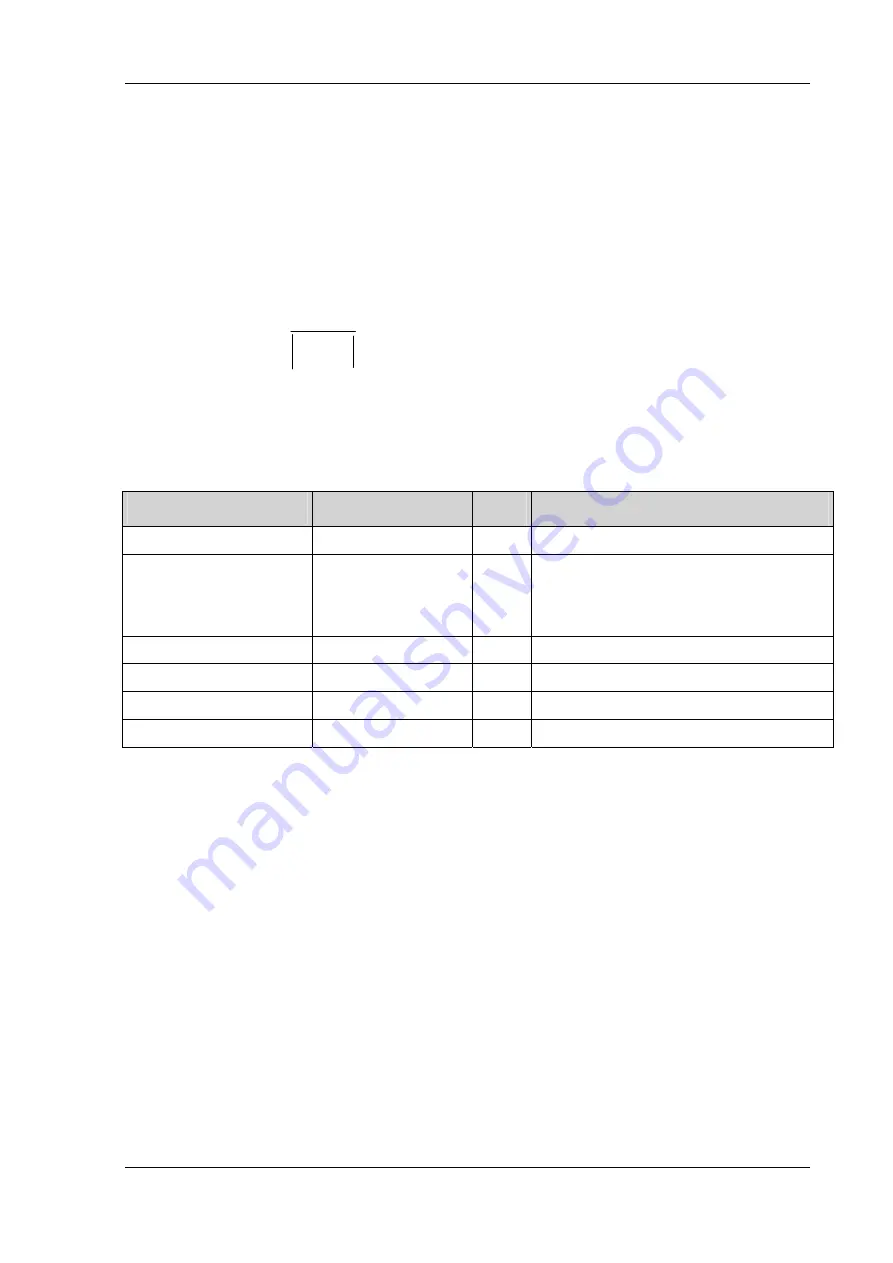
Where
λ
= excitation frequency / natural frequency
for
λ
not equal to 1
Excitation frequency Natural frequency
λ
Degree of insulation
10 Hz
20 Hz
0.5
-33 %
Warning! Amplification!
20 Hz
20 Hz
1
Warning! Resonance, approx. –
500 % and greater! High
amplification!
Approx. 28 Hz
20 Hz
√
2
0, no insulation
40 Hz
20 Hz
2
66 %
60 Hz
20 Hz
3
88 %
80 Hz
20 Hz
4
93 %
Based on this table, we can clearly expect very good insulation for excitation frequencies
that are twice as high as the system’s natural frequency.
Consequently, the amplitude of the reaction accelerations of the DLoG MPC 6 still only
reaches 66 % of the amplitude of the excitation accelerations, which actually have an
effect twice that of the natural frequency.
The table also demonstrates the costs of achieving this, namely that all excitation
frequencies below the natural frequency are amplified – to a maximum when resonance
occurs.
ung
eschleunig
Anregungsb
g
chleunigun
aktionsbes
grad
Isolations
/
Re
%
100
1
1
1
2
=
×
⎟
⎟
⎟
⎠
⎞
⎜
⎜
⎜
⎝
⎛
−
−
≅
λ






























