UMAX0608XX‐1000. Tri‐Axial J1939 CAN Inclinometer. Version 9B
Page: 10-66
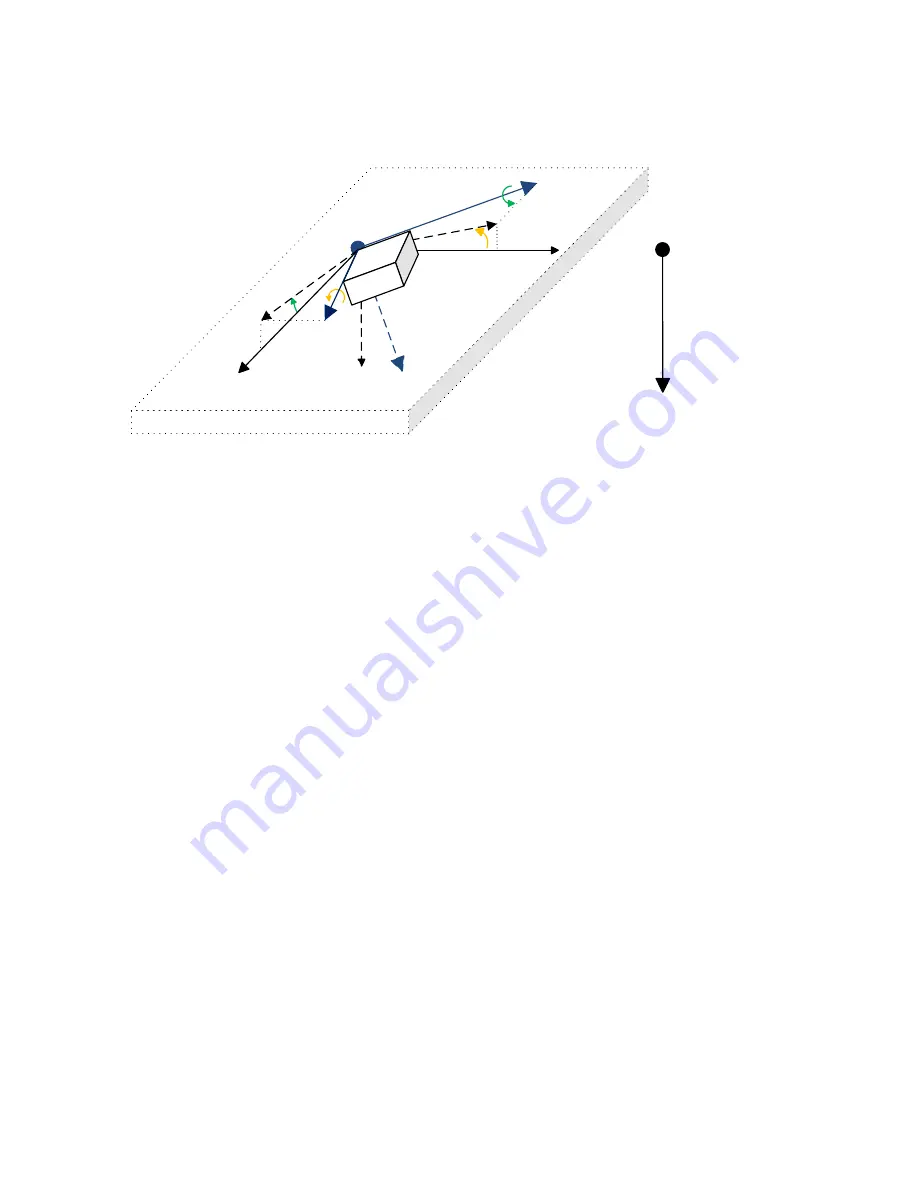
2.1.3.2.1 Unit Rotation Angles
The unit rotation angles define rotations about the axes in the unit frame (X,Y,Z) the following
way, see Figure 4.
X‐ Axis
Y ‐ Axis
Z‐ axis
Plane (X
E
,Y
E
) is parallel to the Earth surface
θ
u
‐ Pitch
φ
u
‐ Roll
X
E(XZ)
*
Y
E(YZ)
*
θ
u
> 0
φ
u
< 0
Gravity vector
𝑔
is coincident
with the Z
E
axis
⃗
Y
E
X
E
Z
E
Figure 4. Simple Rotation Angles
The rotation about the Y-axis defines the pitch angle
𝜃
and the rotation about the X axis – the
roll angle
𝜙
. The pitch angle
𝜃
is an angle between the horizontal projection X
E(XZ)
*
of the
unit X axis onto the (X
E
,Z
E
) plane and the X
E
axis. Similarly, the roll angle
𝜙
is an angle
between the horizontal projection Y
E(YZ)
*
of the unit Y axis onto the (Y
E
,Z
E
) plane and the Y
E
axis.
The (X
E
,Z
E
) and (Y
E
,Z
E
) planes are perpendicular to the Earth surface (X
E
,Y
E
) in the Earth
frame (X
E
,Y
E
,Z
E
). The angle between X
E(XZ)
*
and Y
E(YZ)
*
is always 90°.
The rotation about the Z-axis (yaw angle) is not shown in Figure 4. It cannot be calculated
based on the gravity acceleration
𝑔⃗
.
The sign of the pitch and roll angles is defined by the right-hand rule and presented by the
arrows about the Y and X axes. Since the pitch angle
𝜃
direction in Figure 4 is the same as
the positive direction defined by the yellow arrow about the Y axis, the angle is positive. The
same way, the roll angle
𝜙
direction is the opposite to the positive direction defined by the
green arrow about the X axis. Therefore, the roll angle
𝜙
in Figure 4 is negative.
The unit rotation angles are calculated using the following formulas:
𝜃
𝑎𝑡𝑎𝑛2 𝑔 , 𝑔
,
𝜃 ∈
180
°
; 180
°
,
(7)
𝜙
𝑎𝑡𝑎𝑛2 𝑔 , 𝑔
,
𝜙 ∈
180
°
; 180
°
,
where: 𝑔
𝑎 , 𝑔
𝑎 , 𝑔
𝑎 .
The roll-over condition is observed when:
|𝜃 |
90
°
or
|𝜙 |
90
°
.
When the unit is parallel to the Earth surface, the unit rotation angles are zero:
𝜃
𝜙
0°
.

























