LumaSense technologies MIKRON MCL640, Руководство пользователя

Страница: 23 / 39

MCL640 Manual Principles of Thermal Imaging
∂
23
If all energy falling on an object were absorbed (no transmission or reflection), the absorptivity
would equal to 1. At a steady temperature, all the energy absorbed could be re-radiated
(emitted) so that the emissivity of such a body would equal 1. Therefore in a blackbody,
absorptivity = emissivity = 1
Practical real life objects do not behave exactly as this ideal, but as described with transmissivity
and reflectivity,
absorp transmis reflectivity = 1
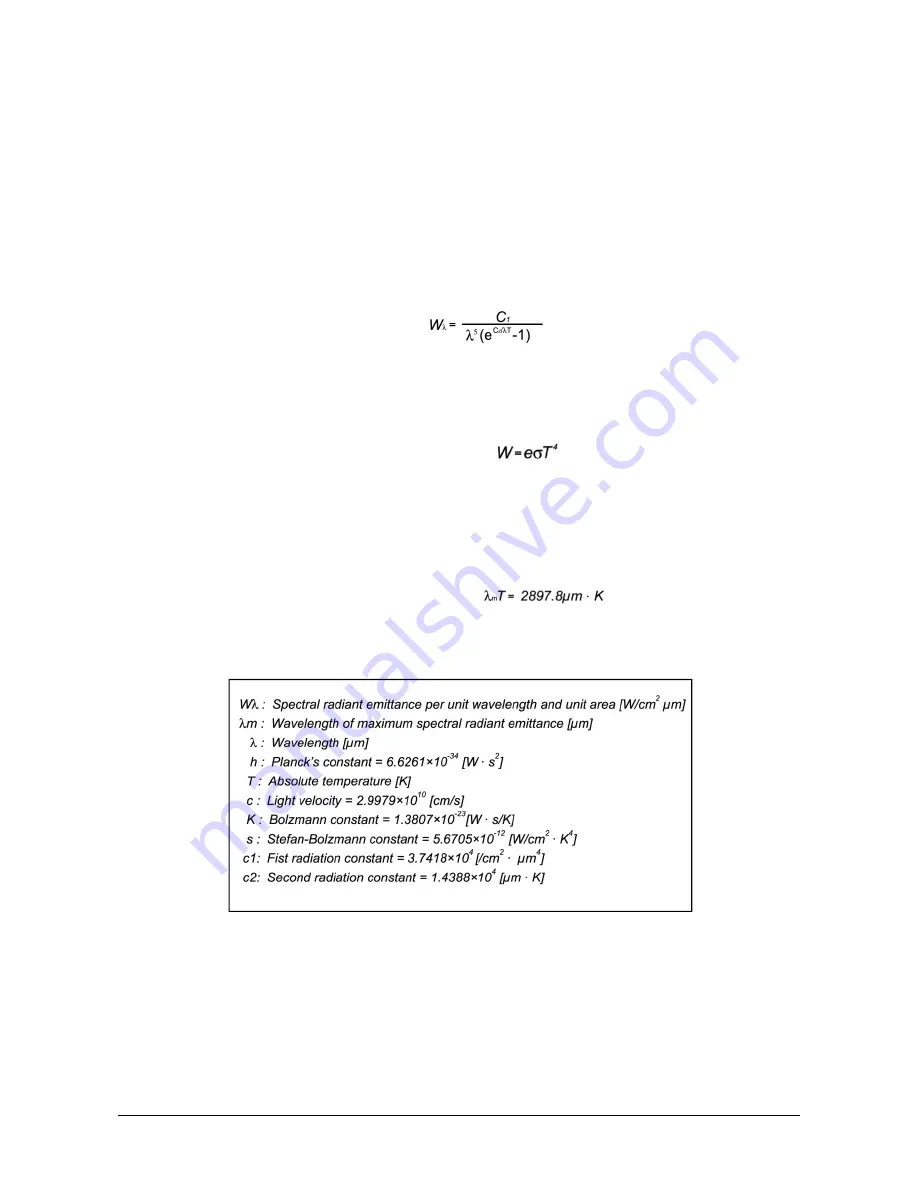
Planck’s Law
Energy radiated from the blackbody is described as follows
[“Planck’s Law”.]
1)
Stefan Bolzmann’s
equation
In order to obtain total radiant emittance of the blackbody,
integrate the equation (1) through all wavelengths (0 to infinity).
The result is as follows and is called “Stefan-Bolzmann equation.”
2)
Wien’s displacement
law
The temperature of blackbody can be obtained directly from the
radiant energy of the blackbody by this equation. In order to find
out the wavelength on the maximum spectral radiant emittance,
differentiate Planck’s law and take the value to 0.
3)
The equation is called “Wien’s displacement law”.
Where in (1) to (3),
In radiation of a normal object, as the emissivity is (<1) times of the blackbody, multiply above
equation by the emissivity. The following figures show the spectral radiant emittance of a
blackbody.
(a) is shown by logarithmic scale and (b) is shown by linear scale.