Lambda SR1, Руководство по эксплуатации, Страница: 146 / 317

SR1 Operation Manual 146
© 2014 Stanford Research Systems
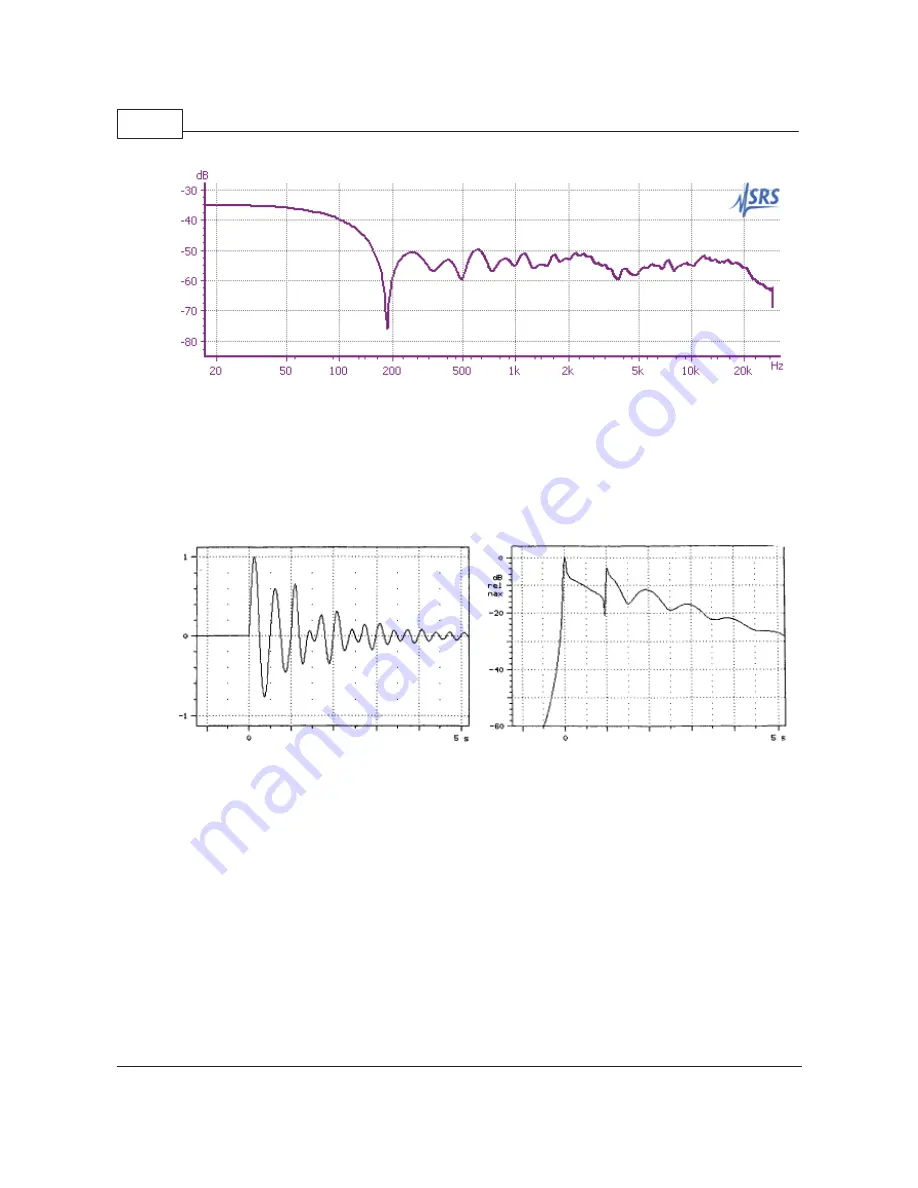
Anechoic Frequency Response
Energy Time Curve
The energy time curve (ETC), is an attempt to find an "envelope" function for the impulse response that
attempts to illuminate features of the impulse response that may be obscured by interference effects.
For example, the synthesized impulse response shown on the left below consists of a high-amplitude
sinewave with a fast decay time constant mixed with a delayed lower amplitude sign with a slower decay
time-constant.
Left: Im pulse response consisting of a high am plitude sinusoid w ith a fast decay tim e constant and a delayed
low er am plitude sine w ith a slow er decay tim e constant. Right: ETC of the im pulse response. (From Andrew
Duncan, "The Analytic Im pulse", Presented at the 81st AES Convention, Novem ber 1986.)
While this may not be immediately obvious from the impulse response graph it is more evident in the
computed ETC.
The ETC is calculated by finding an appropriate "imaginary part" for the impulse response in the same
way that sine is the appropriate imaginary part for a uniform phasor whose real part is cosine.
Technically the imaginary part is found by computing the Hilbert transform of the impulse response. The
complex function whose real part is the impulse response and whose imaginary part is its Hilbert
transform is known as the analytic impulse response of the system. The ETC is the magnitude of the
analytic impulse.
SR1 calculates the ETC from the anechoic frequency response. A frequency-domain window is applied
to the anechoic frequency response to reduce alias effects. The result is transformed back to the time-
domain to obtain the real part of the analytic impulse response. A phase shifted (Hilbert transformed
version) is transformed to obtain the imaginary part of the analytic impulse. The magnitude of the
resulting function is the ETC.