51
F.2
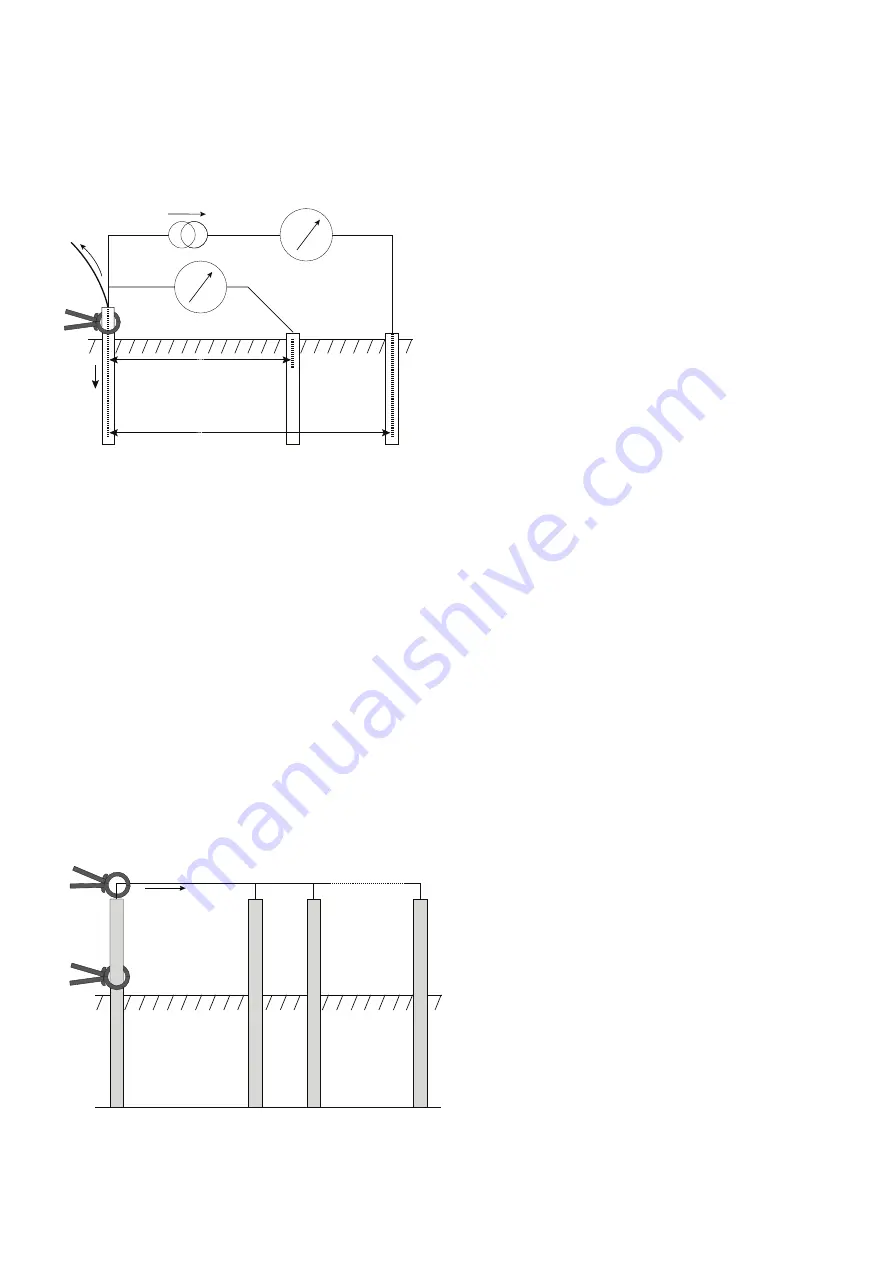
Principle of operation (three-terminal resistance measurement using ART)
The classic three-terminal test method has a disadvantage, namely that the electrode under test must be disconnected from the system
it is supposed to protect in the event of a power system fault. The reason for this is that the injected test current will take all possible
routes to ground and not all of it will necessarily flow through the electrode under test. In this case, the instrument will make a reading
of the entire earthing network, not just the individual electrode.
By using a current transducer (the Megger ICLAMP) to measure the current flowing through the electrode under test as a fraction of the
total test current injected, the instrument can determine the individual resistance. This arrangement is shown below:
A
Earth electrode
under test
Connection
to rest of system
B
C (H)
P (S)
X (E)
I2
I1
Potential stake
ICLAMP
Current stake
V
I
schematic for three-terminal resistance measurement using
In this configuration, the injected test current I splits along two paths into I1 (flowing into the connected earthing system) and I2
(flowing into the electrode under test, i.e. I=I1+I2. The resistance of the electrode under test is calculated as R=V/I2 or R=V/(I-I1). The
current transducer (ICLAMP) measures I2 and feeds this value back to the instrument.
F.3
Principle of operation (two-clamp stake-less resistance measurement)
MFT1835 only
In this example, the electrode under test is connected to a network of other electrodes. It is either
impractical or unsafe to disconnect an individual electrode for testing. Also, there might be insufficient space to perform a classic three-
terminal resistance measurement. The stake-less test method using both VCLAMP and ICLAMP can be used to obtain a measurement
for the electrode under test.
A defined test voltage is injected into the system using the VCLAMP, inducing a current, I, to flow and be measured by the ICLAMP. The
model shown in Figure 7 can be simplified to the resistance of the electrode under test, Rx and the resistance of the other electrodes in
parallel, i.e. R1 || R2 || … || Rn.
Therefore, the current induced by the test voltage is I=V/[Rx+(R1 || R2 || … || Rn)]. It follows that as the resistance of the other electrodes
in parallel approaches zero, then the resistance measured, approaches the value of the electrode under test.
Earth electrode
under test
Connection
to rest of system
X (E)
I
I
V
R2
R1
Rx
ICLAMP
VCLAMP
Rn
schematic for two-clamp stake-less resistance measurement





































